Understanding Portfolio Variance: The Key to Informed Investment Decisions
In the world of investing, managing risk is a delicate balancing act. One crucial aspect of this process is understanding portfolio variance, a metric that measures the dispersion of returns around the mean. Calculating the variance of a portfolio is essential for investors and portfolio managers, as it provides valuable insights into the overall risk profile of their investments. By grasping the concept of portfolio variance, investors can make informed decisions about asset allocation, risk management, and performance evaluation. In essence, knowing how to calculate the variance of a portfolio is a vital step in creating a well-diversified investment strategy that balances risk and return. In this article, we will explore the importance of portfolio variance, its calculation, and its applications in real-world investment scenarios, providing readers with a comprehensive guide on how to master portfolio risk management.
What is Portfolio Variance and Why Does it Matter?
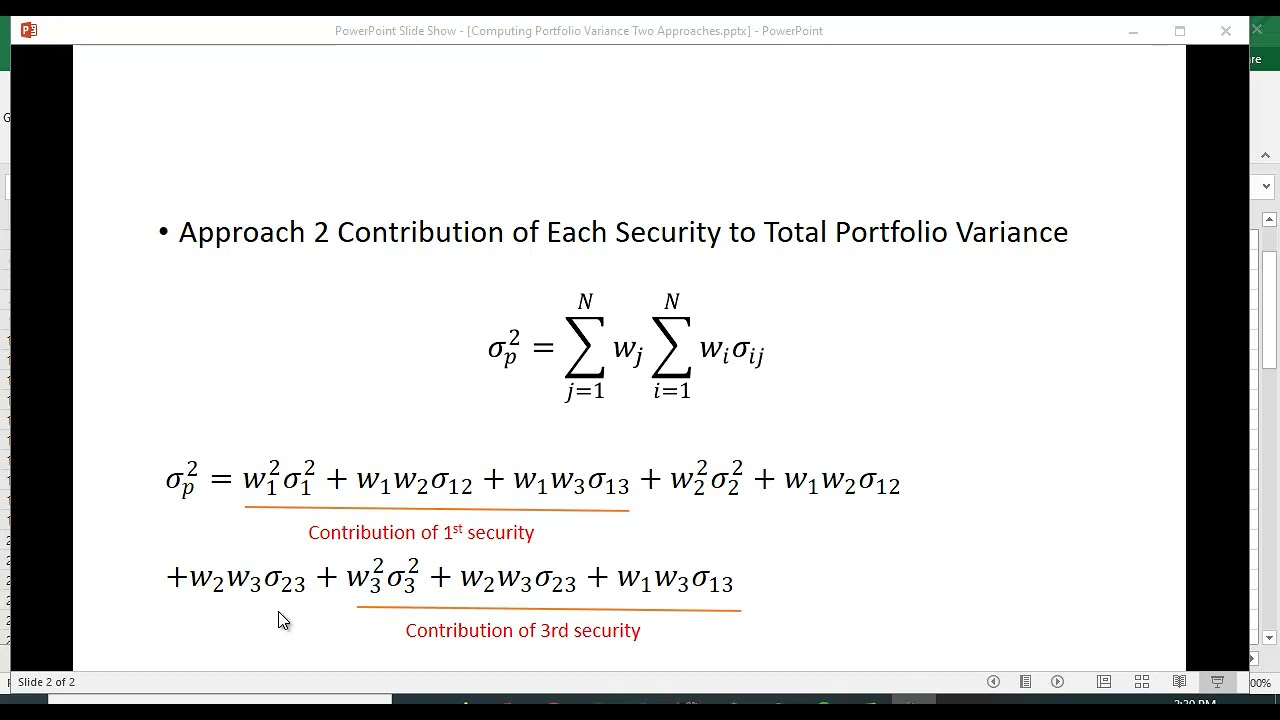
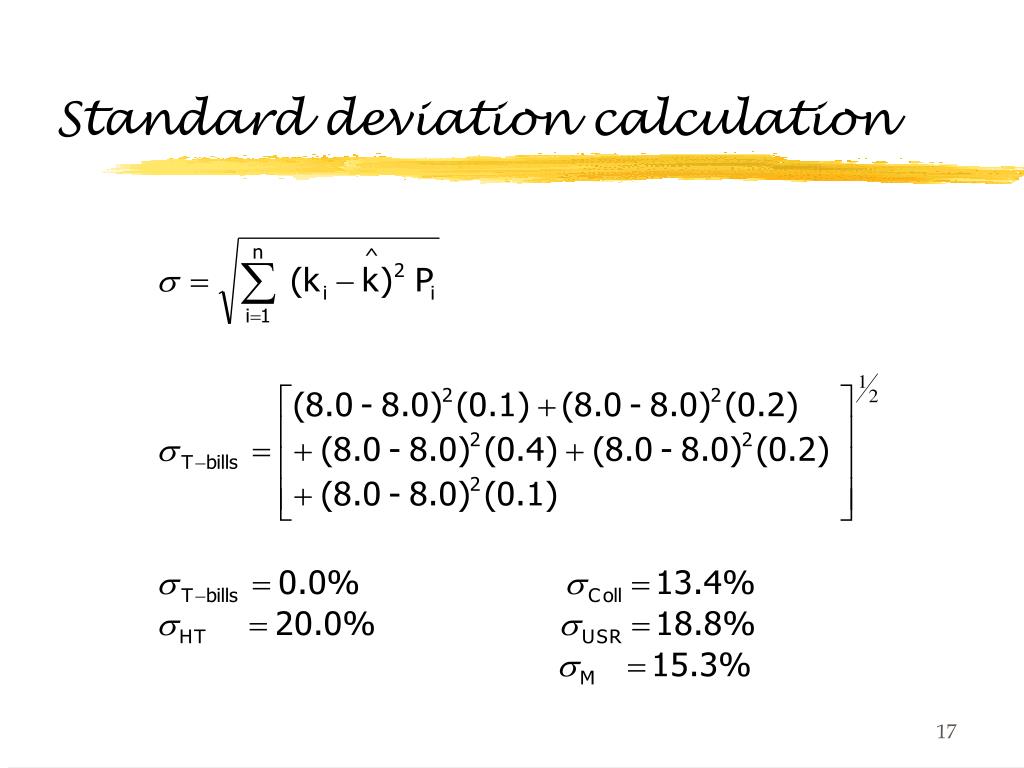
Portfolio variance is a statistical measure that quantifies the dispersion of returns around the mean, providing a snapshot of the overall risk profile of an investment portfolio. It is calculated as the average of the squared differences between each return and the mean return. The formula for portfolio variance is: σ^2 = Σ(xi – μ)^2 / (n – 1), where σ^2 is the portfolio variance, xi is each individual return
How to Calculate Portfolio Variance: A Simplified Approach
Calculating portfolio variance is a crucial step in understanding the risk profile of an investment portfolio. Fortunately, the process is relatively straightforward. To calculate portfolio variance, you’ll need the following data: the returns of each asset in the portfolio, the weights of each asset, and the number of observations. The formula for portfolio variance is: σ^2 = Σ(xi – μ)^2 / (n – 1), where σ^2 is the portfolio variance, xi is each individual return, μ is the mean return, and n is the number of observations. To illustrate the calculation process, let’s consider a simple example. Suppose we have a portfolio consisting of two assets, A and B, with returns of 10% and 8%, respectively, and weights of 60% and 40%, respectively. Using the formula, we can calculate the portfolio variance as follows: σ^2 = [(10% – 9%)^2 + (8% – 9%)^2] / (2 – 1) = 0.002. This result indicates that the portfolio has a relatively low variance, suggesting a lower level of risk. By understanding how to calculate the variance of a portfolio, investors and portfolio managers can gain valuable insights into the risk profile of their investments and make informed decisions to optimize portfolio performance.
The Role of Asset Correlations in Portfolio Variance
Asset correlations play a crucial role in portfolio variance calculation, as they can significantly impact the overall risk profile of an investment portfolio. Correlations measure the degree to which the returns of two or more assets move in tandem. When assets are highly correlated, their returns tend to move together, increasing the overall portfolio risk. Conversely, when assets are negatively correlated, their returns tend to offset each other, reducing portfolio risk. To incorporate correlations into the variance calculation, investors and portfolio managers can use the following formula: σ^2 = ΣΣ(xi – μ)(xj – μ) / (n – 1), where σ^2 is the portfolio variance, xi and xj are the returns of assets i and j, μ is the mean return, and n is the number of observations. By accounting for correlations, investors can gain a more accurate understanding of their portfolio’s risk profile and make informed decisions to optimize performance. For example, a portfolio consisting of two highly correlated assets, such as two stocks in the same industry, may have a higher variance than a portfolio consisting of two negatively correlated assets, such as a stock and a bond. By understanding how to calculate the variance of a portfolio and incorporating correlations into the calculation, investors can better navigate the complexities of portfolio risk management and achieve their investment goals.
Common Mistakes to Avoid When Calculating Portfolio Variance
When calculating portfolio variance, investors and portfolio managers must be aware of common mistakes that can lead to inaccurate results and misguided investment decisions. One common error is failing to account for correlations between assets, which can significantly impact portfolio risk and returns. Another mistake is using incorrect or outdated data, such as historical returns that do not reflect current market conditions. Additionally, investors may incorrectly assume that a diversified portfolio automatically reduces risk, without considering the correlations between assets. Furthermore, using overly complex formulas or models can lead to errors and misunderstandings. To avoid these mistakes, it is essential to understand the underlying concepts of portfolio variance and to carefully follow the calculation process. By doing so, investors can ensure that their calculations are accurate and reliable, and that their investment decisions are informed by a thorough understanding of portfolio risk. For example, when calculating the variance of a portfolio, investors should ensure that they are using the correct formula, such as σ^2 = Σ(xi – μ)^2 / (n – 1), and that they are accounting for correlations between assets. By avoiding common mistakes and following best practices, investors can unlock the full potential of portfolio variance calculation and make informed investment decisions.
Interpreting Portfolio Variance Results: What Do the Numbers Mean?
Once the portfolio variance has been calculated, investors and portfolio managers must be able to interpret the results to inform their investment decisions. The portfolio variance represents the expected volatility of the portfolio, with higher values indicating greater uncertainty and potential for losses. A low portfolio variance, on the other hand, suggests a more stable portfolio with lower potential for losses. To interpret the results, investors should consider the following factors: the absolute value of the variance, the relative value compared to benchmarks or other portfolios, and the implications for investment returns. For example, a portfolio with a high variance may require more frequent rebalancing to maintain an optimal asset allocation. Conversely, a portfolio with a low variance may be more suitable for investors with a low risk tolerance. By understanding how to interpret the results of portfolio variance calculations, investors can make informed decisions to optimize their portfolio performance and achieve their investment goals. Additionally, investors can use the output to identify areas for improvement, such as adjusting the asset allocation or diversification strategy. By learning how to calculate the variance of a portfolio and interpret the results, investors can gain a deeper understanding of their portfolio’s risk profile and make data-driven decisions to drive success.
Real-World Applications of Portfolio Variance Calculation
Portfolio variance calculation is a crucial tool in real-world investment scenarios, with applications in asset allocation, risk management, and performance evaluation. For instance, in asset allocation, portfolio variance calculation helps investors determine the optimal mix of assets to achieve their investment goals while managing risk. By calculating the variance of a portfolio, investors can identify the most volatile assets and adjust their allocation accordingly. In risk management, portfolio variance calculation enables investors to quantify and manage risk, ensuring that their portfolio is aligned with their risk tolerance. Additionally, portfolio variance calculation is used in performance evaluation to assess the risk-adjusted returns of a portfolio. This helps investors to evaluate the performance of their portfolio and make informed decisions to optimize its performance. For example, a portfolio manager may use portfolio variance calculation to evaluate the risk-adjusted returns of different asset classes and adjust the portfolio’s allocation to maximize returns while minimizing risk. By understanding how to calculate the variance of a portfolio and its real-world applications, investors can make informed decisions to drive success in their investment endeavors. Furthermore, portfolio variance calculation can be used in other areas such as portfolio optimization, risk parity, and factor-based investing, making it a versatile tool in the investment management process.
Conclusion: Unlocking the Power of Portfolio Variance Calculation
In conclusion, calculating portfolio variance is a crucial step in mastering portfolio risk management. By understanding how to calculate the variance of a portfolio, investors and portfolio managers can gain valuable insights into their portfolio’s risk profile and make informed decisions to optimize its performance. The benefits of portfolio variance calculation are numerous, including improved risk management, enhanced investment returns, and more effective asset allocation. By applying the knowledge gained from this article, investors can unlock the power of portfolio variance calculation and take their investment decisions to the next level. Whether you are a seasoned investor or just starting out, understanding how to calculate the variance of a portfolio is an essential skill that can help you achieve your investment goals. So, take the first step today and start calculating your portfolio variance to make more informed investment decisions and drive success in your investment endeavors.