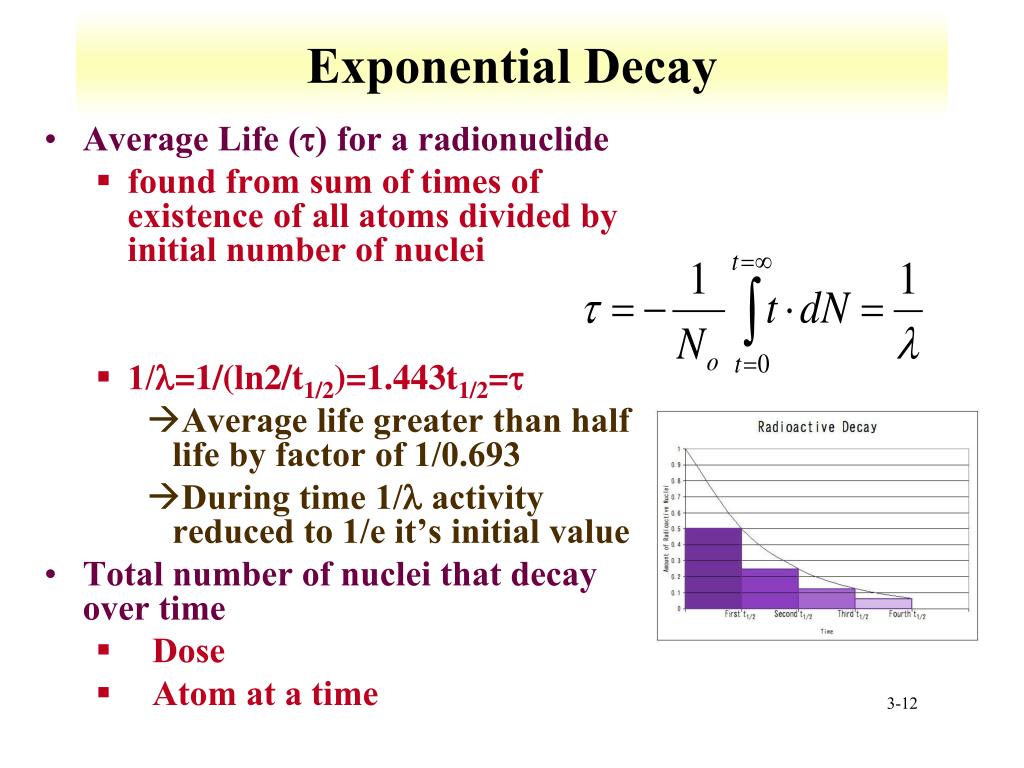
Understanding the Concept of Decay Factor
The concept of decay factor is a fundamental aspect of exponential decay, a ubiquitous phenomenon in nature and human-made systems. But what is the decay factor, and why is it essential in various fields such as physics, engineering, and finance? The decay factor, denoted by the symbol λ (lambda), is a measure of the rate at which a quantity decreases exponentially over time. It’s a crucial parameter in modeling and analyzing exponential decay, which is used to describe a wide range of phenomena, from the decay of radioactive materials to the depreciation of assets. In essence, the decay factor is a key component in understanding how exponential decay works, and its significance extends to various fields where exponential decay is a critical concept.
How to Calculate Decay Factor in Real-World Scenarios
Calculating the decay factor is a crucial step in modeling exponential decay, and it’s essential to understand the process in different contexts. In this section, we’ll provide a step-by-step guide on how to calculate the decay factor in various scenarios, including radioactive decay, population growth, and financial modeling.
In radioactive decay, the decay factor is used to model the rate of decay of radioactive materials. The decay factor (λ) is calculated using the formula λ = ln(2) / t1/2, where t1/2 is the half-life of the material. For example, if the half-life of a radioactive material is 10 years, the decay factor would be λ = ln(2) / 10 = 0.0693 per year.
In population growth, the decay factor is used to model the rate of decline of a population. The decay factor is calculated using the formula λ = r / N, where r is the rate of decline and N is the initial population size. For instance, if a population is declining at a rate of 5% per year, and the initial population size is 1000, the decay factor would be λ = 0.05 / 1000 = 0.0005 per year.
In financial modeling, the decay factor is used to model the depreciation of assets. The decay factor is calculated using the formula λ = 1 – (1 – d)^n, where d is the depreciation rate and n is the number of periods. For example, if an asset depreciates at a rate of 20% per year, and there are 5 periods, the decay factor would be λ = 1 – (1 – 0.2)^5 = 0.3277 per year.
These examples illustrate the importance of understanding how to calculate the decay factor in different contexts. By applying these formulas, individuals can accurately model exponential decay and make informed decisions in their respective fields.
The Role of Half-Life in Decay Factor Calculations
Half-life is a fundamental concept in exponential decay, and it plays a crucial role in decay factor calculations. In essence, half-life is the time it takes for the quantity to reduce to half of its initial value. The decay factor (λ) is intimately connected with half-life, as it determines the rate at which the quantity decays.
The relationship between half-life and decay factor can be expressed mathematically as λ = ln(2) / t1/2, where t1/2 is the half-life. This formula highlights the inverse relationship between half-life and decay factor: as half-life increases, the decay factor decreases, and vice versa.
In real-world scenarios, understanding the role of half-life in decay factor calculations is essential. For instance, in nuclear physics, the half-life of a radioactive material determines its decay rate, which in turn affects the decay factor. By knowing the half-life, scientists can accurately calculate the decay factor and predict the material’s decay over time.
In biomedical engineering, the half-life of a drug or medication is critical in determining its efficacy and decay rate. By understanding the relationship between half-life and decay factor, researchers can design more effective treatments and optimize drug delivery systems.
In conclusion, the half-life of a quantity plays a vital role in decay factor calculations, and understanding this relationship is essential in various fields. By grasping the concept of half-life and its connection to decay factor, individuals can make more informed decisions and accurately model exponential decay in real-world scenarios.
Applications of Decay Factor in Science and Engineering
The decay factor has far-reaching applications in various fields of science and engineering, where it plays a crucial role in modeling and analyzing exponential decay. In this section, we’ll explore some of the most significant applications of decay factor in these fields.
In nuclear physics, the decay factor is used to model the decay of radioactive materials, such as uranium and thorium. By understanding the decay factor, scientists can predict the half-life of these materials and design safer storage facilities. The decay factor also helps in calculating the radiation exposure and risk assessment.
In biomedical engineering, the decay factor is used to model the decay of drugs and pharmaceuticals in the body. This helps researchers to design more effective drug delivery systems and optimize the dosage for patients. The decay factor also plays a critical role in understanding the pharmacokinetics and pharmacodynamics of drugs.
In materials science, the decay factor is used to model the degradation of materials over time. This helps engineers to design more durable materials and predict the lifespan of structures, such as bridges and buildings. The decay factor also helps in understanding the corrosion rates and fatigue life of materials.
The decay factor also has applications in other fields, such as finance, where it’s used to model the depreciation of assets and calculate the present value of future cash flows. In population dynamics, the decay factor is used to model the growth and decline of populations, helping demographers to predict population trends and plan for resource allocation.
In conclusion, the decay factor has a wide range of applications in science and engineering, where it plays a vital role in modeling and analyzing exponential decay. By understanding the decay factor, professionals in these fields can make more informed decisions and design more effective systems.
Common Misconceptions About Decay Factor
Despite its widespread application, the decay factor is often misunderstood, leading to incorrect calculations and misinterpretations. In this section, we’ll address some common misconceptions about decay factor, providing clear explanations to dispel these misconceptions.
Misconception 1: Decay factor is only applicable to radioactive decay.
This is a common misconception, as the decay factor has far-reaching applications in various fields, including finance, biology, and materials science. The decay factor is a fundamental concept in exponential decay, and its applications extend beyond radioactive decay.
Misconception 2: Decay factor is a constant value.
This is not entirely accurate, as the decay factor can vary depending on the context and the specific application. For instance, the decay factor for radioactive decay is different from the decay factor for population growth. It’s essential to understand the specific decay factor for each application to ensure accurate calculations.
Misconception 3: Decay factor is only used to model exponential decay.
While the decay factor is primarily used to model exponential decay, it can also be used to model other types of decay, such as linear or logarithmic decay. The decay factor is a versatile concept that can be applied to various types of decay, making it a powerful tool in modeling complex systems.
Misconception 4: What is the decay factor is a simple question with a straightforward answer.
This is not entirely accurate, as the decay factor is a complex concept that requires a deep understanding of exponential decay and its applications. While the decay factor can be calculated using a simple formula, its interpretation and application require a nuanced understanding of the underlying principles.
By addressing these common misconceptions, we can ensure a clearer understanding of the decay factor and its applications. By recognizing the complexity and versatility of the decay factor, we can unlock its full potential in modeling complex systems and making informed decisions.
Real-World Examples of Decay Factor in Action
In this section, we’ll explore concrete examples of decay factor in action, illustrating its application in various fields. These examples will help to solidify the concept of decay factor and demonstrate its significance in real-world scenarios.
Example 1: Radioactive Decay of Uranium-238
Uranium-238 is a radioactive isotope with a half-life of approximately 4.5 billion years. Using the decay factor formula, we can calculate the decay rate of uranium-238 over time. For instance, if we start with 100 grams of uranium-238, after 1 billion years, we would be left with approximately 75 grams. This example illustrates the decay factor’s role in modeling radioactive decay.
Example 2: Population Growth in a City
Let’s consider a city with an initial population of 100,000 people, growing at a rate of 2% per year. Using the decay factor formula, we can calculate the population growth over time. For instance, after 10 years, the population would increase to approximately 121,970 people. This example demonstrates the decay factor’s application in population dynamics.
Example 3: Depreciation of Assets in Finance
In finance, the decay factor is used to model the depreciation of assets over time. For instance, let’s consider a company that purchases a piece of equipment for $10,000, with a depreciation rate of 10% per year. Using the decay factor formula, we can calculate the asset’s value over time. After 5 years, the asset’s value would decrease to approximately $6,477. This example illustrates the decay factor’s role in financial modeling.
These examples demonstrate the decay factor’s versatility and importance in various fields. By understanding the decay factor, professionals can make informed decisions, predict outcomes, and identify patterns in complex systems.
The Importance of Decay Factor in Modeling Complex Systems
In complex systems, understanding the decay factor is crucial for making informed decisions, predicting outcomes, and identifying patterns. By grasping the concept of decay factor, professionals can better navigate the intricacies of exponential decay and its applications.
In fields such as nuclear physics, biomedical engineering, and materials science, the decay factor plays a vital role in modeling complex systems. For instance, in nuclear physics, the decay factor helps predict the half-life of radioactive materials, enabling scientists to design safer and more efficient reactors. In biomedical engineering, the decay factor is used to model the degradation of biomaterials, informing the development of more durable and effective medical devices.
In finance, the decay factor is essential for modeling asset depreciation, allowing businesses to make informed decisions about investments and resource allocation. By understanding the decay factor, financial analysts can better predict the value of assets over time, mitigating risks and optimizing returns.
The decay factor’s significance extends beyond these fields, as it provides a fundamental understanding of exponential decay and its applications. By recognizing the importance of decay factor, professionals can develop more accurate models, make more informed decisions, and drive innovation in their respective domains. As the complexity of systems continues to grow, the decay factor will remain a vital tool for unlocking insights and driving progress.
What is the decay factor, and how does it impact the modeling of complex systems? By grasping this concept, professionals can unlock the secrets of exponential decay and make more informed decisions in their respective fields.
Conclusion: Mastering the Decay Factor for Informed Decision-Making
In conclusion, understanding the decay factor is crucial for making informed decisions in various fields, including physics, engineering, and finance. By grasping the concept of decay factor, professionals can unlock the secrets of exponential decay, predict outcomes, and identify patterns in complex systems.
Throughout this article, we’ve explored the significance of decay factor, its calculation, and its applications in real-world scenarios. We’ve also addressed common misconceptions and provided concrete examples to illustrate the concept. By mastering the decay factor, professionals can drive innovation, mitigate risks, and optimize returns in their respective domains.
So, what is the decay factor, and how can it inform decision-making? By recognizing the importance of decay factor, professionals can develop more accurate models, make more informed decisions, and drive progress in their fields. As the complexity of systems continues to grow, the decay factor will remain a vital tool for unlocking insights and driving innovation.
In summary, understanding the decay factor is essential for anyone working with exponential decay. By applying the concepts outlined in this article, professionals can unlock the full potential of decay factor and make informed decisions that drive success.