Understanding Portfolio Standard Deviation: A Key Metric
In the world of finance, risk analysis is a crucial aspect of investment decision-making. One key metric that helps investors gauge the risk of their portfolio is standard deviation. Standard deviation measures the volatility or dispersion of a portfolio’s returns from its mean return. A higher standard deviation indicates a riskier portfolio, while a lower standard deviation suggests a more stable one. Calculating portfolio standard deviation in Excel is a valuable skill for investors, as it enables them to make informed decisions about their investments. By understanding the concept of portfolio standard deviation, investors can better navigate the complexities of the financial markets and optimize their investment strategies. In fact, learning how to calculate portfolio standard deviation in Excel can be a game-changer for investors, providing them with a deeper understanding of their portfolio’s risk profile and enabling them to make more informed investment decisions.
Why Excel is the Ideal Tool for Portfolio Analysis
When it comes to portfolio analysis, having the right tool can make all the difference. Excel is an ideal choice for portfolio analysis due to its flexibility, ease of use, and ability to handle large datasets. With Excel, investors can easily organize and analyze their portfolio data, making it easier to calculate key metrics such as portfolio standard deviation. Excel’s built-in functions and formulas, such as the STDEV.S function, make it easy to calculate portfolio standard deviation, and its data visualization tools enable investors to create informative charts and graphs to help them understand their portfolio’s risk profile. Furthermore, Excel’s flexibility allows investors to customize their portfolio analysis to suit their specific needs, making it an essential tool for any investor looking to gain a deeper understanding of their portfolio’s risk and return characteristics. By learning how to calculate portfolio standard deviation in Excel, investors can unlock the full potential of this powerful tool and take their portfolio analysis to the next level.
How to Calculate Portfolio Standard Deviation in Excel: A Step-by-Step Guide
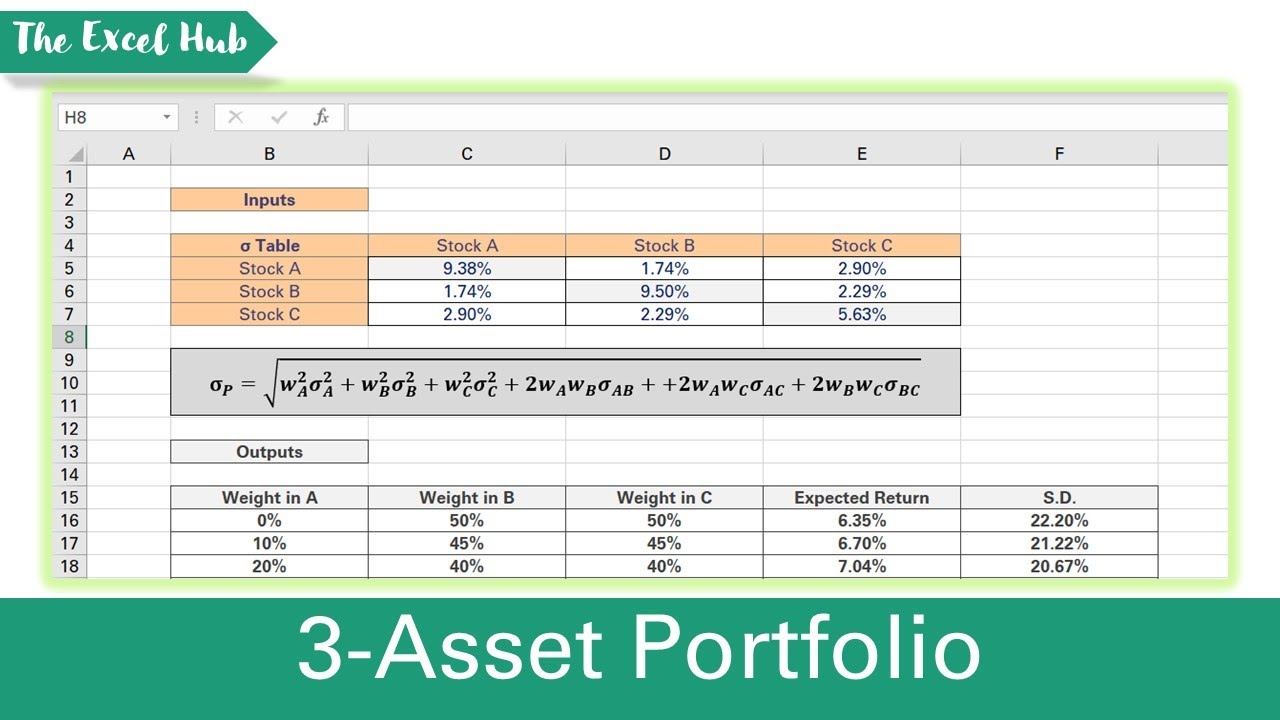
Calculating portfolio standard deviation in Excel is a straightforward process that can be accomplished with a few simple steps. To get started, investors will need to gather their portfolio data, including the returns for each asset in their portfolio. This data can be organized in an Excel spreadsheet, with each column representing a different asset and each row representing a different time period. Once the data is organized, investors can use the STDEV.S function in Excel to calculate the standard deviation of each asset. The STDEV.S function takes the range of returns for each asset as its input and returns the standard deviation of those returns.
The next step is to calculate the weighted average of the standard deviations of each asset. This can be done by multiplying the standard deviation of each asset by its weight in the portfolio and then summing the results. The weight of each asset is simply its proportion of the total portfolio. For example, if an asset makes up 20% of the portfolio, its weight would be 0.2.
The final step is to calculate the portfolio standard deviation using the following formula: portfolio standard deviation = sqrt(weight1^2 \* stdev1^2 + weight2^2 \* stdev2^2 + … + weightn^2 \* stdevn^2). This formula takes into account the correlation between the assets in the portfolio, which is essential for accurate risk analysis. By following these steps, investors can easily calculate their portfolio standard deviation in Excel and gain a deeper understanding of their portfolio’s risk profile. Learning how to calculate portfolio standard deviation in Excel is a valuable skill for any investor looking to make informed investment decisions.
Interpreting Portfolio Standard Deviation Results
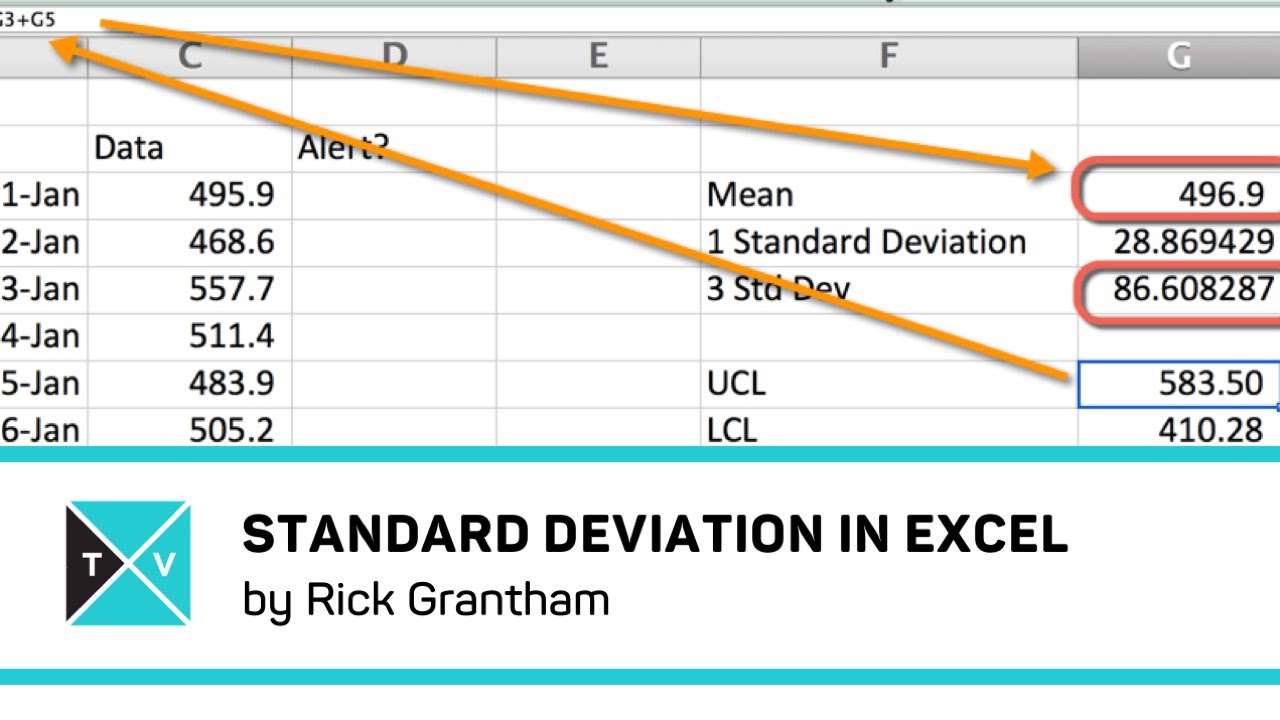
Once investors have calculated their portfolio standard deviation in Excel, they need to interpret the results to understand the risk profile of their portfolio. The portfolio standard deviation is a measure of the volatility of the portfolio, with higher values indicating greater volatility. A high standard deviation indicates that the portfolio’s returns are more spread out, meaning that there is a higher chance of extreme returns, both positive and negative. On the other hand, a low standard deviation indicates that the portfolio’s returns are more stable, with less chance of extreme returns.
In general, a portfolio standard deviation of less than 10% is considered low risk, while a standard deviation of more than 20% is considered high risk. However, the interpretation of the results also depends on the investor’s risk tolerance and investment goals. For example, a conservative investor may consider a portfolio with a standard deviation of 15% to be too risky, while an aggressive investor may consider a portfolio with a standard deviation of 25% to be acceptable.
By understanding the portfolio standard deviation, investors can make informed decisions about their investment strategy. For example, if the portfolio standard deviation is higher than desired, investors may need to rebalance their portfolio by adjusting the weights of the assets or adding new assets to reduce the risk. On the other hand, if the portfolio standard deviation is lower than desired, investors may need to take on more risk to achieve their investment goals. By learning how to calculate portfolio standard deviation in Excel and interpreting the results, investors can optimize their investment strategy and achieve their financial goals.
Common Errors to Avoid When Calculating Portfolio Standard Deviation
When calculating portfolio standard deviation in Excel, it’s essential to avoid common mistakes that can lead to inaccurate results. One of the most common errors is incorrect data entry. This can include entering returns data in the wrong format, such as entering percentages instead of decimal values. To avoid this error, investors should ensure that their returns data is entered correctly and consistently throughout the spreadsheet.
Another common error is formula errors. When using the STDEV.S function in Excel, investors need to ensure that they are using the correct syntax and that the function is applied to the correct range of cells. A simple mistake, such as forgetting to include the entire range of returns data, can lead to an inaccurate calculation of portfolio standard deviation. To avoid this error, investors should double-check their formulas and ensure that they are correct.
Additionally, investors should be aware of the assumptions underlying the portfolio standard deviation calculation. For example, the calculation assumes that the returns data is normally distributed, which may not always be the case. Investors should be aware of these assumptions and take steps to validate them, such as by using statistical tests to check for normality.
By being aware of these common errors and taking steps to avoid them, investors can ensure that their calculation of portfolio standard deviation in Excel is accurate and reliable. This, in turn, will enable them to make informed investment decisions and optimize their investment strategy. Remember, learning how to calculate portfolio standard deviation in Excel is a valuable skill for any investor, and avoiding common errors is an essential part of that process.
Using Portfolio Standard Deviation to Optimize Your Investment Strategy
Once investors have calculated their portfolio standard deviation in Excel, they can use this metric to optimize their investment strategy. One of the primary ways to do this is through diversification. By diversifying a portfolio, investors can reduce the overall risk of the portfolio, which is reflected in a lower standard deviation. This can be achieved by investing in a mix of assets with low correlations, such as stocks, bonds, and real estate.
Another way to optimize investment strategy using portfolio standard deviation is through risk management techniques. For example, investors can use the standard deviation to set risk targets, such as a maximum acceptable level of volatility. They can then use this target to adjust the portfolio’s asset allocation, ensuring that the portfolio’s risk profile aligns with their investment goals.
Additionally, investors can use portfolio standard deviation to evaluate the performance of different investment strategies. By comparing the standard deviation of different portfolios, investors can determine which strategy is most effective in managing risk. This can help investors to refine their investment approach and make more informed decisions.
For example, an investor may use portfolio standard deviation to compare the performance of a passive index fund with an actively managed fund. By calculating the standard deviation of each fund, the investor can determine which fund is more volatile and adjust their investment strategy accordingly. This type of analysis can be particularly useful for investors who are trying to balance risk and return in their portfolios.
By using portfolio standard deviation to optimize investment strategy, investors can create a more efficient portfolio that aligns with their investment goals. Whether through diversification, risk management, or performance evaluation, understanding how to calculate portfolio standard deviation in Excel is a critical skill for any investor.
Advanced Portfolio Analysis Techniques in Excel
Once investors have mastered the calculation of portfolio standard deviation in Excel, they can take their portfolio analysis to the next level by using advanced techniques. One such technique is Monte Carlo simulations, which can be used to model different investment scenarios and estimate the potential outcomes of a portfolio. By running multiple simulations, investors can gain a better understanding of the potential risks and returns of their portfolio and make more informed investment decisions.
Another advanced technique is scenario analysis, which involves analyzing the potential impact of different economic scenarios on a portfolio. For example, an investor may want to analyze the potential impact of a recession or a change in interest rates on their portfolio. By using scenario analysis, investors can identify potential vulnerabilities in their portfolio and take steps to mitigate them.
In addition to Monte Carlo simulations and scenario analysis, investors can also use Excel to perform stress testing and sensitivity analysis. Stress testing involves analyzing the potential impact of extreme market conditions on a portfolio, while sensitivity analysis involves analyzing the potential impact of changes in individual assets on the overall portfolio. By using these advanced techniques, investors can gain a deeper understanding of their portfolio’s risk profile and make more informed investment decisions.
For example, an investor may use Excel to perform a stress test on their portfolio, analyzing the potential impact of a 20% decline in the stock market on their portfolio’s value. By using this type of analysis, investors can identify potential weaknesses in their portfolio and take steps to mitigate them. Similarly, investors can use sensitivity analysis to analyze the potential impact of changes in individual assets on their portfolio’s overall risk profile.
By incorporating these advanced techniques into their portfolio analysis, investors can gain a more comprehensive understanding of their portfolio’s risk profile and make more informed investment decisions. Whether using Monte Carlo simulations, scenario analysis, stress testing, or sensitivity analysis, Excel provides a powerful tool for investors to refine their investment strategies and achieve their goals.
Conclusion: Taking Your Portfolio Analysis to the Next Level
By mastering the calculation of portfolio standard deviation in Excel, investors can take a significant step towards making more informed investment decisions. This key metric provides a powerful tool for understanding and managing risk, and can be used to optimize investment strategies and improve overall portfolio performance.
By following the steps outlined in this article, investors can learn how to calculate portfolio standard deviation in Excel, interpret the results, and avoid common errors. They can also explore advanced portfolio analysis techniques, such as Monte Carlo simulations and scenario analysis, to further refine their investment strategies.
Whether you’re a seasoned investor or just starting out, understanding how to calculate portfolio standard deviation in Excel is an essential skill for anyone looking to make the most of their investments. By applying the concepts and techniques outlined in this article, investors can gain a deeper understanding of their portfolio’s risk profile and make more informed decisions to achieve their investment goals.
Remember, calculating portfolio standard deviation in Excel is just the first step in a comprehensive portfolio analysis. By combining this metric with other advanced techniques, investors can create a powerful toolkit for managing risk and optimizing investment strategies. So why wait? Start calculating your portfolio standard deviation in Excel today and take your investment decisions to the next level!




:max_bytes(150000):strip_icc()/STDEV_Overview-5bd0de31c9e77c005104b850.jpg)