Why Geometric Return Matters in Investment Analysis
Investment analysis relies heavily on accurate performance measurement to inform investment decisions. Geometric return, a crucial yet often overlooked metric, provides a more comprehensive understanding of investment performance over time. By grasping how to calculate geometric return, investors can gain a deeper insight into their investment’s true growth, enabling them to make more informed decisions and optimize their portfolios. Unlike arithmetic return, which simply averages returns over a period, geometric return takes into account the compounding effect of returns, giving investors a more realistic picture of their investment’s growth. This distinction is vital, as it can significantly impact investment decisions and ultimately, returns. In this article, we will explore the significance of geometric return in investment analysis, its calculation, and its applications, providing investors with a powerful tool to unlock the full potential of their investments.
Understanding the Difference between Arithmetic and Geometric Returns
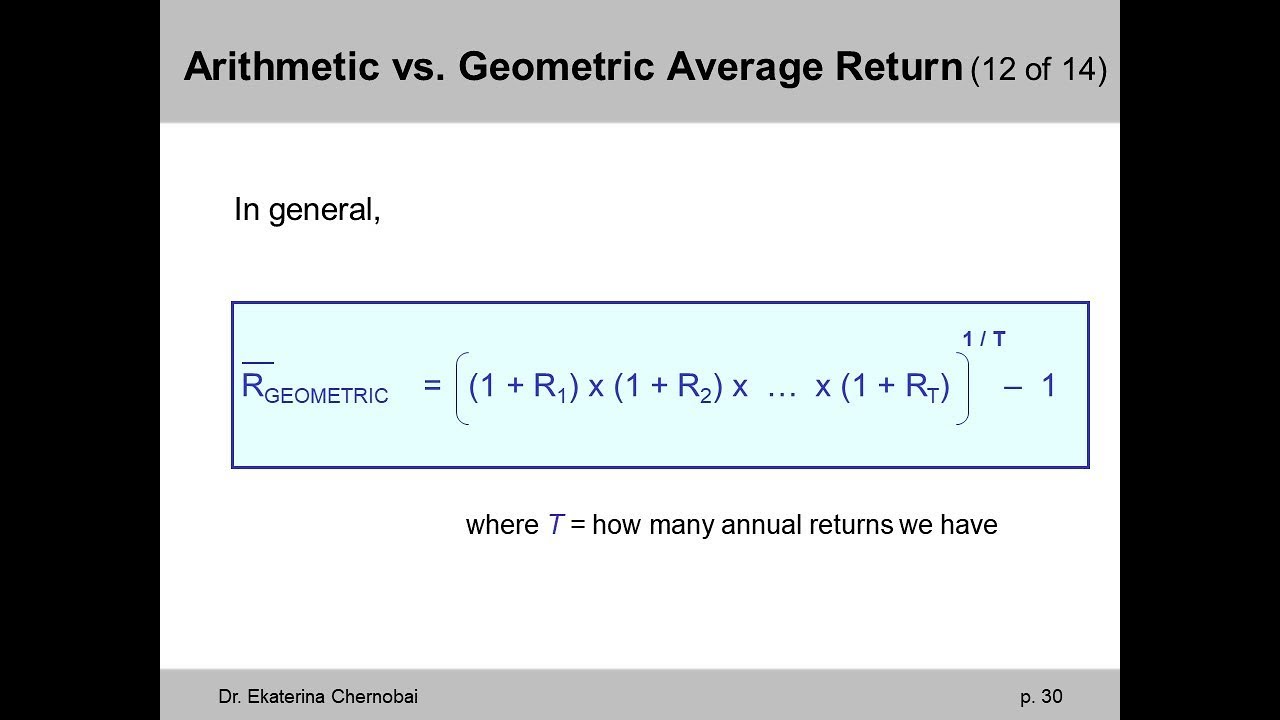
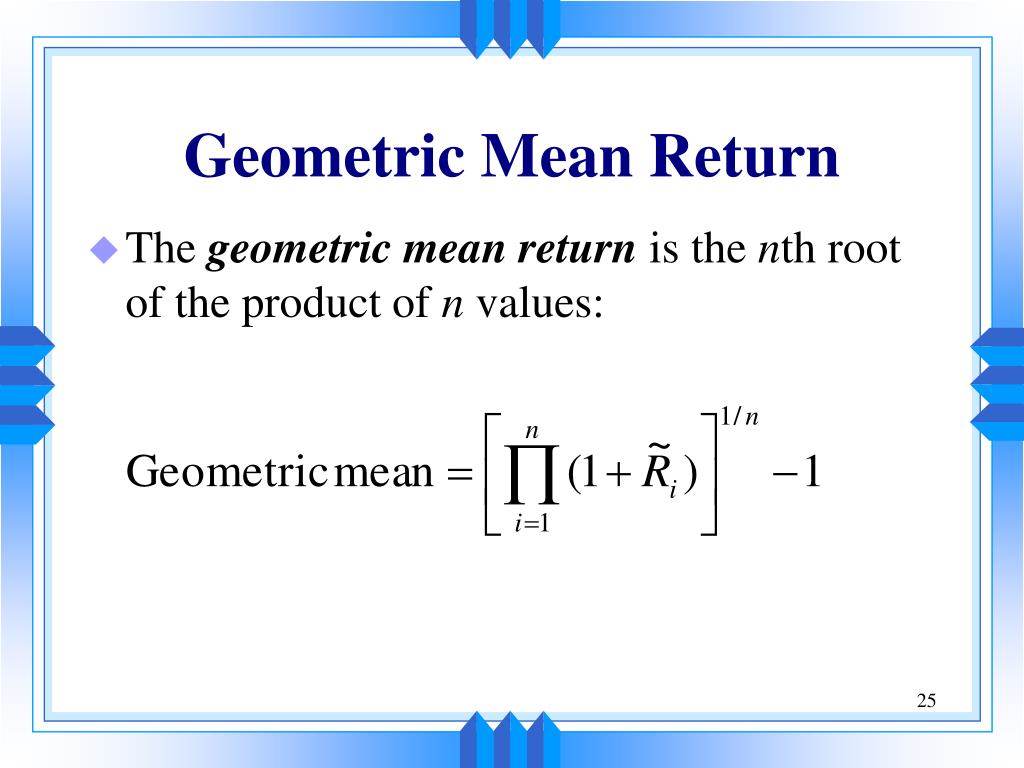
When it comes to measuring investment performance, two common metrics are used: arithmetic return and geometric return. While both provide insights into investment performance, they differ significantly in their calculation and interpretation. Arithmetic return, also known as average return, is calculated by averaging the returns of each period, whereas geometric return takes into account the compounding effect of returns over time. The arithmetic return formula is: (Sum of returns / Number of periods), whereas the geometric return formula is: [(1 + R1) × (1 + R2) × … × (1 + Rn)]^(1/n) – 1, where R1, R2, …, Rn are the returns for each period. To illustrate the difference, consider an investment with returns of 10%, -5%, and 8% over three years. The arithmetic return would be (10% – 5% + 8%) / 3 = 4.33%, while the geometric return would be [(1 + 0.10) × (1 – 0.05) × (1 + 0.08)]^(1/3) – 1 = 3.91%. This difference may seem small, but it can have a significant impact on investment decisions, especially over longer periods. Understanding the distinction between arithmetic and geometric returns is crucial for investors to accurately evaluate their investments and make informed decisions.
How to Calculate Geometric Return: A Step-by-Step Guide
Calculating geometric return is a straightforward process that requires a basic understanding of mathematical concepts. To help investors master this essential skill, we will provide a step-by-step guide on how to calculate geometric return. The formula for geometric return is: [(1 + R1) × (1 + R2) × … × (1 + Rn)]^(1/n) – 1, where R1, R2, …, Rn are the returns for each period. To apply this formula
Common Pitfalls to Avoid When Calculating Geometric Return
When it comes to calculating geometric return, investors often make mistakes that can lead to inaccurate results. To ensure accurate calculations, it’s essential to avoid common pitfalls. One of the most significant mistakes is ignoring compounding. Geometric return takes into account the compounding effect of returns over time, so ignoring this aspect can lead to a significant underestimation of investment performance. Another common error is using incorrect formulas. The geometric return formula is [(1 + R1) × (1 + R2) × … × (1 + Rn)]^(1/n) – 1, where R1, R2, …, Rn are the returns for each period. Using an arithmetic return formula instead can result in inaccurate calculations. Additionally, investors may forget to adjust for inflation or use incorrect time periods, leading to misleading results. To avoid these errors, it’s crucial to carefully follow the step-by-step guide on how
Real-World Applications of Geometric Return in Investment Decisions
Geometric return is not just a theoretical concept; it has practical applications in investment decisions. By understanding how to calculate geometric return, investors can make more informed decisions about their investments. One of the primary applications of geometric return is evaluating investment performance. By calculating the geometric return of an investment, investors can determine its true performance over time, taking into account the compounding effect of returns. This allows investors to compare different investment options and make informed decisions about which investments to hold or sell. Geometric return can also be used to optimize portfolio returns. By calculating the geometric return of a portfolio, investors can identify areas for improvement and make adjustments to maximize returns. Additionally, geometric return can be used to compare the performance of different investment managers or strategies, helping investors to select the best options for their needs. Furthermore, geometric return can be used to evaluate the performance of different asset classes, such as stocks, bonds, or real estate, allowing investors to make informed decisions about their asset allocation. By applying geometric return in these ways, investors can gain a more accurate and comprehensive understanding of their investments and make better decisions to achieve their financial goals.
Geometric Return vs. Other Investment Metrics: What’s the Difference?
When it comes to evaluating investment performance, investors have a range of metrics to choose from. Geometric return, internal rate of return (IRR), and annualized return are three commonly used metrics, each with its own strengths and weaknesses. Understanding the differences between these metrics is crucial to making informed investment decisions. Geometric return, as discussed earlier, provides a comprehensive picture of investment performance over time, taking into account the compounding effect of returns. In contrast, IRR measures the rate of return of an investment, but it does not account for the compounding effect. Annualized return, on the other hand, is a measure of the average return of an investment over a specific period, but it can be misleading if the investment has a volatile return profile. When to use each metric depends on the investment scenario. For example, geometric return is ideal for evaluating long-term investment performance, while IRR is more suitable for evaluating the performance of individual projects or investments. Annualized return, meanwhile, is useful for comparing the performance of different investments over a specific period. By understanding the differences between these metrics, investors can choose the right tool for the job and make more informed investment decisions. Additionally, knowing how to calculate geometric return can provide a more accurate and comprehensive understanding of investment performance, allowing investors to make better decisions.
Using Geometric Return to Evaluate Investment Risk and Volatility
Geometric return is not only useful for evaluating investment performance, but it can also provide valuable insights into investment risk and volatility. By calculating the geometric return of an investment, investors can gain a better understanding of the potential downside of an investment. This is because geometric return takes into account the compounding effect of returns, which can have a significant impact on investment risk. For example, an investment with a high geometric return may also come with higher volatility, which can increase the risk of losses. By understanding the geometric return of an investment, investors can make more informed decisions about the level of risk they are willing to take on. Additionally, geometric return can be used to evaluate the risk-adjusted performance of an investment, providing a more comprehensive understanding of its potential returns. This can be particularly useful for investors who are looking to optimize their portfolio returns while minimizing risk. Furthermore, geometric return can be used to compare the risk profiles of different investments, allowing investors to make more informed decisions about their investment choices. By incorporating geometric return into their investment analysis, investors can gain a more nuanced understanding of investment risk and volatility, and make more informed decisions to achieve their financial goals.
Conclusion: Unlocking the Power of Geometric Return in Investment Analysis
In conclusion, geometric return is a powerful tool in investment analysis, providing a more accurate and comprehensive understanding of investment performance over time. By mastering the calculation of geometric return, investors can gain valuable insights into the performance of their investments, make more informed decisions, and optimize their portfolio returns. Whether evaluating investment performance, comparing different investment options, or assessing investment risk and volatility, geometric return is an essential metric to have in one’s toolkit. By understanding how to calculate geometric return and avoiding common pitfalls, investors can unlock the full potential of this powerful metric and make more informed investment decisions. With its ability to provide a more accurate representation of investment performance, geometric return is an essential component of any investment analysis. By incorporating geometric return into their investment strategy, investors can gain a competitive edge and achieve their financial goals. Remember, knowing how to calculate geometric return is crucial to unlocking the power of this metric, and with practice and patience, investors can master this essential skill.