What is Geometric Mean Return and Why Does it Matter?
In investment analysis, understanding geometric mean return is crucial for making informed decisions. Geometric mean return, a type of average return, provides a more accurate representation of investment performance over time. Unlike arithmetic mean return, which simply averages returns, geometric mean return takes into account the compounding effect of returns, providing a more realistic picture of investment growth. This distinction is vital, as it directly impacts portfolio evaluation and investment strategy. By grasping the concept of geometric mean return, investors can better navigate the complexities of investment analysis and make data-driven decisions. In this article, we’ll explore the importance of geometric mean return and provide a step-by-step guide on how to calculate geometric mean return, empowering readers to unlock valuable insights into their investment portfolios.
Understanding the Formula: Breaking Down Geometric Mean Return Calculation
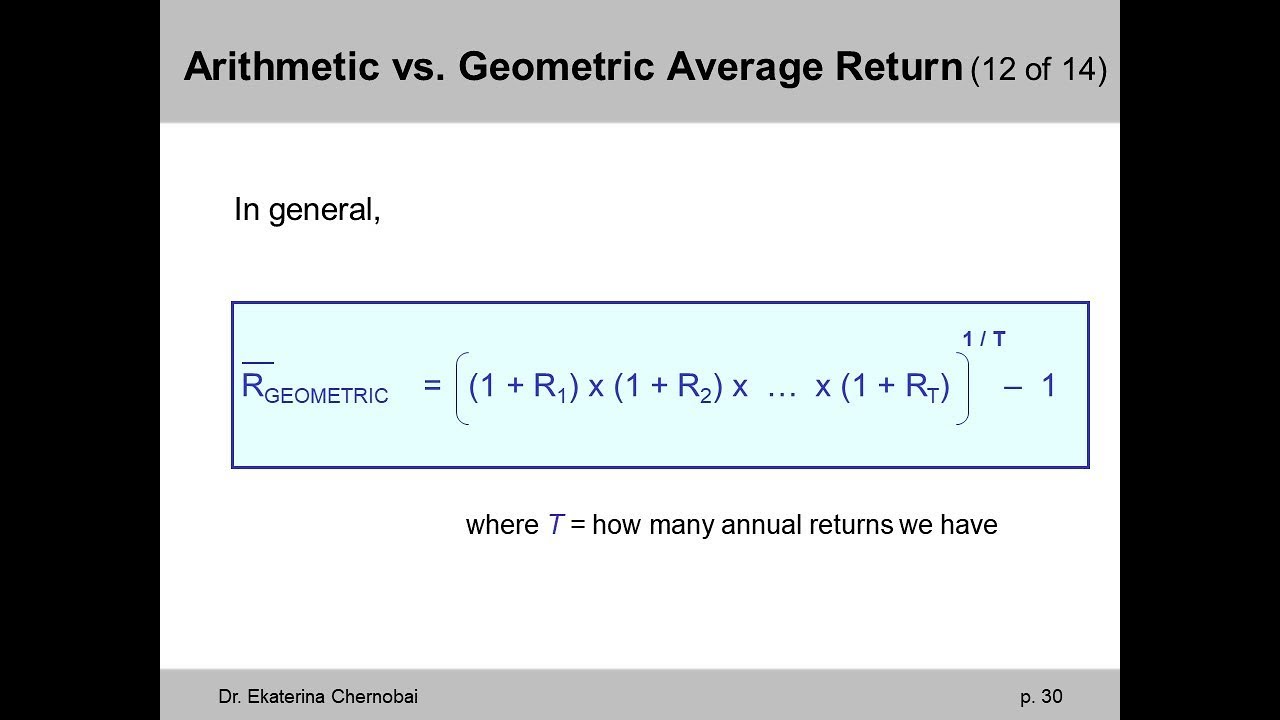
The formula for calculating geometric mean return is as follows: (1 + R1) × (1 + R2) × … × (1 + Rn)^(1/n) – 1, where R1, R2, …, Rn are the returns for each period, and n is the number of periods. This formula takes into account the compounding effect of returns, providing a more accurate representation of investment growth. To illustrate this concept, let’s consider an example. Suppose an investment returns 10% in year one, 15% in year two, and 8% in year three. To calculate the geometric mean return, we would first convert each return to a decimal (0.10, 0.15, and 0.08), then add 1 to each decimal (1.10, 1.15, and 1.08). Next, we would multiply these values together (1.10 × 1.15 × 1.08), and finally, raise the result to the power of 1/n (in this case, 1/3). The resulting value, minus 1, would be the geometric mean return. By understanding each component of this formula, investors can gain a deeper appreciation for how to calculate geometric mean return and make more informed investment decisions.
How to Calculate Geometric Mean Return: A Step-by-Step Example
To illustrate the calculation of geometric mean return, let’s consider a hypothetical investment scenario. Suppose we have an investment that returns 5% in year one, 8% in year two, and 12% in year three. To calculate the geometric mean return, we’ll follow a step-by-step process. First, we’ll convert each return to a decimal by dividing by 100 (0.05, 0.08, and 0.12). Next, we’ll add 1 to each decimal (1.05, 1.08, and 1.12). Then, we’ll multiply these values together (1.05 × 1.08 × 1.12). Finally, we’ll raise the result to the power of 1/n, where n is the number of periods (in this case, 3). The resulting value, minus 1, will be the geometric mean return. In this example, the calculation would be: ((1.05 × 1.08 × 1.12)^(1/3)) – 1 = 8.53%. This value represents the geometric mean return of our investment over the three-year period. When learning how to calculate geometric mean return, it’s essential to avoid common pitfalls, such as incorrect compounding or ignoring returns. By following this step-by-step example, investors can ensure accurate calculations and gain a deeper understanding of their investment’s performance.
The Role of Compounding in Geometric Mean Return Calculation
Compounding plays a crucial role in geometric mean return calculation, as it takes into account the effect of returns on previous returns. This concept is essential in investment analysis, as it helps investors understand how their investments grow over time. To illustrate the impact of compounding, consider an investment that returns 10% in year one and 15% in year two. If we were to calculate the total return using arithmetic mean return, we would simply average the two returns, resulting in a total return of 12.5%. However, this approach ignores the compounding effect, which can significantly affect the investment’s growth. By using geometric mean return, we can accurately account for compounding, resulting in a more realistic representation of the investment’s performance. In this example, the geometric mean return would be approximately 12.24%, highlighting the importance of considering compounding in investment analysis. When learning how to calculate geometric mean return, it’s essential to understand the role of compounding and its impact on investment growth. By doing so, investors can make more informed decisions and optimize their investment strategies.
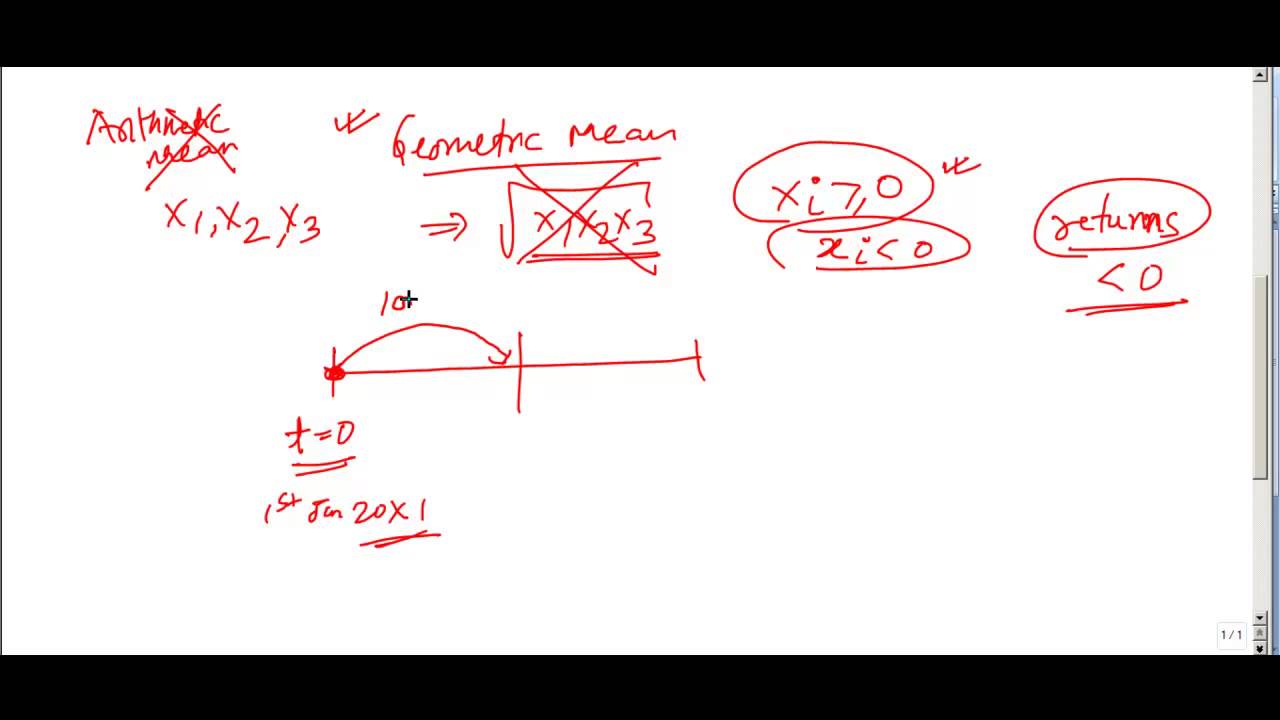
Geometric Mean Return vs. Arithmetic Mean Return: Which is More Accurate?
In investment analysis, there are two primary methods for calculating returns: geometric mean return and arithmetic mean return. While both methods have their uses, they differ significantly in their approach and accuracy. Arithmetic mean return is a simple average of returns, whereas geometric mean return takes into account the compounding effect of returns over time. This distinction is crucial, as it can significantly impact the accuracy of investment analysis. Geometric mean return is often preferred because it provides a more realistic representation of investment performance, especially over longer periods. This is because it accounts for the compounding effect, which can greatly impact investment growth. In contrast, arithmetic mean return can be misleading, as it ignores the compounding effect and may overstate or understate investment performance. For instance, an investment with returns of 10%, -5%, and 15% would have an arithmetic mean return of 6.67%, but a geometric mean return of 5.44%. This difference highlights the importance of using geometric mean return in investment analysis, especially when evaluating portfolio performance or comparing investments. By understanding the differences between geometric mean return and arithmetic mean return, investors can make more informed decisions and optimize their investment strategies. When learning how to calculate geometric mean return, it’s essential to recognize the limitations of arithmetic mean return and the benefits of using geometric mean return in investment analysis.
Real-World Applications of Geometric Mean Return in Investment Analysis
In investment analysis, geometric mean return plays a vital role in evaluating portfolio performance, assessing risk, and measuring investment returns. By understanding how to calculate geometric mean return, investors can make informed decisions and optimize their investment strategies. One of the primary applications of geometric mean return is in portfolio evaluation. By calculating the geometric mean return of a portfolio, investors can gain insights into its overall performance and make adjustments to improve returns. Additionally, geometric mean return is used in risk assessment to evaluate the volatility of an investment and determine its potential for growth. In performance measurement, geometric mean return is used to compare the returns of different investments and identify top-performing assets. For instance, a mutual fund manager may use geometric mean return to evaluate the performance of their fund and make adjustments to improve returns. In finance, geometric mean return is used to calculate the returns of complex investment products, such as options and derivatives. By understanding how to calculate geometric mean return, investors can unlock valuable insights into investment performance and make data-driven decisions. Furthermore, geometric mean return is used in benchmarking, where it is used to compare the returns of an investment to a benchmark or index. This helps investors to evaluate the performance of their investments and identify areas for improvement. Overall, the applications of geometric mean return in investment analysis are vast, and mastering its calculation is essential for making informed investment decisions.
Common Mistakes to Avoid When Calculating Geometric Mean Return
When learning how to calculate geometric mean return, it’s essential to avoid common mistakes that can lead to inaccurate results. One of the most common errors is incorrect compounding, which can significantly impact the calculation. For instance, failing to account for compounding can result in an overestimation or underestimation of investment returns. Another mistake is ignoring returns, which can lead to an incomplete picture of investment performance. Additionally, incorrect data entry or formatting can also lead to errors in calculation. To avoid these mistakes, it’s crucial to carefully review the data and ensure that the correct formula is used. Furthermore, it’s essential to understand the concept of compounding and its impact on geometric mean return. By being aware of these common pitfalls, investors can ensure accurate calculations and make informed investment decisions. For example, when calculating the geometric mean return of a portfolio, it’s essential to account for compounding and ensure that all returns are included. By doing so, investors can gain a more accurate understanding of their investment’s performance and make data-driven decisions. By mastering how to calculate geometric mean return and avoiding common mistakes, investors can unlock valuable insights into investment performance and optimize their investment strategies.
Conclusion: Mastering Geometric Mean Return for Informed Investment Decisions
In conclusion, understanding how to calculate geometric mean return is a crucial skill for investors seeking to make informed investment decisions. By grasping the concept of geometric mean return and its importance in investment analysis, investors can gain valuable insights into portfolio performance and optimize their investment strategies. Throughout this guide, we have walked through the step-by-step process of calculating geometric mean return, explored its real-world applications, and highlighted common mistakes to avoid. By mastering how to calculate geometric mean return, investors can unlock the full potential of their investments and achieve their financial goals. As investors continue to navigate the complex world of finance, it is essential to stay informed and adapt to changing market conditions. By applying the knowledge gained from this guide, investors can make data-driven decisions and stay ahead of the curve. Remember, accurate calculation of geometric mean return is key to unlocking investment insights and achieving long-term success.