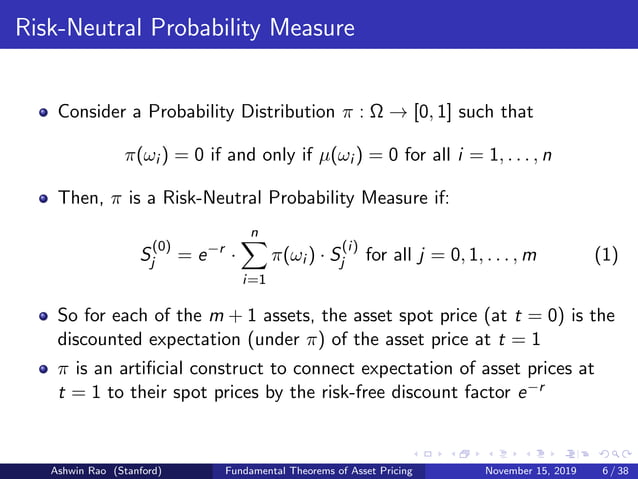
What is the Fundamental Theorem of Asset Pricing?
The fundamental theorem of asset pricing is a foundational concept in modern finance, providing a framework for understanding the behavior of financial markets and the pricing of assets. This theorem, also known as the arbitrage pricing theory (APT), is a mathematical concept that helps investors make informed decisions by identifying the expected returns on investments.
The fundamental theorem of asset pricing is significant in finance because it helps investors understand the relationship between risk and return. By recognizing that higher returns are associated with higher levels of risk, investors can make more informed decisions about their investments. This theorem also provides a basis for portfolio optimization, allowing investors to construct portfolios that balance risk and return.
In essence, the fundamental theorem of asset pricing asserts that the expected return on an asset is a function of its systematic risk, which is measured by its beta. This means that assets with higher betas, or higher levels of systematic risk, should offer higher expected returns to compensate investors for taking on that risk. Conversely, assets with lower betas should offer lower expected returns.
By understanding the fundamental theorem of asset pricing, investors can better navigate the complexities of financial markets, making more informed decisions about their investments and managing their risk exposure more effectively.
How to Apply the Fundamental Theorem in Real-World Investing
The fundamental theorem of asset pricing has numerous practical applications in real-world investing, enabling investors to make informed decisions and optimize their portfolios. One of the primary ways the theorem is used is in risk management, where it helps investors quantify and manage their exposure to systematic risk.
In portfolio optimization, the fundamental theorem of asset pricing is used to construct portfolios that balance risk and return. By identifying the expected returns on different assets and their corresponding betas, investors can create portfolios that maximize returns while minimizing risk. This is particularly useful for institutional investors, such as pension funds and endowments, which require a high degree of risk management.
Asset allocation is another area where the fundamental theorem of asset pricing is applied. By understanding the expected returns on different asset classes, such as stocks, bonds, and real estate, investors can allocate their portfolios to achieve their desired risk-return profile. This is particularly important for individual investors, who may have limited resources and require a more targeted approach to investing.
In addition to these applications, the fundamental theorem of asset pricing is also used in performance measurement and attribution. By analyzing the returns on a portfolio and attributing them to different factors, such as market risk and asset selection, investors can gain a deeper understanding of their investment performance and make more informed decisions.
Overall, the fundamental theorem of asset pricing provides a powerful framework for investors to make informed decisions and optimize their portfolios. By understanding the expected returns on different assets and their corresponding risks, investors can construct portfolios that achieve their desired risk-return profile and maximize their investment performance.
The Mathematics Behind the Fundamental Theorem
The fundamental theorem of asset pricing is built on a foundation of advanced mathematical concepts, including stochastic processes, probability theory, and linear algebra. These mathematical tools enable the theorem to capture the complexities of financial markets and provide a framework for understanding asset prices.
Stochastic processes, such as random walks and Brownian motion, are used to model the behavior of asset prices over time. These processes allow for the incorporation of uncertainty and randomness into the theorem, making it more realistic and applicable to real-world markets.
Probability theory is another crucial component of the fundamental theorem of asset pricing. It provides a framework for understanding the likelihood of different outcomes and enables the calculation of expected returns and risks. By applying probability theory, the theorem can quantify the uncertainty associated with different investments and provide a more accurate picture of their potential performance.
Linear algebra is also essential to the fundamental theorem of asset pricing, as it provides a powerful tool for analyzing and manipulating the relationships between different assets. By representing assets as vectors in a high-dimensional space, linear algebra enables the theorem to identify patterns and correlations that would be difficult to discern using other methods.
The combination of these mathematical concepts enables the fundamental theorem of asset pricing to provide a comprehensive and accurate framework for understanding asset prices. By applying these tools, the theorem can capture the complexities of financial markets and provide a powerful tool for investors seeking to make informed decisions.
Furthermore, the mathematical rigor of the fundamental theorem of asset pricing has led to its widespread adoption in finance and economics. It has been used to develop a range of financial models and tools, including the capital asset pricing model (CAPM) and the arbitrage pricing theory (APT). These models have had a profound impact on our understanding of financial markets and have enabled investors to make more informed decisions.
The Role of Arbitrage in Asset Pricing
Arbitrage plays a crucial role in the fundamental theorem of asset pricing, as it helps maintain market efficiency and ensures that asset prices reflect their true value. Arbitrage is the practice of taking advantage of price differences between two or more markets, buying an asset at a low price in one market and selling it at a higher price in another.
In the context of the fundamental theorem of asset pricing, arbitrage helps to eliminate pricing inefficiencies by exploiting mispricings in the market. When an arbitrage opportunity arises, investors can profit from the price difference, which in turn drives the prices of the assets towards their true value. This process helps to maintain market efficiency, as prices reflect the true value of the assets.
The fundamental theorem of asset pricing relies on the concept of arbitrage to derive the asset pricing equation. The theorem assumes that there are no arbitrage opportunities in the market, meaning that investors cannot earn a risk-free profit by exploiting price differences. This assumption is known as the no-arbitrage principle, which is a fundamental concept in finance.
The no-arbitrage principle has important implications for asset pricing. It implies that asset prices must reflect their true value, as any mispricing would be exploited by arbitrageurs. This, in turn, ensures that investors are rewarded for taking on risk, as the expected returns on an asset are directly related to its riskiness.
In addition, the fundamental theorem of asset pricing provides a framework for understanding the role of arbitrage in maintaining market efficiency. By assuming that there are no arbitrage opportunities, the theorem can derive the asset pricing equation, which provides a powerful tool for investors seeking to make informed decisions.
Overall, the role of arbitrage in asset pricing is crucial, as it helps maintain market efficiency and ensures that asset prices reflect their true value. The fundamental theorem of asset pricing relies on the concept of arbitrage to derive the asset pricing equation, which has had a profound impact on our understanding of financial markets.
Understanding the No-Arbitrage Principle
The no-arbitrage principle is a fundamental concept in finance that plays a crucial role in the fundamental theorem of asset pricing. It states that there are no opportunities for arbitrage in the market, meaning that investors cannot earn a risk-free profit by exploiting price differences between two or more markets.
The no-arbitrage principle has important implications for asset pricing. It implies that asset prices must reflect their true value, as any mispricing would be exploited by arbitrageurs. This, in turn, ensures that investors are rewarded for taking on risk, as the expected returns on an asset are directly related to its riskiness.
The no-arbitrage principle is used to derive the fundamental theorem of asset pricing, which provides a powerful tool for investors seeking to make informed decisions. By assuming that there are no arbitrage opportunities, the theorem can derive the asset pricing equation, which relates the expected return on an asset to its riskiness.
In addition, the no-arbitrage principle has important implications for market efficiency. It implies that markets are efficient, meaning that prices reflect all available information. This, in turn, ensures that investors have access to accurate information, which enables them to make informed decisions.
The fundamental theorem of asset pricing relies heavily on the no-arbitrage principle, as it provides a framework for understanding how asset prices are determined. By assuming that there are no arbitrage opportunities, the theorem can provide a comprehensive and accurate framework for understanding asset prices.
Furthermore, the no-arbitrage principle has been widely used in finance to develop a range of financial models and tools, including the capital asset pricing model (CAPM) and the arbitrage pricing theory (APT). These models have had a profound impact on our understanding of financial markets and have enabled investors to make more informed decisions.
In conclusion, the no-arbitrage principle is a fundamental concept in finance that plays a crucial role in the fundamental theorem of asset pricing. It provides a framework for understanding how asset prices are determined and has important implications for market efficiency and investor decision-making.
The Fundamental Theorem in Different Market Conditions
The fundamental theorem of asset pricing is a powerful tool for investors seeking to make informed decisions, but its behavior in different market conditions is a crucial aspect to consider. In this section, we will explore how the fundamental theorem of asset pricing behaves in bull and bear markets, and its implications for investors.
In a bull market, characterized by rising asset prices and high investor confidence, the fundamental theorem of asset pricing suggests that investors are rewarded for taking on risk. As asset prices increase, the expected returns on an asset also increase, reflecting the higher risk associated with investing in a bull market. This is because investors are more willing to take on risk in a bull market, driving up asset prices and expected returns.
In contrast, in a bear market, characterized by falling asset prices and low investor confidence, the fundamental theorem of asset pricing suggests that investors are penalized for taking on risk. As asset prices decrease, the expected returns on an asset also decrease, reflecting the lower risk associated with investing in a bear market. This is because investors are less willing to take on risk in a bear market, driving down asset prices and expected returns.
The fundamental theorem of asset pricing also has important implications for investors in different market conditions. In a bull market, investors may be tempted to take on excessive risk, leading to potential losses if the market reverses. The fundamental theorem of asset pricing provides a framework for understanding the risks associated with investing in a bull market, enabling investors to make more informed decisions.
In a bear market, investors may be hesitant to invest, leading to potential missed opportunities. The fundamental theorem of asset pricing provides a framework for understanding the risks associated with investing in a bear market, enabling investors to identify potential opportunities and make more informed decisions.
Furthermore, the fundamental theorem of asset pricing can be used to develop strategies for managing risk in different market conditions. For example, investors can use the theorem to develop a risk management strategy that takes into account the expected returns on an asset in different market conditions. This can help investors to minimize losses and maximize returns in both bull and bear markets.
In conclusion, the fundamental theorem of asset pricing is a powerful tool for investors seeking to make informed decisions in different market conditions. By understanding how the theorem behaves in bull and bear markets, investors can develop strategies for managing risk and maximizing returns, regardless of the market conditions.
Criticisms and Limitations of the Fundamental Theorem
The fundamental theorem of asset pricing is a cornerstone of modern finance, but it is not without its criticisms and limitations. Despite its significance, the theorem has been subject to various criticisms and challenges, which are essential to understand to appreciate its limitations.
One of the primary criticisms of the fundamental theorem of asset pricing is its reliance on simplifying assumptions. The theorem assumes that investors are rational and have access to perfect information, which is not always the case in real-world markets. Additionally, the theorem assumes that markets are efficient, which is not always true, especially in times of market stress or crisis.
Another limitation of the fundamental theorem of asset pricing is its failure to account for behavioral biases and irrational investor behavior. The theorem assumes that investors make rational decisions based on expected returns and risk, but in reality, investors are often influenced by emotions, biases, and other psychological factors.
The fundamental theorem of asset pricing also relies heavily on mathematical models, which can be prone to errors and biases. The theorem’s reliance on stochastic processes, probability theory, and linear algebra can lead to oversimplification of complex market phenomena, which can result in inaccurate predictions and investment decisions.
Furthermore, the fundamental theorem of asset pricing has been criticized for its lack of consideration of macroeconomic factors, such as inflation, interest rates, and economic growth. The theorem focuses primarily on individual asset prices and returns, neglecting the broader macroeconomic context in which markets operate.
Despite these limitations, the fundamental theorem of asset pricing remains a powerful tool for investors and financial analysts. By understanding its assumptions, simplifications, and potential biases, investors can use the theorem more effectively and make more informed investment decisions.
In conclusion, while the fundamental theorem of asset pricing is a cornerstone of modern finance, it is essential to recognize its limitations and criticisms. By acknowledging these limitations, investors can use the theorem more effectively and develop more comprehensive investment strategies that account for the complexities of real-world markets.
Conclusion: The Enduring Importance of the Fundamental Theorem
The fundamental theorem of asset pricing is a cornerstone of modern finance, providing a framework for understanding the behavior of financial markets and the pricing of assets. Despite its limitations and criticisms, the theorem remains a powerful tool for investors and financial analysts, helping them to make informed decisions and navigate complex market conditions.
The significance of the fundamental theorem of asset pricing lies in its ability to provide a unified framework for understanding the behavior of financial markets. By recognizing the importance of risk and return, the theorem helps investors to make informed decisions about asset allocation, portfolio optimization, and risk management. The theorem’s emphasis on the no-arbitrage principle and the concept of arbitrage also helps to maintain market efficiency, ensuring that prices reflect all available information.
The fundamental theorem of asset pricing has made significant contributions to the field of finance, shaping our understanding of financial markets and informing investment decisions. Its continued relevance in modern financial markets is a testament to its enduring importance, as investors and financial analysts continue to rely on its principles to navigate the complexities of the market.
In conclusion, the fundamental theorem of asset pricing is a foundational concept in finance, providing a framework for understanding the behavior of financial markets and the pricing of assets. Its significance, contributions, and continued relevance make it an essential tool for investors and financial analysts, helping them to make informed decisions and achieve their investment goals.
As the financial markets continue to evolve, the fundamental theorem of asset pricing will remain a vital component of investment decision-making, providing a foundation for understanding the complex interactions between risk, return, and market efficiency. By recognizing the importance of this theorem, investors and financial analysts can make more informed decisions, navigate complex market conditions, and achieve their investment goals.