Understanding the Expected Rate of Return: A Key to Informed Investing
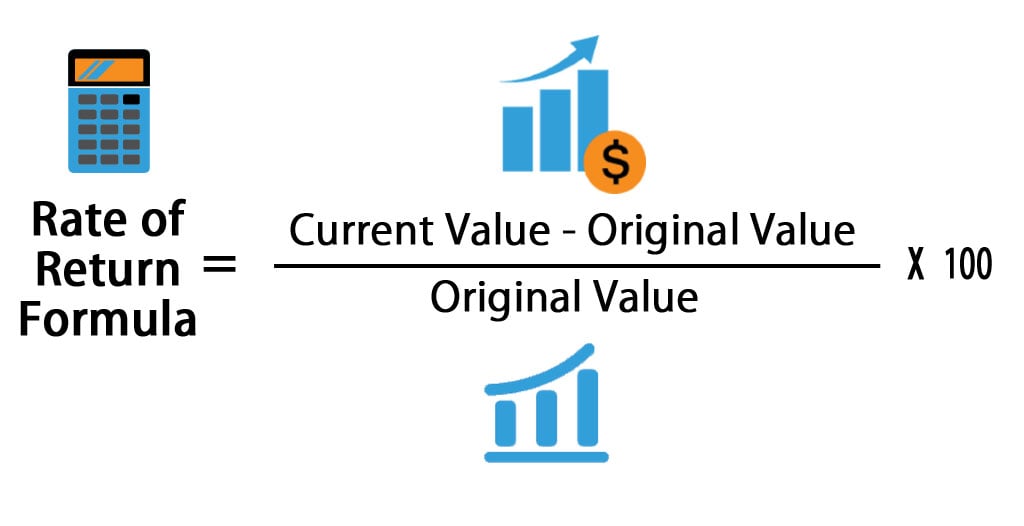
In the world of investing, making informed decisions is crucial to achieving long-term success. One of the most critical components of investment analysis is understanding the expected rate of return. The expected rate of return is a measure of the potential gain or loss of an investment over a specific period. It provides investors with a framework for evaluating the potential performance of an investment and making informed decisions about their portfolio.
The expected rate of return is a fundamental concept in finance, and it plays a vital role in portfolio optimization. By understanding the expected rate of return, investors can assess the potential risk and reward of an investment, making it easier to create a diversified portfolio that aligns with their investment goals. In essence, the expected rate of return serves as a guide for investors, helping them to navigate the complex world of investing and make informed decisions about their financial future.
When evaluating investments, it’s essential to consider the expected rate of return and standard deviation together. This powerful combination provides a comprehensive understanding of an investment’s potential risks and rewards, enabling investors to make more informed decisions about their portfolio. By grasping the expected rate of return and standard deviation, investors can optimize their portfolio, minimize risk, and maximize returns.
Deciphering Standard Deviation: The Role of Volatility in Investment Risk
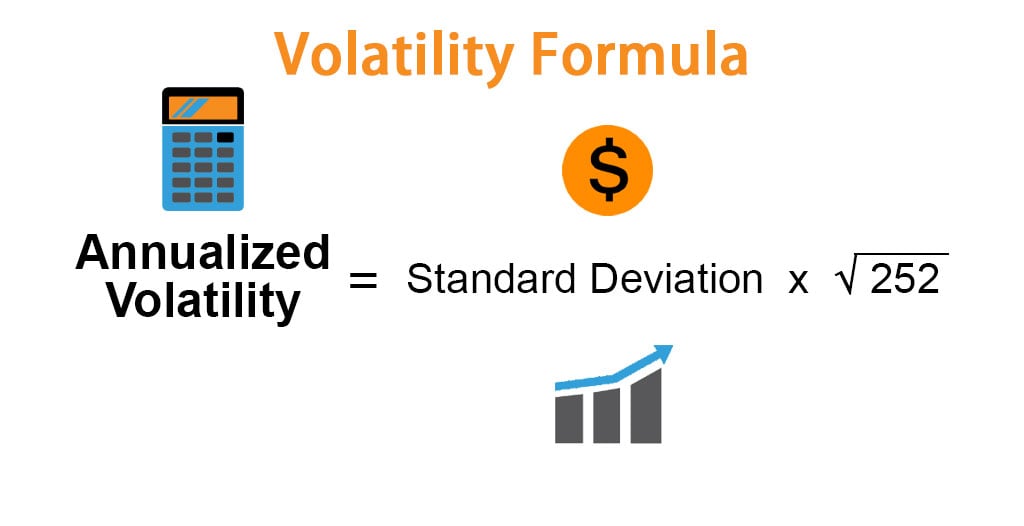
Standard deviation is a crucial concept in finance that measures the volatility of an investment. It provides investors with a sense of the potential risk associated with an investment, allowing them to make informed decisions about their portfolio. In essence, standard deviation is a statistical measure that quantifies the amount of uncertainty or risk involved in an investment.
The relationship between expected rate of return and standard deviation is a critical aspect of investment analysis. Investments with higher expected rates of return typically come with higher standard deviations, indicating greater volatility and risk. Conversely, investments with lower expected rates of return tend to have lower standard deviations, indicating less volatility and risk. This inverse relationship is essential to understand, as it enables investors to strike a balance between risk and potential returns.
Standard deviation has a profound impact on the overall risk profile of an investment. A high standard deviation indicates that an investment’s returns can fluctuate significantly, making it a riskier investment. On the other hand, a low standard deviation suggests that an investment’s returns are more stable, making it a less risky investment. By understanding standard deviation, investors can better assess the potential risks and rewards of an investment, making it easier to create a diversified portfolio that aligns with their investment goals.
In the context of expected rate of return and standard deviation, investors can use this information to optimize their portfolio. By combining investments with different expected rates of return and standard deviations, investors can create a portfolio that balances risk and potential returns. This approach enables investors to maximize returns while minimizing risk, ultimately achieving long-term investment success.
How to Calculate Expected Rate of Return and Standard Deviation: A Step-by-Step Guide
Calculating expected rate of return and standard deviation is a crucial step in investment analysis. These metrics provide investors with valuable insights into the potential performance of an investment, enabling them to make informed decisions about their portfolio. In this section, we will provide a step-by-step guide on how to calculate expected rate of return and standard deviation, including examples and formulas.
To calculate the expected rate of return, investors can use the following formula:
E(R) = Σ (Probability of Outcome x Rate of Return)
Where E(R) is the expected rate of return, Probability of Outcome is the likelihood of a particular outcome, and Rate of Return is the potential return of the investment.
For example, let’s say an investor is considering two possible outcomes for an investment: a 20% return with a 60% probability and a 10% return with a 40% probability. Using the formula above, the expected rate of return would be:
E(R) = (0.6 x 20%) + (0.4 x 10%) = 14%
Standard deviation, on the other hand, can be calculated using the following formula:
σ = √(Σ (Probability of Outcome x (Rate of Return – E(R))^2))
Where σ is the standard deviation, Probability of Outcome is the likelihood of a particular outcome, Rate of Return is the potential return of the investment, and E(R) is the expected rate of return.
Using the same example as above, the standard deviation would be:
σ = √((0.6 x (20% – 14%)^2) + (0.4 x (10% – 14%)^2)) = 5.48%
By calculating expected rate of return and standard deviation, investors can gain a deeper understanding of the potential risks and rewards of an investment. This information can be used to optimize a portfolio, minimize risk, and maximize returns.
In the context of expected rate of return and standard deviation, investors can use these metrics to evaluate the potential performance of different investments and create a diversified portfolio that aligns with their investment goals. By understanding how to calculate these metrics, investors can make more informed decisions about their investments and achieve long-term investment success.
The Relationship Between Expected Rate of Return and Standard Deviation: What You Need to Know
The relationship between expected rate of return and standard deviation is a critical aspect of investment analysis. Understanding this relationship is essential for making informed investment decisions and optimizing a portfolio. In this section, we will explore the inverse relationship between expected rate of return and standard deviation, and how this impacts investment decisions.
Expected rate of return and standard deviation are two interconnected concepts that provide investors with valuable insights into the potential performance of an investment. The expected rate of return represents the potential return of an investment, while standard deviation measures the volatility or risk associated with that investment. The relationship between these two metrics is inverse, meaning that investments with higher expected rates of return typically come with higher standard deviations, and vice versa.
This inverse relationship has significant implications for investment decisions. Investments with high expected rates of return often come with higher levels of risk, which can result in significant losses if the investment does not perform as expected. On the other hand, investments with lower expected rates of return tend to be less risky, but may not provide the same level of returns. By understanding the relationship between expected rate of return and standard deviation, investors can make more informed decisions about their investments and strike a balance between risk and potential returns.
For example, an investor considering two investments with different expected rates of return and standard deviations may choose to allocate their portfolio accordingly. If the investor is willing to take on more risk in pursuit of higher returns, they may allocate a larger portion of their portfolio to the investment with the higher expected rate of return and standard deviation. Conversely, if the investor is risk-averse, they may allocate a larger portion of their portfolio to the investment with the lower expected rate of return and standard deviation.
In conclusion, the relationship between expected rate of return and standard deviation is a critical aspect of investment analysis. By understanding this relationship, investors can make more informed decisions about their investments, manage risk, and maximize returns. In the next section, we will explore strategies for managing risk and maximizing returns, including diversification, asset allocation, and risk-reward analysis.
Managing Risk and Maximizing Returns: Strategies for Optimizing Your Portfolio
Effective portfolio management requires a delicate balance between risk and potential returns. By understanding the expected rate of return and standard deviation of an investment, investors can develop strategies to manage risk and maximize returns. In this section, we will explore various strategies for optimizing a portfolio, including diversification, asset allocation, and risk-reward analysis.
Diversification is a key strategy for managing risk in a portfolio. By spreading investments across different asset classes, sectors, and geographic regions, investors can reduce their exposure to any one particular investment. This can help to minimize losses and maximize returns over the long term. For example, an investor may allocate 60% of their portfolio to stocks, 30% to bonds, and 10% to alternative investments, such as real estate or commodities.
Asset allocation is another important strategy for managing risk and maximizing returns. By allocating a portfolio across different asset classes, investors can optimize their returns based on their risk tolerance and investment goals. For example, an investor with a high risk tolerance may allocate a larger portion of their portfolio to stocks, while an investor with a low risk tolerance may allocate a larger portion to bonds.
Risk-reward analysis is a critical component of portfolio management. By evaluating the expected rate of return and standard deviation of an investment, investors can determine the potential risks and rewards associated with that investment. This can help investors to make informed decisions about their investments and optimize their portfolio for maximum returns. For example, an investor may use a risk-reward analysis to determine that an investment with a high expected rate of return and standard deviation is not worth the potential risks, and instead allocate their portfolio to a lower-risk investment with a lower expected rate of return.
In addition to these strategies, investors can also use expected rate of return and standard deviation to optimize their portfolio through regular rebalancing. By regularly reviewing their portfolio and rebalancing their investments, investors can ensure that their portfolio remains aligned with their investment goals and risk tolerance. This can help to maximize returns over the long term and minimize losses.
In conclusion, managing risk and maximizing returns requires a deep understanding of expected rate of return and standard deviation. By using strategies such as diversification, asset allocation, and risk-reward analysis, investors can optimize their portfolio and achieve long-term investment success. In the next section, we will explore real-world examples of how expected rate of return and standard deviation are used in investment decisions.
Real-World Examples of Expected Rate of Return and Standard Deviation in Action
In the world of finance, expected rate of return and standard deviation are not just theoretical concepts, but rather essential tools used by investors and financial professionals to make informed investment decisions. In this section, we will explore real-world examples of how expected rate of return and standard deviation are used in investment decisions, including case studies and industry examples.
Case Study: Apple Inc.
In 2019, Apple Inc. had an expected rate of return of 10% and a standard deviation of 15%. This meant that investors could expect a 10% return on their investment in Apple, but with a level of volatility that could result in a return as high as 25% or as low as -5%. By understanding the expected rate of return and standard deviation of Apple’s stock, investors could make informed decisions about whether to invest in the company.
Industry Example: Real Estate Investment Trusts (REITs)
Real Estate Investment Trusts (REITs) are a type of investment vehicle that allows individuals to invest in real estate without directly owning physical properties. REITs often have a lower expected rate of return compared to stocks, but with a lower standard deviation, making them a more stable investment option. For example, a REIT with an expected rate of return of 5% and a standard deviation of 5% may be attractive to investors seeking a lower-risk investment option.
Practical Application: Portfolio Optimization
Expected rate of return and standard deviation are also used in portfolio optimization, where investors seek to maximize returns while minimizing risk. By analyzing the expected rate of return and standard deviation of different investments, investors can create a diversified portfolio that balances risk and potential returns. For example, an investor may allocate 60% of their portfolio to stocks with a higher expected rate of return and standard deviation, and 40% to bonds with a lower expected rate of return and standard deviation.
In conclusion, expected rate of return and standard deviation are essential concepts in finance that have real-world applications in investment decisions. By understanding these concepts, investors can make informed decisions about their investments and optimize their portfolios for maximum returns. In the next section, we will explore common mistakes to avoid when calculating expected rate of return and standard deviation.
Common Mistakes to Avoid When Calculating Expected Rate of Return and Standard Deviation
Calculating expected rate of return and standard deviation is a crucial step in making informed investment decisions. However, even small mistakes in the calculation process can lead to inaccurate results, which can have a significant impact on investment outcomes. In this section, we will highlight common mistakes to avoid when calculating expected rate of return and standard deviation.
Error 1: Inaccurate Data
One of the most common mistakes is using inaccurate or outdated data to calculate expected rate of return and standard deviation. This can lead to incorrect results, which can misguide investment decisions. It is essential to use reliable and up-to-date data sources to ensure accurate calculations.
Error 2: Incorrect Formula Application
Another mistake is incorrectly applying the formulas for calculating expected rate of return and standard deviation. This can lead to errors in calculation, which can result in inaccurate results. It is crucial to understand the formulas and apply them correctly to ensure accurate calculations.
Error 3: Failure to Consider Time Horizon
The time horizon of an investment is a critical factor in calculating expected rate of return and standard deviation. Failing to consider the time horizon can lead to inaccurate results, as the expected rate of return and standard deviation can vary significantly over different time periods.
Error 4: Ignoring Risk-Free Rate
The risk-free rate is an essential component in calculating expected rate of return and standard deviation. Ignoring the risk-free rate can lead to inaccurate results, as it fails to account for the return on a risk-free investment.
Error 5: Misinterpreting Results
Finally, misinterpreting the results of expected rate of return and standard deviation calculations can lead to incorrect investment decisions. It is essential to understand the results in the context of the investment and to consider other factors, such as risk tolerance and investment goals.
In conclusion, calculating expected rate of return and standard deviation requires attention to detail and a thorough understanding of the formulas and concepts involved. By avoiding common mistakes, investors can ensure accurate calculations and make informed investment decisions. In the next section, we will summarize the importance of understanding expected rate of return and standard deviation in investment decisions.
https://www.youtube.com/watch?v=MzNy9Ngf2A8
Conclusion: Mastering Expected Rate of Return and Standard Deviation for Long-Term Investment Success
In conclusion, understanding expected rate of return and standard deviation is crucial for making informed investment decisions and achieving long-term investment success. By grasping these concepts, investors can optimize their portfolios, manage risk, and maximize returns. It is essential to remember that expected rate of return and standard deviation are interconnected, and a thorough understanding of their relationship is vital for investment success.
Throughout this guide, we have explored the importance of expected rate of return and standard deviation in investment decisions, including how to calculate them, their relationship, and common mistakes to avoid. By applying this knowledge, investors can create a diversified portfolio that balances risk and potential returns, ultimately leading to long-term investment success.
As investors navigate the complex world of finance, it is crucial to stay informed and adapt to changing market conditions. By mastering expected rate of return and standard deviation, investors can make informed decisions, minimize risk, and maximize returns. Remember, a deep understanding of these concepts is key to achieving long-term investment success and securing a prosperous financial future.