Understanding Portfolio Risk: Measuring Volatility
Portfolio risk is an essential concept for investors to understand, as it reflects the potential for an investment’s value to fluctuate. A key metric for measuring this risk is the standard deviation, which quantifies the dispersion or spread of returns around the average return of a portfolio. Essentially, standard deviation helps investors understand how much the returns of their portfolio are likely to deviate from the average return they’ve experienced. A higher standard deviation implies a higher degree of volatility, indicating that the portfolio’s returns can swing widely, while a lower standard deviation indicates more stable, consistent returns. It is important to note that knowing how to calculate standard deviation of portfolio is a fundamental step in assessing the inherent risks within an investment strategy. Before diving into the calculations, it’s important to understand the framework in which risk is measured.
Investors need to understand that standard deviation provides a statistical measure of the historical volatility of a portfolio. It’s a way to gauge the past performance’s variability, offering a sense of the potential ups and downs one might expect in the future. It’s this dispersion of returns, as captured by the standard deviation, that allows investors to understand the overall risk landscape. It is not just about the potential for loss; it’s also about the potential for gains, which can be volatile. The ability to calculate standard deviation of portfolio is key to understanding that historical volatility does not guarantee future volatility, but it provides valuable insights into the risk profile of an investment portfolio. Therefore, learning how to accurately calculate standard deviation of portfolio is the bedrock upon which more advanced risk management strategies are built.
The Building Blocks: Return Calculation
Before delving into how to calculate standard deviation of portfolio, it’s essential to understand the foundation upon which it’s built: the returns of the assets within a portfolio. The return of an asset over a specific period is the percentage change in its value during that timeframe. This is calculated by subtracting the initial value of the asset from its final value, and then dividing that result by the initial value. For example, if an asset was initially worth $100 and is now worth $110, its return is ($110-$100)/$100 = 10%. Calculating portfolio return involves more than simply averaging individual asset returns; it requires a weighted average. The weight of each asset is the proportion of the total portfolio value it represents. If a portfolio contains two assets, asset A with 30% of the total value and a return of 10% and asset B with 70% of the total value and a return of 5%, the portfolio return would be calculated as (0.30 * 0.10) + (0.70 * 0.05) = 0.065 or 6.5%. This accurate calculation of portfolio return, utilizing weights, is a necessary first step in order to calculate standard deviation of portfolio, as it serves as the baseline for measuring volatility.
The weighting process is vital because it reflects the actual impact each asset’s performance has on the overall portfolio. Assets with higher weights will contribute more significantly to the portfolio’s return, either positively or negatively. This weighted return then serves as the benchmark from which we measure deviations when we calculate standard deviation of portfolio. Without considering weights, the resulting standard deviation would be inaccurate. For instance, using the previous example if we just simply averaged the returns, (10% + 5%) / 2 = 7.5% , this would skew the calculation and ultimately not accurately reflect how the portfolio performed. The weighted portfolio return is crucial to understanding the overall performance and is a critical input to calculate standard deviation of portfolio. Understanding the fundamental process for calculating both individual and portfolio returns paves the way for understanding more complex concepts. Correct calculation of portfolio return, by using asset weights, allows for the next steps in calculating portfolio risk.
Delving Deeper: Understanding Variance
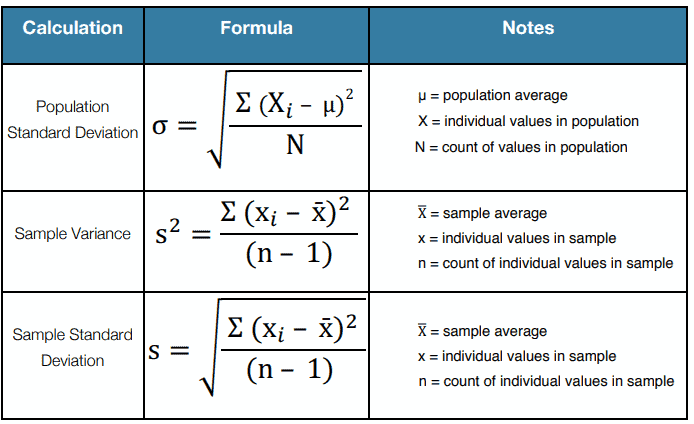
Before we can calculate standard deviation of portfolio, it’s essential to grasp the concept of variance, which serves as a crucial stepping stone. Variance quantifies the dispersion of returns around a portfolio’s average return, specifically by measuring the average of the squared differences from that average. Unlike simply calculating the average deviation, which would always sum to zero, squaring the differences ensures that both positive and negative deviations contribute positively to the variance, effectively emphasizing the magnitude of the deviations. This provides a clearer picture of the overall variability within the portfolio’s returns. For example, consider a simplified portfolio with three hypothetical period returns: 5%, 10%, and -2%. First, we need to calculate the average return. That is achieved by summing the returns (5% + 10% + (-2%) = 13%) and then dividing by the number of periods (3), resulting in an average return of approximately 4.33%. To calculate variance, we subtract the average return from each individual return, square the result, and then find the average of these squared differences. So, we have: (5% – 4.33%)² = 0.004489, (10% – 4.33%)² = 0.321489, and (-2% – 4.33%)² = 0.003969. The variance would then be the sum of these squared differences divided by the number of returns: (0.004489 + 0.321489 + 0.003969) / 3 = 0.1100. Understanding the variance is important as it prepares us to calculate standard deviation of portfolio, which is simply the square root of this value.
The variance figure itself, while informative, is not directly comparable to the original returns because it is expressed in squared units. This is where calculating standard deviation of portfolio becomes crucial, as it is expressed in the same units as the returns, making it easily interpretable. In the prior hypothetical example, we calculated a variance of approximately 0.1100. To calculate standard deviation of portfolio we would find the square root of that number. This step is crucial for a better understanding of portfolio risk because it transforms the squared deviations of variance into the more relatable format of percentages and allows us to easily see how wide the distribution of returns is around the average return. To continue with the example, a variance of 0.1100 would convert to a standard deviation of 0.3316, or about 33.16%. This value shows that, on average, the returns of this hypothetical portfolio will deviate by about 33.16% from its average return. This high volatility figure suggests this portfolio has a large degree of risk. By understanding both the variance and how to calculate standard deviation of portfolio, investors can assess risk much more effectively.
Step-by-Step: Calculating Portfolio Standard Deviation
The process to calculate standard deviation of portfolio involves a few key steps, building upon the concepts of returns and variance. The standard deviation is essentially the square root of the variance, which we established measures the dispersion of returns around the average. The formula for standard deviation of portfolio is: σp = √∑[wi * (Ri – Rp)²] where σp is the portfolio standard deviation, wi is the weight of asset i in the portfolio, Ri is the return of asset i, and Rp is the return of the portfolio. To calculate standard deviation of portfolio, start by calculating the portfolio return. This was previously calculated as the weighted average return of all assets in your portfolio. For instance, let’s assume we have two assets in our portfolio: Asset A with a weight of 60% and a return of 10%, and Asset B with a weight of 40% and a return of 5%. The portfolio return is then (0.60 * 0.10) + (0.40 * 0.05) = 0.08, or 8%. Next, calculate the variance by subtracting the portfolio return from each asset’s return, squaring the difference, and multiplying by the asset’s weight. For asset A: (0.10 – 0.08)² * 0.60 = 0.00024 and for asset B: (0.05 – 0.08)² * 0.40 = 0.00036. The sum of the variances is 0.0006, and to arrive at the portfolio standard deviation, take the square root of the variance: √0.0006 = 0.0245, or 2.45%. This result indicates the volatility of this hypothetical portfolio, providing insight into how much the portfolio’s returns are expected to fluctuate.
The step-by-step process to calculate standard deviation of portfolio, though seemingly complex, becomes manageable when broken down. First, it’s crucial to ensure accurate calculation of each asset’s return and the overall portfolio return. As mentioned before, this is a weighted average, and it is calculated by considering the proportion or weight of each asset in the total investment. After the returns are calculated, the calculation of variance is next, by using the formula mentioned earlier, where each return’s difference from the portfolio’s average return is squared and weighted, and then added together, and as a final step, calculating the square root, which is the portfolio standard deviation. Now, using the example, you would first calculate the return of each asset in the portfolio, subtract from it the portfolio return, and square the resulting number, and then multiply it by the weight of the asset in the portfolio. Doing this for all the assets and summing them together will give you the variance. In our previous example, the variance came out to be 0.0006, and the square root of this is approximately 0.0245, or 2.45%, which is the standard deviation of the portfolio. A standard deviation of 2.45% suggests a relatively low level of volatility. When you calculate standard deviation of portfolio, you are essentially measuring its risk, and this value is critical for risk management and making informed decisions.
The Importance of Correlation
Understanding the correlation between assets within a portfolio is paramount when aiming to accurately calculate standard deviation of portfolio, a key indicator of its overall risk. Correlation measures how the returns of two assets move in relation to each other. A positive correlation suggests that assets tend to move in the same direction, while a negative correlation implies they move in opposite directions. The degree of correlation significantly influences the portfolio’s standard deviation; simply combining assets does not automatically reduce risk if they are highly correlated. In fact, assets that exhibit a positive correlation will often amplify each other’s volatility, leading to higher portfolio standard deviation, hence increased risk. Conversely, diversification through assets with low or negative correlations has the potential to lower the overall portfolio standard deviation. This happens because the losses in one asset may be offset by gains in another, thus stabilizing the portfolio’s value and reducing the need to calculate standard deviation of portfolio that would otherwise be more severe.
For instance, consider the typical low correlation between the stock market and government bonds. During periods of economic uncertainty, investors often move out of stocks and into the perceived safety of bonds, leading to stock prices falling while bond prices rise; this negative correlation provides a natural hedge within a diversified portfolio. Another good example might be the low correlation observed between precious metals, such as gold, and the broader stock market. Gold often acts as a safe-haven asset, increasing in value during times of economic downturn when stocks may be declining. Adding gold to a stock portfolio may help to reduce the portfolio’s overall risk and provide a smoother overall return. These examples highlight how strategic asset allocation, with a focus on assets with low or negative correlations, can be crucial for managing and reducing a portfolio’s standard deviation. Therefore, when aiming to calculate standard deviation of portfolio, it is not just about the individual risk of assets, but also their relationships with each other.
Analyzing and Interpreting Results
Understanding how to interpret the calculated standard deviation of a portfolio is crucial for effective risk management. The standard deviation, expressed as a percentage, represents the degree of volatility or dispersion of returns around the average portfolio return. A higher standard deviation indicates that the portfolio’s returns have historically fluctuated more widely, implying greater risk. Conversely, a lower standard deviation suggests that the returns have been more stable and predictable, indicating a lower risk profile. For instance, if a portfolio has a standard deviation of 15%, it implies that returns have typically varied by approximately 15 percentage points from the average return. This volatility can translate into larger potential gains but also more significant potential losses. When deciding to calculate standard deviation of a portfolio, investors should understand this relationship between volatility and risk, realizing that it’s crucial to match the level of portfolio risk with their investment goals and risk tolerance. Different investors might find different levels of volatility acceptable. Someone with a long-term horizon may be more comfortable with higher standard deviations, whereas a more risk-averse investor or one with a shorter time horizon would probably prefer a lower standard deviation for a portfolio.
Investors can utilize the calculated standard deviation of a portfolio to make informed decisions concerning diversification and risk management. By analyzing how the standard deviation changes as assets are added or subtracted from a portfolio, investors can determine whether their portfolio is becoming more or less risky. The aim is to find a balance between risk and return that aligns with an individual’s investment strategy. For instance, investors might look at the standard deviation of their current holdings to assess whether diversification strategies are needed to reduce overall risk. However, it’s important to note that the standard deviation is not a perfect measure of risk, it primarily quantifies historical volatility, and doesn’t necessarily predict future volatility or account for tail risks (extreme, less probable events). It also assumes a normal distribution of returns, which might not be accurate for all types of assets or market conditions. Despite these limitations, it remains an essential tool in the arsenal of portfolio management and provides a valuable reference point for any investor who wants to calculate standard deviation of portfolio and wants to understand the volatility and potential risk within an investment.
Tools and Methods for Streamlined Calculations
Calculating the standard deviation of a portfolio can be complex when done manually, especially with a large number of assets or data points. Fortunately, several tools and methods streamline this process, enhancing both efficiency and accuracy. Spreadsheet software, such as Microsoft Excel and Google Sheets, provides built-in functions that greatly simplify these calculations. In Excel, for instance, the `STDEV.S` function is used to calculate the standard deviation of a sample, while in Google Sheets, the `STDEV` function serves the same purpose. To calculate the standard deviation of a portfolio using these tools, you would first organize your data, including asset returns and their corresponding weights, in a structured table. Then, after calculating the portfolio return, and the variance, the standard deviation can be obtained by applying the appropriate function to the calculated returns.
These spreadsheet applications not only automate the core calculation but also reduce the risk of human error. The benefit of using these functions to calculate standard deviation of portfolio is that they handle the repetitive and intricate mathematical steps, allowing investors to focus more on analysis and interpretation rather than the computation itself. Additionally, spreadsheet software allows for easy recalculation when new data or weighting adjustments are introduced, providing a dynamic view of portfolio risk over time. For example, you might have columns for asset names, asset weights, and their periodic returns. A separate column would calculate the weighted return. After doing this, and calculating the average return, another column would use the previous results to apply the `STDEV.S` (or `STDEV`) function to calculate the standard deviation. This process can be further enhanced by using the `VAR.S` or `VAR` function for variance calculation, which is often needed to help calculate standard deviation of portfolio.
Beyond spreadsheet software, specialized financial software and programming languages like Python with libraries such as Pandas and NumPy, can be employed for more complex portfolios or when dealing with vast datasets. These tools provide greater flexibility and allow for more intricate risk modeling. While using the spreadsheet method is user friendly and often sufficient for individual investors, institutional investors or professional financial managers may prefer specialized software for their more advanced needs. Regardless of the tool, the ability to quickly and accurately calculate standard deviation of portfolio is crucial in modern finance, enabling investors to make well-informed decisions regarding portfolio diversification and risk management. The use of these tools minimizes human error, saves time and facilitates a continuous assessment of portfolio risk as new data becomes available. It is thus essential to employ the proper tool to calculate the standard deviation of portfolio.
Practical Applications and Risk Management
Calculating the standard deviation of a portfolio stands as a cornerstone of robust risk management and informed investment strategy. The ability to accurately measure portfolio dispersion, as facilitated by standard deviation, allows investors to gauge the potential volatility of their holdings. It’s crucial to understand that a higher standard deviation indicates greater potential price swings, and thus a higher degree of risk. Conversely, a lower standard deviation suggests that a portfolio’s returns are more tightly clustered around its average, which implies lower risk. This knowledge is invaluable for crafting a portfolio that aligns with individual risk tolerance and financial objectives. Actively monitoring the risk exposure of a portfolio is vital, and calculating portfolio standard deviation provides the necessary metric to achieve this. It’s important for investors to not only calculate standard deviation of portfolio, but also to understand how to use it to make adjustments as needed. Using this metric empowers investors to make informed decisions about diversifying a portfolio and managing potential downside. A well diversified portfolio, often incorporating assets with low or negative correlations, can reduce the overall portfolio standard deviation. This strategic diversification offers a pathway to a more balanced and less volatile investment approach.
The dynamic nature of financial markets demands ongoing vigilance in portfolio risk assessment. It is not sufficient to calculate standard deviation of portfolio once and assume it will remain constant over time. Investors need to understand the impermanent nature of markets, where conditions shift, and new data emerges continuously. Recalculating standard deviation periodically is crucial, as asset returns and correlations can fluctuate substantially due to various market forces. This iterative process allows investors to effectively track and react to changes in portfolio risk, ensuring that investment strategies stay aligned with their risk tolerance and financial goals. Regular calculation of the standard deviation of a portfolio serves as a safeguard against unexpected market events, and enables investors to fine tune their investment strategy in response to new information and market evolution. Utilizing standard deviation for strategic decision-making enables a more proactive and informed approach to portfolio management. Calculating portfolio standard deviation is a tool that empowers investors to adapt to changing market conditions effectively.