Understanding Put Options: A Primer
A put option grants the buyer the right, but not the obligation, to sell an underlying asset at a specified price (the strike price) on or before a certain date (the expiration date). This contrasts with a call option, which provides the right to buy. Essentially, put options are often used by investors who believe the price of an asset will decrease. Imagine you own shares of a tech company, and you’re concerned about a potential market downturn. You could purchase a put option on those shares. If the share price falls below the strike price, the put option becomes profitable, allowing you to sell your shares at the higher strike price. The price you pay for this right is called the premium. The premium acts like an insurance payment, protecting you against potential losses. If the stock price goes up instead, the put option expires worthless, and your loss is limited to the premium. This illustrates both the risk and reward profiles. The investor could use the black scholes put option formula later to price these protections.
Put options are a powerful tool for both hedging and speculation. The interplay of the strike price, expiration date, and premium determines the option’s value and its potential profitability. For instance, a put option with a strike price close to the current price is considered ‘at the money’, while a strike price higher is ‘in the money’ and lower is ‘out of the money’. The time until expiration also significantly affects the price. As the expiration date approaches, the option value can change rapidly, reflecting the diminishing chance of it becoming profitable. The premium reflects these factors, acting as a critical consideration in the overall strategy. Understanding these core features is essential before delving into the intricacies of option valuation, including the black scholes put option formula. This formula offers a theoretical framework for pricing options and will be discussed in the following sections of this article.
The Black-Scholes Model: A Foundation for Option Pricing
The Black-Scholes model stands as a cornerstone in the world of finance, providing a theoretical framework for valuing options contracts. This groundbreaking model, developed by Fischer Black and Myron Scholes, revolutionized the way investors perceive and trade options. It’s essential to understand that the black scholes put option formula isn’t merely a mathematical equation; it represents a fundamental shift in how option pricing is approached. At its core, the model operates under several key assumptions, including the notion that markets are efficient, price movements follow a log-normal distribution, and volatility remains constant. While these assumptions don’t always perfectly align with real-world market conditions, the Black-Scholes model has proven to be an indispensable tool, widely utilized for its clarity and simplicity. Its historical significance is undeniable; before its arrival, options pricing was largely based on intuition and experience rather than rigorous mathematical analysis. The model allowed for a more standardized and quantifiable method of assessing option values, fostering greater transparency and efficiency in the options market. Its widespread adoption by traders, investors, and financial institutions underlines its fundamental importance. Despite the fact that modern finance has developed a range of more sophisticated models, the black scholes put option formula continues to serve as a key benchmark.
It is important to consider that the Black-Scholes model should not be approached as a definitive answer, but rather a valuable theoretical framework. The assumption of constant volatility, for example, is a primary limitation because in the real world volatility fluctuates depending on numerous market influences. Similarly, the model assumes no transaction costs or dividends are involved, neither of which is generally true in practical trading. Furthermore, the model is best suited for European-style options, which can only be exercised at expiration, and not for American-style options, which can be exercised at any point before expiry. The beauty of the Black-Scholes framework lies in its ability to simplify complicated ideas into a manageable formula, providing a good initial approximation for the value of options. Understanding the assumptions, though limiting, is key to realizing the power and proper use of the black scholes put option formula. In essence, the model laid the groundwork for future developments in derivatives pricing and continues to be a vital component of financial education and practice. By grasping the fundamentals of the Black-Scholes model, one gains a greater insight into the dynamics of options trading and the factors that affect the prices of these instruments.
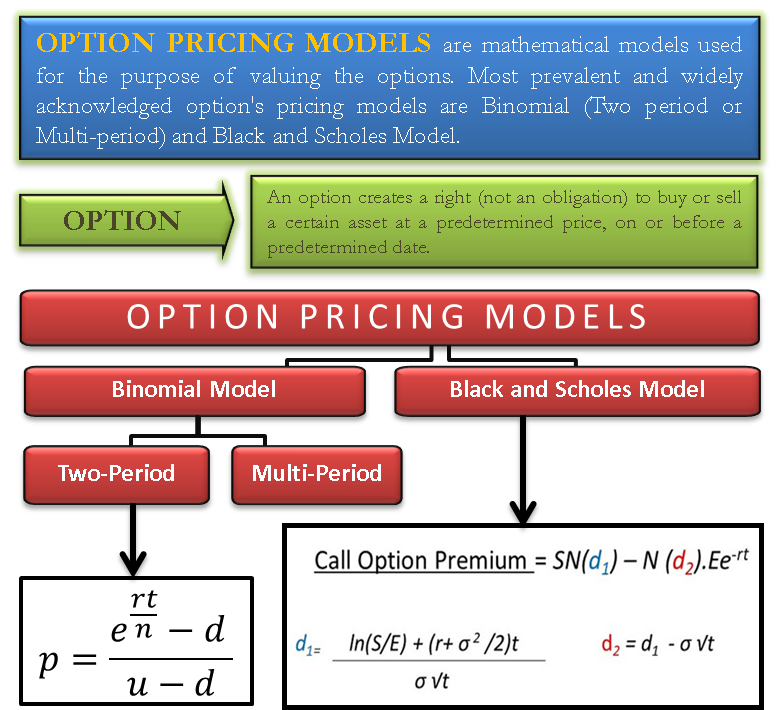
Dissecting the Black-Scholes Put Option Formula: Step-by-Step
The heart of understanding put option pricing lies within the black scholes put option formula. This formula, though appearing complex initially, is built upon several key variables that represent various market factors. The core formula is as follows: P = Ke-rTN(-d2) – S0N(-d1). Let’s break down each component. ‘P’ denotes the theoretical price of the put option. ‘K’ signifies the strike price, representing the price at which the underlying asset can be sold. ‘S0‘ stands for the current price of the underlying asset. ‘r’ is the risk-free interest rate, typically the yield on a government bond. ‘T’ represents the time to expiration, expressed in years. The exponential term ‘e-rT‘ is a mathematical constant that reflects the present value of a future cash flow. ‘N(x)’ is the cumulative standard normal distribution function of x, which essentially calculates probabilities. Finally, ‘d1‘ and ‘d2‘ are two intermediate calculations derived from other variables; where, d1 = (ln(S0/K) + (r + σ2/2)T) / (σ√T), and d2 = d1 – σ√T. Here, ‘σ’ represents the volatility of the underlying asset, often interpreted as the standard deviation of price changes.
To visually illustrate the significance of each component in the black scholes put option formula, consider a stock option diagram. The current stock price (S0) is the starting point, while the strike price (K) acts as a reference price. The time to expiration (T) is a time dimension influencing the overall value. Higher volatility (σ), depicted with wider price fluctuation, increases the chance of the put option expiring in-the-money, thus increasing its value. Similarly, a higher risk-free interest rate (r) generally increases the value of put options due to discounted values. Each variable influences the value of the put option differently. A higher strike price or greater volatility usually increases the price of the put option. Conversely, a higher price of the underlying asset or less time until expiry reduces the value of the put option. By understanding how each input interacts within the black scholes put option formula, one gains the ability to predict a put option’s theoretical value.
How to Calculate Put Option Value Using the Black-Scholes Formula
This section provides a practical guide on applying the black scholes put option formula to determine the theoretical price of a put option. To begin, it’s essential to gather the necessary inputs: the current stock price (S), the strike price of the option (K), the time to expiration (T) expressed in years, the risk-free interest rate (r), and the volatility of the stock price (σ). Time to expiration is commonly calculated as the number of days remaining until expiration divided by 365. For illustrative purposes, let’s assume we have a stock currently trading at $50 (S=50). We are interested in a put option with a strike price of $55 (K=55) that expires in 90 days (T=90/365), with an annual risk-free interest rate of 5% (r=0.05), and an estimated annual volatility of 20% (σ=0.20). Once these values are established, they are inserted into the Black-Scholes formula, which also relies on the cumulative standard normal distribution function, denoted by N(x), which can be computed with statistical tools or spreadsheets.
The Black-Scholes put option formula is expressed as: P = K * e^(-rT) * N(-d2) – S * N(-d1). Where d1 and d2 are intermediary variables, calculated as: d1 = [ln(S/K) + (r + σ²/2) * T] / (σ * sqrt(T)) and d2 = d1 – σ * sqrt(T). Using our example values, we first calculate d1 and d2. Let’s calculate d1 as [ln(50/55) + (0.05 + 0.20²/2) * (90/365)] / (0.20 * sqrt(90/365)), which comes to approximately -0.368. Next, d2 is computed as d1 – 0.20 * sqrt(90/365), approximately -0.467. Next, the cumulative normal distribution, N(-d1) and N(-d2) must be found. Using a statistical table or software, we find N(-d1) or N(0.368) to be approximately 0.356 and N(-d2) or N(0.467) to be approximately 0.320. Now, we can plug these values into the Black-Scholes put option formula: P = 55 * e^(-0.05 * 90/365) * 0.320 – 50 * 0.356. The exponential function, e^(-0.05 * 90/365), is roughly 0.9877. Thus P = 55 * 0.9877 * 0.320 – 50 * 0.356, which is approximately 17.45 – 17.8, resulting in a theoretical put option price of approximately $ -0.35. However, the formula calculates the theoretical value, and due to the mathematical treatment of the variables, a negative price might come up in certain cases, which would practically mean a price of close to zero. The price is then said to be below its intrinsic value; a value of $2.73 results if the N values are reversed, in this instance, this would be considered an almost “in the money” put option.
Interpreting the Results: What the Black-Scholes Value Means
The value obtained from the black scholes put option formula represents the theoretical fair price of a put option, under the specific assumptions of the model. This calculated value is an estimate of what the option should be worth, based on factors like the current stock price, the option’s strike price, the time remaining until expiration, the risk-free interest rate, and the volatility of the underlying asset. For put option buyers, this value is a guide to determine if an option is potentially under or overvalued in the market. A market price significantly below the black scholes put option formula result might indicate a buying opportunity, while a price much higher could suggest it is overpriced. For sellers, this theoretical value helps gauge the potential risk and reward of writing put options. The black scholes put option formula is not a predictor of future prices, but a tool to understand the intrinsic and extrinsic value of an option at a specific point in time, as such, it is important to understand its utility as a theoretical model, not as a market predictor.
It is crucial to differentiate between the theoretical price given by the black scholes put option formula and the actual market price of an option. The theoretical value serves as a benchmark, and the market price is where buyers and sellers agree to transact. Discrepancies between these two values can arise from various market factors including supply and demand, investor sentiment, and trading activity. In a liquid market with sufficient trading volumes, the market price usually hovers around the theoretical price, as sophisticated traders and institutions use the black scholes put option formula as a baseline for making decisions. However, in less liquid markets, or when considering options far from their expiration date, the actual market price may diverge quite significantly, due to many other factors not present in the black scholes put option formula. Thus, while the model is invaluable for understanding option pricing, it is essential to be aware that market price often provides a more nuanced reflection of the option’s value in real time.
In essence, the black scholes put option formula’s result is a theoretical point of reference. It assists traders in deciding how to execute a trade. If the market price is lower than the formula’s result, some buyers will find the price interesting; but, it doesn’t mean they will buy the option. Similarly, if the market price is higher than the formula’s result, sellers will see that as an opportunity to sell. Thus, the theoretical value provided by the black scholes put option formula is only one element among many that market participants must consider when making decisions about buying or selling put options. It is important to remember that the model does not account for every real world element that may influence an option’s price, and therefore, should not be the sole element in a trader’s or investor’s strategy.
Limitations and Considerations of the Black-Scholes Model
The Black-Scholes model, while a cornerstone for understanding the theoretical value of a put option, operates under several assumptions that may not perfectly align with the realities of the market. One of its most significant limitations is the assumption of constant volatility. In the real world, volatility, which is a measure of how much a stock’s price fluctuates, is far from constant; it can change rapidly and dramatically due to market events, news, and other factors. This means that a black scholes put option formula calculation, based on a static volatility figure, may not accurately reflect the true price of the put option, especially when applied to longer-term options or in volatile markets. Another limitation lies in the assumption of a frictionless market, where transaction costs and taxes are ignored. These real-world factors can impact the final profitability of a put option strategy, and the simple black scholes put option formula does not account for them. The model also assumes that stock prices follow a log-normal distribution, which is an idealization of how prices behave; in reality, stock price movements can exhibit what are known as “fat tails,” meaning there are more extreme events than the log-normal model would predict, thus potentially underestimating the risk of large price swings. Finally, the black scholes put option formula assumes a constant risk-free interest rate over the option’s life, which is unlikely given changes in economic conditions and central bank policies.
Furthermore, the black scholes put option formula assumes that there are no dividend payments on the underlying asset during the life of the option. Dividends can significantly affect the price of the underlying stock and, consequently, the put option’s value. The model’s limitation concerning volatility is perhaps the most discussed, leading to the development of alternative models that incorporate stochastic or fluctuating volatility. These alternative models, which are more complex, attempt to address the shortcomings of the Black-Scholes model. Despite its limitations, the Black-Scholes model remains a valuable tool for understanding the fundamental factors that influence option prices and a starting point for more complex analysis. By recognizing that the black scholes put option formula provides a theoretical price, not a guaranteed market price, users can manage their expectations more realistically and make informed trading decisions. The theoretical valuation generated by the formula should be used as a component in a larger framework of analysis that includes real-world observation, market sentiment, and other pricing influences, and not as the single determining factor of an options value.
Practical Applications of Black-Scholes Put Option Pricing
Understanding the practical applications of the Black-Scholes put option formula extends far beyond theoretical exercises, playing a crucial role in various financial strategies. One prominent area is in hedging, where investors utilize put options to protect their portfolios from potential downturns. For instance, an investor holding a substantial stock position might purchase put options on the same stock, thus creating a safety net. If the stock price falls, the profits from the put options can help offset the losses in the stock portfolio, effectively limiting downside risk. The Black-Scholes formula assists in determining the appropriate price for these hedging put options, ensuring that the insurance is neither too expensive nor insufficient to provide the necessary protection. This allows investors to use the black scholes put option formula to confidently implement risk management techniques, creating more resilient investment portfolios. Another important application is in trading strategies, where traders use put options to speculate on price declines. For example, if a trader believes a stock price will decrease, purchasing put options is an effective method for profiting from that belief without holding the stock. The accuracy of the black scholes put option formula is a major component of building such trading decisions. The Black-Scholes model allows traders to assess the theoretical value of these put options, aiding in their decisions about whether to buy or sell.
Furthermore, the application of the black scholes put option formula is essential for risk management by institutions and investment firms. They frequently manage large portfolios with multiple positions, and put options are used to protect and minimize losses in their financial operations. This involves complex strategies, where the Black-Scholes model helps in establishing fair prices for put options, thus allowing financial institutions to manage their risk exposures. These institutions do not just use options for hedging, but sometimes they also use them as part of more complex investment structures, where understanding the value of the put option via the Black-Scholes is a key element in determining the value of the whole investment and how it will change under different market scenarios. In addition, the black scholes put option formula is often incorporated into sophisticated financial models used for portfolio construction and stress testing. By calculating the theoretical price of put options, portfolio managers can better assess the potential impact of market volatility on their positions, thereby improving their risk-adjusted returns. The consistent use of the black scholes put option formula in these various practical applications demonstrates its pivotal role in financial decision-making, investment planning, and risk mitigation across the financial landscape.
Advanced Concepts and Further Exploration
Beyond the foundational understanding provided by the black scholes put option formula, several advanced concepts offer a deeper insight into option pricing. Implied volatility, a crucial metric derived from market prices, provides a more realistic measure of volatility than historical data used in the basic Black-Scholes model. Understanding implied volatility is essential for accurately pricing options and recognizing market sentiment. The black scholes put option formula’s output is significantly influenced by implied volatility; a higher implied volatility generally leads to a higher put option price.
The “Greeks”—Delta, Gamma, Theta, Vega, and Rho—represent the sensitivity of an option’s price to changes in various underlying factors. Delta measures the rate of change of the option price with respect to changes in the underlying asset’s price. Gamma quantifies the rate of change of Delta. Theta reflects the time decay of the option’s value as the expiration date approaches. Vega shows the sensitivity to changes in volatility, while Rho measures the sensitivity to changes in the risk-free interest rate. Mastering these Greeks is crucial for sophisticated option trading strategies and risk management. Accurate calculation of these Greeks often relies on extensions and refinements of the basic black scholes put option formula.
For those seeking a more nuanced understanding beyond the continuous-time framework of the Black-Scholes model, binomial trees offer a discrete-time alternative. Binomial trees model the price movements of the underlying asset over a series of time steps, allowing for a more granular analysis of option pricing. While more complex computationally, binomial trees can handle situations where the assumptions of the black scholes put option formula, such as constant volatility, are less realistic. Understanding these advanced concepts enhances the practical application and interpretation of the black scholes put option formula, making it a more powerful tool in the arsenal of option traders and investors.