Understanding the Concept of Duration in Bond Analysis
In the world of bond investing, duration is a critical concept that measures the sensitivity of bond prices to changes in interest rates. It provides investors with a valuable tool to gauge the potential impact of interest rate fluctuations on their bond portfolios. When interest rates rise, bond prices typically fall, and vice versa. By grasping the concept of duration, investors can better navigate the complexities of bond markets and make more informed decisions. In essence, duration helps investors understand how much the value of their bond portfolio will change in response to changes in interest rates. This is particularly important for investors seeking to minimize risk and maximize returns in their bond portfolios. As we delve deeper into the world of bond analysis, it’s essential to understand the concept of Macaulay duration, a specific type of duration that plays a vital role in bond investing.
What is Macaulay Duration: A Comprehensive Definition
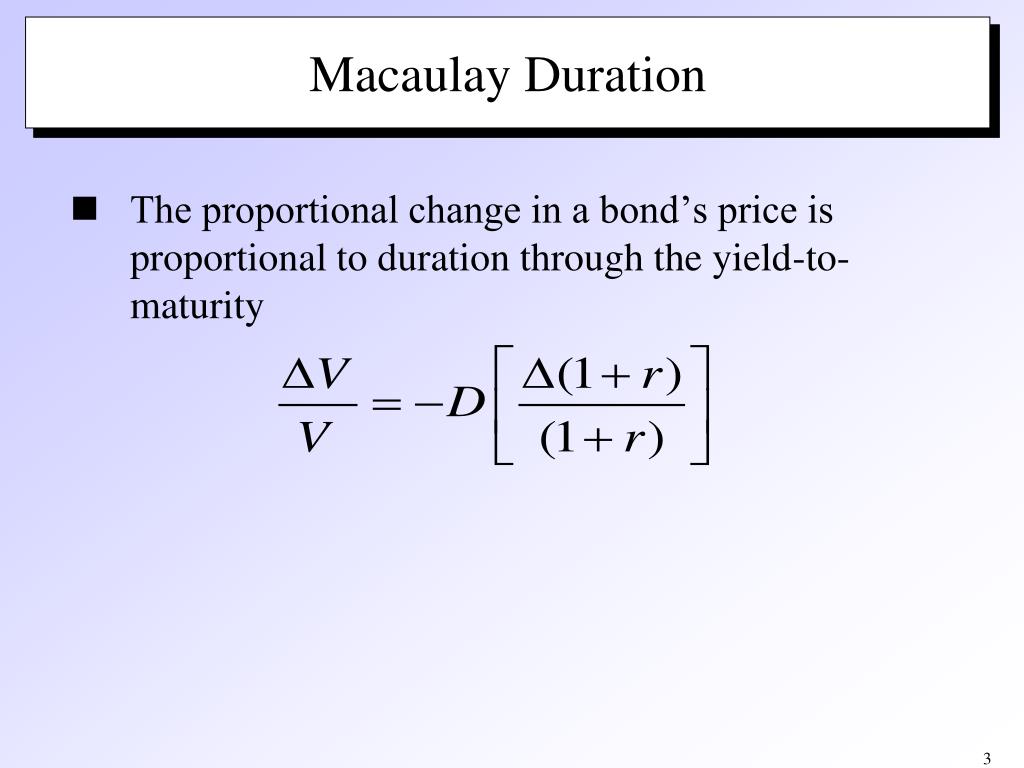
Macaulay duration, a concept developed by Frederick Macaulay, is a measure of the sensitivity of a bond’s price to changes in interest rates. It is a crucial metric in bond analysis, as it helps investors understand the potential impact of interest rate fluctuations on their bond portfolios. In essence, Macaulay duration measures the weighted average of the times until a bond’s cash flows are received, with the weights being the present value of each cash flow. This metric is expressed in years and provides a snapshot of a bond’s sensitivity to interest rate changes. For instance, a bond with a Macaulay duration of 5 years means that for every 1% change in interest rates, the bond’s price will change by approximately 5%. Understanding what is Macaulay duration of a bond is vital for investors seeking to manage risk and optimize returns in their bond portfolios.
How to Calculate Macaulay Duration: A Step-by-Step Guide
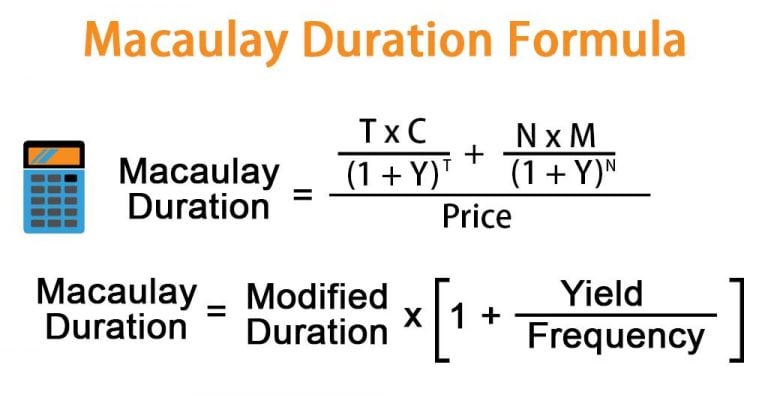
Calculating Macaulay duration is a crucial step in bond analysis, as it provides investors with a valuable tool to measure the sensitivity of bond prices to changes in interest rates. The formula to calculate Macaulay duration is as follows:
Macaulay Duration = Σ (t x PVCF) / Bond Price
Where:
- t = time until cash flow is received
- PVCF = present value of cash flow
- Bond Price = current market price of the bond
To illustrate this concept, let’s consider an example. Suppose we have a 10-year bond with a face value of $1,000, a coupon rate of 5%, and a yield to maturity of 4%. Using the formula above, we can calculate the Macaulay duration as follows:
Step 1: Calculate the present value of each cash flow using the yield to maturity.
Step 2: Multiply each present value by the time until the cash flow is received.
Step 3: Sum the products from Step 2.
Step 4: Divide the sum from Step 3 by the current market price of the bond.
The resulting Macaulay duration would be approximately 7.5 years, indicating that for every 1% change in interest rates, the bond’s price would change by approximately 7.5%. Accurate calculations are essential in bond analysis, as small errors can lead to significant differences in Macaulay duration and, ultimately, investment decisions. By mastering the calculation of Macaulay duration, investors can gain a deeper understanding of what is Macaulay duration of a bond and make more informed decisions in their bond portfolios.
The Role of Macaulay Duration in Bond Portfolio Management
In bond portfolio management, Macaulay duration plays a vital role in measuring the sensitivity of a bond portfolio to changes in interest rates. By understanding the Macaulay duration of a bond, investors can better manage risk and make informed investment decisions. A bond with a higher Macaulay duration is more sensitive to interest rate changes, while a bond with a lower Macaulay duration is less sensitive.
In a bond portfolio, Macaulay duration can be used to:
- Manage interest rate risk: By adjusting the Macaulay duration of a bond portfolio, investors can mitigate the impact of interest rate changes on their investments.
- Optimize returns: Macaulay duration can help investors identify bonds with higher returns and lower risk, enabling them to optimize their portfolio’s performance.
- Improve diversification: By combining bonds with different Macaulay durations, investors can create a diversified portfolio that is less sensitive to interest rate changes.
For instance, an investor with a bond portfolio consisting of long-term bonds with high Macaulay durations may want to consider diversifying their portfolio by adding short-term bonds with lower Macaulay durations. This would help reduce the overall sensitivity of the portfolio to interest rate changes, thereby minimizing potential losses.
Understanding what is Macaulay duration of a bond is crucial in bond portfolio management, as it enables investors to make informed decisions about their investments and optimize their returns. By incorporating Macaulay duration into their investment strategy, investors can better navigate the complexities of the bond market and achieve their investment goals.
Interpreting Macaulay Duration: What Does it Mean for Investors?
Once calculated, Macaulay duration provides valuable insights into the behavior of a bond in response to changes in interest rates. Understanding what is Macaulay duration of a bond is crucial for investors to make informed decisions about their investments. Here’s how to interpret Macaulay duration:
A higher Macaulay duration indicates that a bond is more sensitive to interest rate changes. For example, a bond with a Macaulay duration of 10 years will experience a greater price change than a bond with a Macaulay duration of 5 years, given the same change in interest rates.
Conversely, a lower Macaulay duration suggests that a bond is less sensitive to interest rate changes. This makes it a more attractive option for investors seeking to minimize interest rate risk.
Macaulay duration also has implications for bond yields and returns. A bond with a higher Macaulay duration will typically offer a higher yield to compensate investors for taking on greater interest rate risk. Conversely, a bond with a lower Macaulay duration will offer a lower yield.
For investors, understanding Macaulay duration is essential for making informed decisions about their bond investments. By considering the Macaulay duration of a bond, investors can:
- Assess interest rate risk and adjust their portfolio accordingly
- Identify bonds with higher yields and lower risk
- Optimize their portfolio’s performance by combining bonds with different Macaulay durations
By grasping the concept of Macaulay duration and its implications for bond prices, yields, and returns, investors can make more informed decisions and achieve their investment goals.
Macaulay Duration vs. Modified Duration: What’s the Difference?
In bond analysis, two commonly used measures of duration are Macaulay duration and modified duration. While both measures are used to quantify the sensitivity of bond prices to changes in interest rates, they differ in their calculation and interpretation.
Macaulay duration, as discussed earlier, is a measure of the weighted average time to maturity of a bond’s cash flows. It takes into account the present value of each cash flow and the time until it is received. Macaulay duration is a more comprehensive measure of duration, as it considers the entire cash flow profile of a bond.
Modified duration, on the other hand, is a simplified measure of duration that assumes a flat yield curve. It is calculated as the percentage change in a bond’s price in response to a 1% change in interest rates. Modified duration is a more approximate measure of duration, as it does not consider the entire cash flow profile of a bond.
The key differences between Macaulay duration and modified duration are:
- Calculation: Macaulay duration is calculated using the present value of each cash flow, while modified duration is calculated using a simplified formula.
- Accuracy: Macaulay duration is a more accurate measure of duration, as it considers the entire cash flow profile of a bond. Modified duration is an approximation that assumes a flat yield curve.
- Interpretation: Macaulay duration provides a more comprehensive understanding of a bond’s sensitivity to interest rate changes, while modified duration provides a simplified measure of duration.
When to use each measure:
- Macaulay duration is preferred when analyzing bonds with complex cash flow profiles or when seeking a more accurate measure of duration.
- Modified duration is suitable for quick estimates of duration or when analyzing bonds with simple cash flow profiles.
In conclusion, while both Macaulay duration and modified duration are used to measure the sensitivity of bond prices to changes in interest rates, they differ in their calculation and interpretation. Understanding the differences between these two measures is essential for bond investors seeking to make informed decisions about their investments.
Real-World Applications of Macaulay Duration in Bond Analysis
In the world of bond investing, Macaulay duration plays a crucial role in informing investment decisions and managing risk. Here are some real-world examples of how Macaulay duration is used in bond analysis:
Case Study 1: Portfolio Optimization
A pension fund manager is tasked with optimizing a bond portfolio to minimize interest rate risk. By calculating the Macaulay duration of each bond in the portfolio, the manager can identify the bonds that are most sensitive to interest rate changes. The manager can then adjust the portfolio’s composition to reduce its overall Macaulay duration, thereby minimizing interest rate risk.
Industry Application 1: Bond Indexing
Bond index funds use Macaulay duration to track the performance of a bond market index. By calculating the Macaulay duration of the bonds in the index, the fund manager can create a portfolio that mirrors the index’s duration profile. This ensures that the fund’s performance is closely aligned with the index’s performance.
Case Study 2: Risk Management
A corporate treasurer is responsible for managing the interest rate risk of a company’s bond portfolio. By calculating the Macaulay duration of each bond, the treasurer can identify the bonds that are most exposed to interest rate changes. The treasurer can then use this information to hedge against interest rate risk, thereby protecting the company’s financial position.
Industry Application 2: Asset Liability Management
Insurance companies use Macaulay duration to manage the asset-liability mismatch of their bond portfolios. By calculating the Macaulay duration of their assets (bonds) and liabilities (insurance policies), the insurer can ensure that the duration of their assets matches the duration of their liabilities. This helps to minimize interest rate risk and ensure that the insurer can meet its future obligations.
These examples demonstrate the importance of Macaulay duration in bond analysis. By understanding what is Macaulay duration of a bond, investors and portfolio managers can make informed decisions about their investments and manage risk more effectively.
Conclusion: Mastering Macaulay Duration for Bond Investing Success
In conclusion, Macaulay duration is a powerful tool in bond investing, providing investors with a comprehensive measure of a bond’s sensitivity to interest rate changes. By understanding what is Macaulay duration of a bond, investors can make informed decisions about their investments, manage risk more effectively, and optimize their bond portfolios.
The significance of Macaulay duration lies in its ability to quantify the impact of interest rate changes on bond prices, yields, and returns. By calculating Macaulay duration, investors can identify bonds that are most sensitive to interest rate changes and adjust their portfolios accordingly.
In bond portfolio management, Macaulay duration plays a crucial role in risk management and investment decisions. By understanding the Macaulay duration of their bond portfolios, investors can minimize interest rate risk, maximize returns, and achieve their investment objectives.
As demonstrated through real-world examples and case studies, Macaulay duration is a widely used and effective tool in bond analysis. Its applications extend beyond individual bond investing to portfolio management, asset liability management, and risk management.
In today’s complex bond market, mastering Macaulay duration is essential for bond investing success. By grasping the concepts and applications of Macaulay duration, investors can navigate the bond market with confidence, make informed decisions, and achieve their investment goals.