What is Beta in CAPM and Why is it Important?
In the Capital Asset Pricing Model (CAPM), beta is a crucial component that measures the systematic risk of an investment. It is a numerical value that indicates the volatility of an investment relative to the overall market. A beta of 1 indicates that the investment moves in tandem with the market, while a beta greater than 1 suggests higher volatility.
The significance of beta lies in its ability to help investors and portfolio managers gauge the potential risks and returns of an investment. By understanding beta, investors can make informed decisions about their investment portfolios, balancing risk and potential returns. In essence, beta is a key factor in determining the expected return on an investment, making it a critical component of the investment decision-making process.
As investors seek to optimize their portfolios, they often ask themselves, “how to calculate beta for capm?” Understanding the beta calculation process is essential for accurate risk assessment and informed investment decisions. In this article, we will delve into the world of beta calculation, exploring the formula, data requirements, and step-by-step examples to help you master this critical skill.
Understanding the Formula: Breaking Down the Beta Calculation
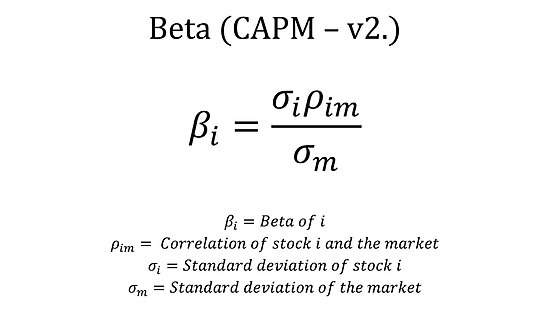
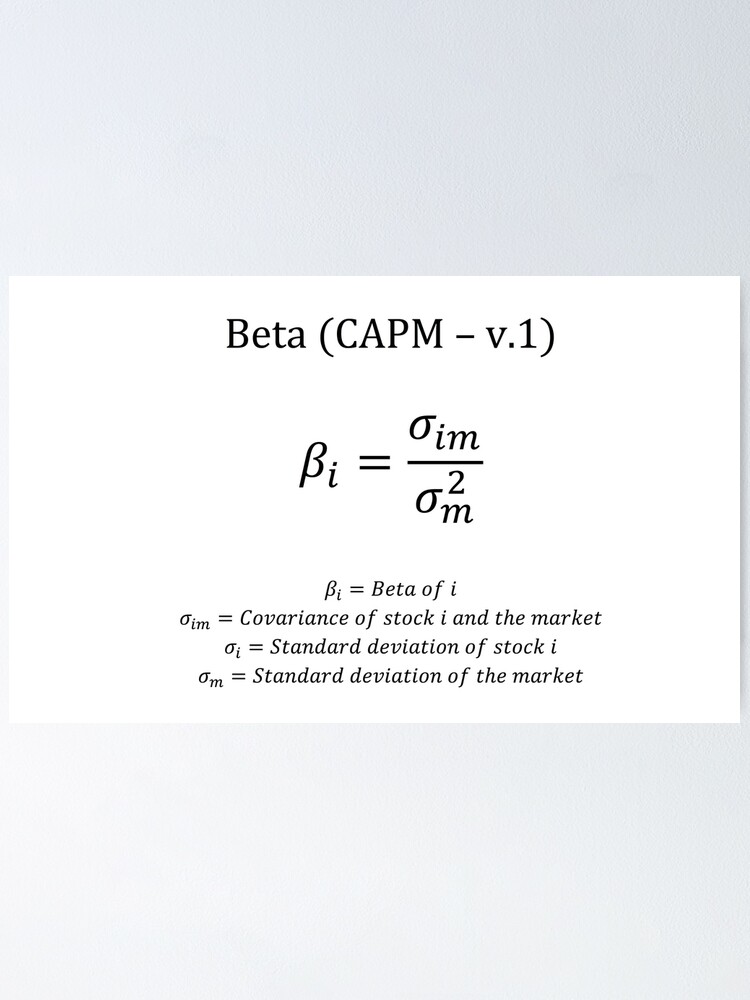
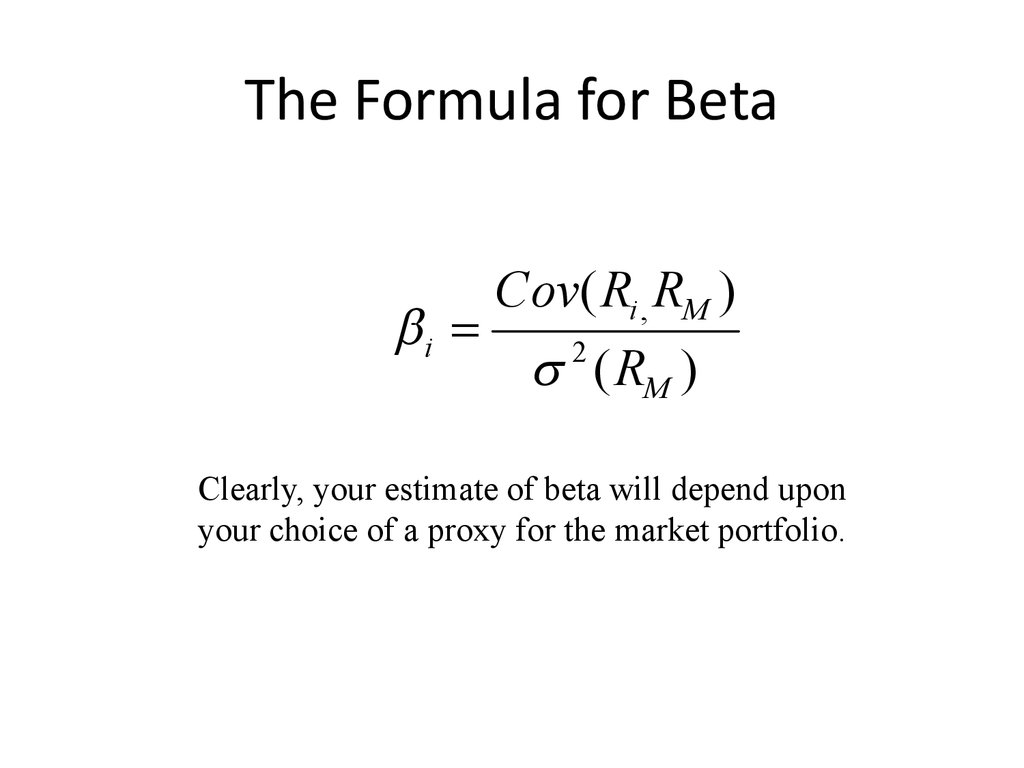
The beta calculation formula is a fundamental component of the Capital Asset Pricing Model (CAPM). It is a mathematical expression that helps investors quantify the systematic risk of an investment. The formula is as follows:
β = Covariance (Ri, Rm) / Variance (Rm)
Where:
- β = Beta of the investment
- Ri = Return of the investment
- Rm = Return of the market
- Covariance (Ri, Rm) = Covariance between the investment return and market return
- Variance (Rm) = Variance of the market return
This formula may seem complex, but it’s essential to understand the variables involved and their significance. The covariance between the investment return and market return measures the degree to which the investment moves in tandem with the market. The variance of the market return, on the other hand, represents the overall market risk.
To illustrate this concept, let’s consider a simple example. Suppose we want to calculate the beta of a stock using historical data. We can use a spreadsheet to calculate the covariance and variance, and then plug in the values to get the beta. For instance, if the covariance is 0.05 and the variance is 0.01, the beta would be 5. This means that for every 1% move in the market, the stock is expected to move 5%.
Understanding the beta calculation formula is crucial for investors who want to learn how to calculate beta for capm. By grasping this concept, investors can make more informed decisions about their investment portfolios and better manage risk.
Gathering Data: What You Need to Calculate Beta
To calculate beta, investors need to gather specific data that will help them estimate the systematic risk of an investment. The required data includes historical stock prices, market returns, and risk-free rates. These data points are essential in understanding how the investment moves in relation to the overall market.
Historical stock prices are necessary to calculate the returns of the investment. This data can be obtained from financial databases, such as Yahoo Finance or Quandl, or from the investment’s website. It’s essential to have a sufficient amount of data, typically 2-5 years, to ensure accurate calculations.
Market returns are also crucial in beta calculation. This data represents the overall market performance and can be obtained from market indices, such as the S&P 500. The market return data should be aligned with the historical stock prices to ensure accurate calculations.
Risk-free rates, such as the yield on U.S. Treasury bonds, are used to estimate the return of a risk-free investment. This data can be obtained from government websites or financial databases.
The quality of the data is critical in beta calculation. Investors should ensure that the data is accurate, complete, and consistent. Any errors or inconsistencies in the data can lead to inaccurate beta calculations, which can result in poor investment decisions.
Understanding how to gather the necessary data is a critical step in learning how to calculate beta for capm. By having access to accurate and reliable data, investors can make informed decisions about their investment portfolios and better manage risk.
Calculating Beta: A Step-by-Step Example
To illustrate the beta calculation process, let’s use a real-world example. Suppose we want to calculate the beta of Apple Inc. (AAPL) using historical data. We’ll use a 3-year period, from 2018 to 2020, to calculate the beta.
Step 1: Gather Historical Data
We’ll need the historical stock prices of AAPL and the market returns of the S&P 500 index. We can obtain this data from financial databases such as Yahoo Finance or Quandl.
Step 2: Calculate Returns
Using the historical data, we’ll calculate the returns of AAPL and the S&P 500 index. We can do this by subtracting the previous day’s closing price from the current day’s closing price and dividing by the previous day’s closing price.
Step 3: Calculate Covariance and Variance
Next, we’ll calculate the covariance between the returns of AAPL and the S&P 500 index. We can do this using a spreadsheet or statistical software. The covariance represents the degree to which the returns of AAPL move in tandem with the market returns.
Step 4: Calculate Beta
Finally, we’ll calculate the beta of AAPL using the formula: β = Covariance (Ri, Rm) / Variance (Rm). Plugging in the values, we get a beta of 1.15.
Interpretation
The beta of 1.15 indicates that AAPL is slightly more volatile than the overall market. For every 1% move in the market, AAPL is expected to move 1.15%. This information is crucial for investors and portfolio managers who want to understand the systematic risk of AAPL and make informed investment decisions.
By following these steps, investors can learn how to calculate beta for capm and gain a better understanding of the systematic risk of their investments. Remember to use accurate and reliable data to ensure accurate beta calculations.
Interpreting Beta: What the Results Mean for Investors
Once the beta calculation is complete, investors need to interpret the results to understand the implications for their investment decisions. The beta value provides insight into the systematic risk of an investment, which is essential for portfolio management and risk assessment.
A beta value of 1 indicates that the investment moves in line with the market, meaning it carries average systematic risk. A beta value greater than 1 indicates higher systematic risk, while a beta value less than 1 indicates lower systematic risk.
High-beta investments, such as those in the technology sector, are more volatile and tend to amplify market movements. These investments are suitable for aggressive investors who are willing to take on more risk in pursuit of higher returns.
Low-beta investments, such as those in the consumer goods sector, are less volatile and tend to reduce market movements. These investments are suitable for conservative investors who prioritize capital preservation over growth.
Understanding the beta value is crucial for investors and portfolio managers who want to construct an optimal portfolio that balances risk and return. By combining high-beta and low-beta investments, investors can create a diversified portfolio that minimizes risk and maximizes returns.
When learning how to calculate beta for capm, it’s essential to understand the interpretation of the results. By doing so, investors can make informed decisions about their investments and create a portfolio that aligns with their risk tolerance and investment objectives.
Common Pitfalls and Challenges in Beta Calculation
When learning how to calculate beta for capm, it’s essential to be aware of common pitfalls and challenges that can affect the accuracy of the results. By understanding these potential issues, investors can take steps to overcome them and ensure reliable beta calculations.
Data Quality Issues
One of the most common challenges in beta calculation is data quality. Historical stock prices, market returns, and risk-free rates must be accurate and reliable to produce a reliable beta value. Investors should ensure that their data sources are credible and up-to-date to avoid errors in the calculation process.
Time Period Selection
The choice of time period for beta calculation can also impact the results. A shorter time period may not capture the full range of market fluctuations, while a longer time period may be influenced by outdated data. Investors should select a time period that balances these considerations and provides a representative beta value.
Model Assumptions
The CAPM model relies on certain assumptions, such as the assumption of a normal distribution of returns. However, in reality, returns may not always follow a normal distribution, which can affect the accuracy of the beta calculation. Investors should be aware of these assumptions and consider alternative models or approaches when necessary.
Tips for Overcoming Challenges
To overcome these challenges, investors can take several steps. First, they should ensure that their data is accurate and reliable by using credible sources and updating their data regularly. Second, they should select a time period that balances the need for representative data with the need for timely information. Finally, they should be aware of the assumptions underlying the CAPM model and consider alternative approaches when necessary.
By understanding these common pitfalls and challenges, investors can improve the accuracy of their beta calculations and make more informed investment decisions. Remember, learning how to calculate beta for capm is an essential skill for any investor or portfolio manager, and being aware of these challenges is crucial for success.
Advanced Beta Calculation Techniques: Leveraging Regression Analysis
In addition to the traditional beta calculation method, investors can use advanced techniques to estimate beta, including regression analysis. This approach provides a more nuanced understanding of the relationship between the investment and the market, allowing for more accurate beta calculations.
Regression Analysis
Regression analysis is a statistical technique that examines the relationship between two or more variables. In the context of beta calculation, regression analysis can be used to estimate the beta coefficient by analyzing the historical relationship between the investment’s returns and the market’s returns.
The benefits of using regression analysis for beta calculation include:
- Improved accuracy: Regression analysis can provide a more precise estimate of beta by accounting for the nuances of the investment’s returns.
- Increased flexibility: Regression analysis allows investors to incorporate additional variables, such as macroeconomic factors, into the beta calculation.
- Enhanced understanding: Regression analysis provides insights into the underlying drivers of the investment’s returns, enabling investors to make more informed decisions.
However, regression analysis also has limitations, including:
- Complexity: Regression analysis requires a higher level of statistical expertise and data analysis skills.
- Data requirements: Regression analysis requires a large dataset of historical returns, which may not be available for all investments.
- Model assumptions: Regression analysis relies on certain assumptions, such as linearity and homoscedasticity, which may not always hold true.
By leveraging regression analysis, investors can gain a more sophisticated understanding of the investment’s systematic risk and make more informed decisions. When learning how to calculate beta for capm, it’s essential to consider these advanced techniques to improve the accuracy and reliability of beta calculations.
Conclusion: Mastering Beta Calculation for Informed Investment Decisions
In conclusion, understanding how to calculate beta for capm is a crucial skill for investors and portfolio managers. By grasping the concept of beta and its role in measuring systematic risk, investors can make more informed decisions and optimize their portfolios. Throughout this guide, we have walked through the step-by-step process of calculating beta, from understanding the formula to interpreting the results. We have also explored advanced techniques, such as regression analysis, to improve the accuracy of beta calculations.
By mastering the art of beta calculation, investors can gain a competitive edge in the market. Whether you are a seasoned investor or just starting out, learning how to calculate beta for capm is an essential skill that can help you navigate the complexities of the financial markets. Remember, accurate beta calculation is key to making informed investment decisions, and with practice and patience, you can unlock the power of CAPM to achieve your investment goals.