What are Macaulay and Modified Durations?
Macaulay and modified durations are essential measures of a bond’s sensitivity to interest rate changes. Imagine you’re buying a bond; these durations essentially tell you how long it will take to recover your initial investment, considering all the interest payments. Macaulay duration provides a weighted average time until the bond’s cash flows are received. Modified duration refines this measure by considering the bond’s yield to maturity (YTM), offering a more precise estimate of how a bond’s price will react to interest rate shifts. Understanding Macaulay and modified durations are crucial for effectively managing interest rate risk in any bond portfolio. They are powerful tools for investors seeking to understand and control the impact of fluctuating interest rates on their investments.
Think of it like this: a longer duration means a bond is more sensitive to interest rate changes. If interest rates rise, a bond with a long duration will likely fall more in price than a bond with a short duration. Conversely, if interest rates fall, a bond with a long duration will rise more in price. Macaulay and modified durations are, therefore, vital tools for investors to assess and manage the risks associated with their bond investments. The effective use of these metrics allows for better-informed decisions regarding portfolio construction and risk mitigation strategies. They provide a framework to predict how your bond investments might behave in response to shifts in the overall interest rate environment.
Macaulay and modified durations are not just theoretical concepts; they are practical tools used daily by bond portfolio managers and investors. By understanding these measures, investors can make more informed decisions about which bonds to include in their portfolio and how to adjust their holdings based on their interest rate risk tolerance. They are key components in the construction of well-diversified and effectively managed portfolios. Investors can use these tools to gauge the impact of interest rate changes and implement strategies to minimize potential losses and maximize returns. Understanding Macaulay and modified durations are therefore essential for navigating the complexities of the bond market.
Calculating Macaulay Duration: A Step-by-Step Guide
Macaulay duration measures the weighted average time until a bond’s cash flows are received. It essentially tells you the average time it takes to recoup your investment. Macaulay and modified durations are crucial for understanding a bond’s sensitivity to interest rate changes. The calculation considers the timing and size of each coupon payment and the final principal repayment. The time value of money is paramount; each cash flow is discounted back to its present value.
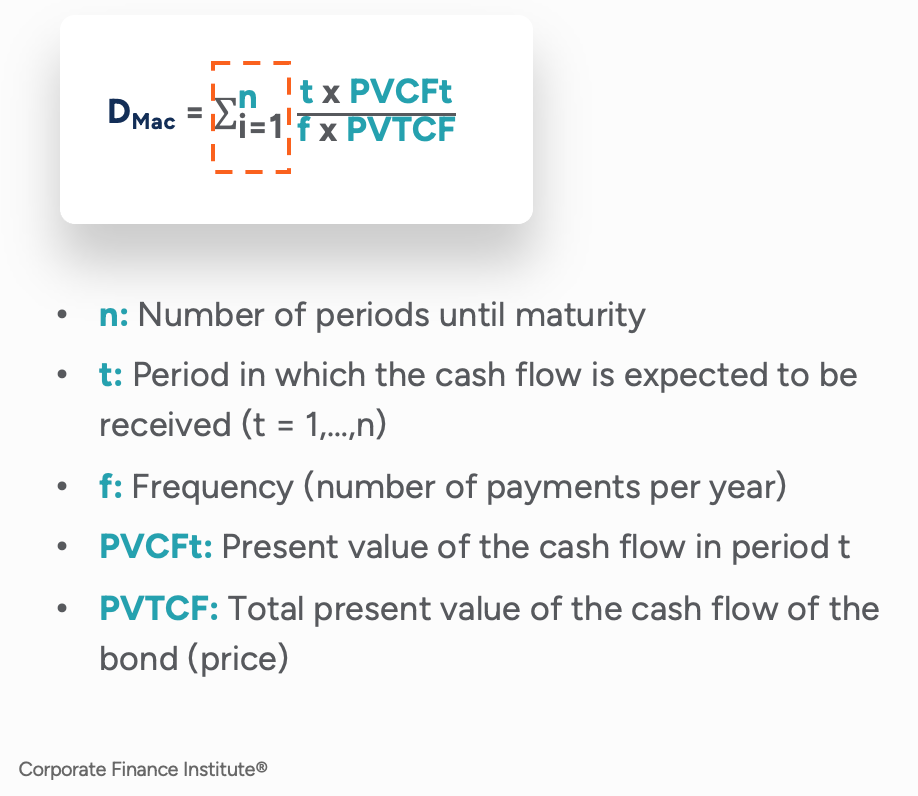
Let’s illustrate with a hypothetical 5-year bond with a 10% annual coupon rate and a face value of $1000. Assume a yield to maturity (YTM) of 8%. We’ll use the following formula: Macaulay Duration = Σ [t * PV(CFt) / Bond Price], where ‘t’ represents the time period, ‘PV(CFt)’ represents the present value of the cash flow at time t, and ‘Bond Price’ represents the present value of all future cash flows. A table will simplify this:
| Year (t) | Cash Flow (CFt) | PV(CFt) at 8% | t * PV(CFt) |
|---|---|---|---|
| 1 | $100 | $92.59 | $92.59 |
| 2 | $100 | $85.73 | $171.46 |
| 3 | $100 | $79.38 | $238.14 |
| 4 | $100 | $73.50 | $294.00 |
| 5 | $1100 | $735.03 | $3675.15 |
| Total | $1186.23 | $4471.34 |
Macaulay Duration = $4471.34 / $1186.23 ≈ 3.77 years. This indicates the average time until the investor receives their investment back. Remember, Macaulay and modified durations are essential tools for managing interest rate risk. Understanding the time value of money is crucial for accurate duration calculations. Each cash flow’s present value reflects its worth today. A higher YTM reduces present values and consequently, the duration.
Understanding Modified Duration: Its Relationship to Macaulay Duration
Modified duration builds upon Macaulay duration. It refines the measure by accounting for the yield to maturity (YTM). Macaulay duration provides a weighted average time to receive a bond’s cash flows. Modified duration, however, adjusts this average for the effects of compounding. This makes it a more practical tool for estimating bond price changes in response to interest rate fluctuations. The formula for modified duration is Macaulay duration divided by (1 + YTM).
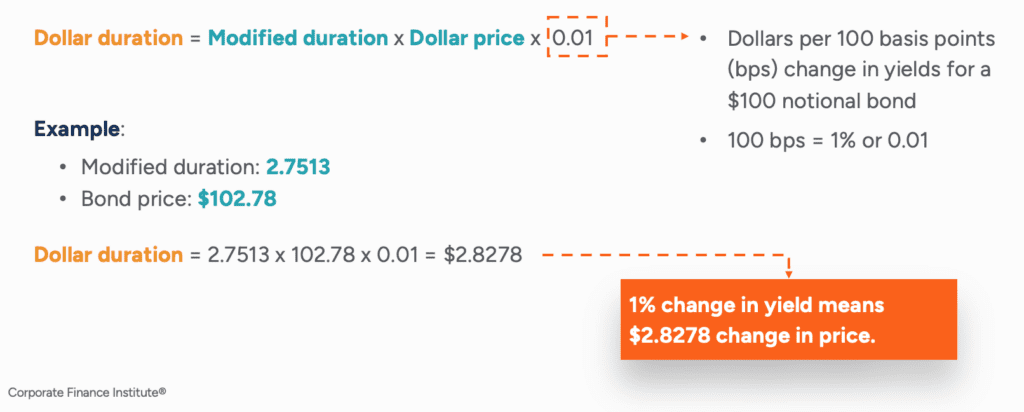
Macaulay and modified durations are closely related, yet distinct. Macaulay duration measures the average time until the bond’s cash flows are received. Modified duration, on the other hand, estimates the percentage change in a bond’s price for a 1% change in its YTM. This makes it particularly useful for assessing interest rate risk. For instance, a bond with a modified duration of 5 indicates that a 1% increase in YTM would lead to approximately a 5% decrease in its price. The choice between using Macaulay duration and modified duration depends on the specific application. Macaulay duration provides a useful measure of the overall timing of cash flows. Modified duration is more directly applicable to assessing price sensitivity to interest rate changes. Macaulay and modified durations are essential tools for managing interest rate risk.
The difference lies primarily in their application. Macaulay duration is a fundamental concept illustrating the weighted average time until cash flows are received. It’s useful for understanding the bond’s cash flow profile. Modified duration, however, is directly applicable to estimating price changes given interest rate movements. It offers a more practical approach for risk management. Investors utilize modified duration to gauge the sensitivity of their bond holdings to interest rate shifts. This enables them to make informed decisions, potentially hedging against potential losses due to rising interest rates. Macaulay and modified durations are valuable tools for portfolio managers striving to optimize bond portfolio performance.
How to Use Macaulay and Modified Durations to Manage Risk
Macaulay and modified durations are essential tools for managing interest rate risk in bond portfolios. Investors use Macaulay duration to estimate a bond’s average time to repayment. This provides insight into the bond’s sensitivity to interest rate changes. A higher Macaulay duration indicates greater interest rate sensitivity. Modified duration refines this measure by considering the yield to maturity, offering a more precise prediction of price changes due to interest rate fluctuations. Macaulay and modified durations are valuable because they help investors anticipate potential losses or gains from interest rate movements.
By understanding Macaulay and modified durations, investors can construct portfolios with desired levels of interest rate sensitivity. For example, a conservative investor seeking stability might favor bonds with low durations. Conversely, an investor seeking higher returns might accept greater risk by investing in bonds with longer durations. Macaulay and modified durations are particularly useful when anticipating interest rate hikes or cuts by central banks. Investors can use these metrics to adjust their portfolio allocations proactively, minimizing potential losses during periods of rising interest rates or maximizing gains when rates fall. The skillful application of these measures helps investors navigate the complexities of the bond market and make informed decisions.
Consider a scenario where interest rates are expected to rise. An investor holding a portfolio of bonds with high Macaulay and modified durations faces significant potential losses. This is because rising rates cause bond prices to fall, and longer-duration bonds experience larger price declines. Armed with duration analysis, the investor could adjust their holdings by selling some longer-duration bonds and purchasing shorter-duration ones, reducing the portfolio’s overall interest rate risk. Conversely, if interest rates are anticipated to fall, increasing the duration of a bond portfolio could enhance potential gains as bond prices rise. Macaulay and modified durations are, therefore, indispensable for effective bond portfolio construction and risk management. They are not a perfect predictor, but they provide valuable insights that improve investment outcomes.
The Limitations of Macaulay and Modified Duration
Macaulay and modified durations are valuable tools, but they rely on several key assumptions. A crucial assumption is a constant yield curve. In reality, the yield curve shifts and twists, impacting the accuracy of duration calculations. This means Macaulay and modified durations might over- or underestimate the true interest rate sensitivity of a bond, especially in volatile market conditions. Further, these measures assume parallel shifts in the yield curve. However, interest rate changes often affect different maturities differently, leading to non-parallel shifts. This divergence from the assumption again reduces the reliability of duration estimates. Macaulay and modified durations are most effective when used cautiously and with an awareness of their inherent limitations.
Another significant limitation arises from the assumption that cash flows remain constant. For bonds with embedded options like callable bonds or putable bonds, the actual cash flows can deviate from the initial projections. These options significantly alter the bond’s interest rate sensitivity, rendering duration measures less accurate. The simplified nature of Macaulay and modified durations also neglects the impact of factors like changes in credit spreads or liquidity risk. These factors significantly influence bond prices, but are not reflected in traditional duration calculations. Therefore, Macaulay and modified durations are best viewed as approximations rather than precise predictors of bond price movements.
Finally, Macaulay and modified durations are linear measures. They assume a linear relationship between bond prices and interest rates. However, this relationship is actually convex. This means that for large interest rate changes, the actual price change can deviate significantly from that predicted by duration. Ignoring convexity can lead to substantial errors, especially when managing large portfolios or anticipating significant interest rate shifts. Macaulay and modified durations are useful for approximating the interest rate sensitivity of bonds but should be applied carefully, considering their limitations and supplementing them with additional analysis when appropriate, especially for bonds with embedded options or during periods of high market volatility.
Duration and Convexity: A More Complete Picture
Macaulay and modified durations are valuable tools for assessing interest rate risk, but they have limitations. These measures assume a linear relationship between bond prices and interest rate changes. In reality, this relationship is often non-linear. This is where convexity comes in. Convexity measures the curvature of the relationship between bond prices and yields. A higher convexity indicates a greater degree of curvature, meaning that price changes are not perfectly proportional to yield changes. Macaulay and modified durations are, therefore, most accurate when interest rate changes are small and the yield curve shifts in a parallel manner. For larger changes, or non-parallel shifts, these estimations fall short.
Understanding convexity helps refine duration-based estimates of price changes. It accounts for the fact that the price increase from a decrease in yield is typically larger than the price decrease from an equivalent increase in yield. This asymmetry is captured by convexity. By incorporating convexity into the analysis, investors can obtain a more precise prediction of how a bond’s price will react to interest rate fluctuations. This is especially useful when dealing with large interest rate movements or non-parallel shifts in the yield curve. Macaulay and modified durations alone fail to capture this important nuance.
In essence, convexity acts as a refinement to duration. While Macaulay and modified durations provide a first-order approximation of price sensitivity, convexity provides a second-order approximation. Combining duration and convexity gives investors a much more comprehensive picture of a bond’s interest rate risk profile. This allows for more accurate risk management and potentially better investment decisions. Therefore, while Macaulay and modified durations are important tools, considering convexity improves the analysis significantly. This provides a more accurate assessment of a bond’s price sensitivity to interest rate changes, particularly for larger interest rate movements or non-parallel yield curve shifts.
Comparing Macaulay and Modified Duration: Which One to Use?
Macaulay and modified durations are both crucial measures of a bond’s interest rate sensitivity, but they serve different purposes. Macaulay duration, calculated as the weighted average time until a bond’s cash flows are received, provides a measure of a bond’s average maturity. It’s useful for understanding the overall timing of cash flows. However, Macaulay and modified durations are not directly comparable in terms of sensitivity to interest rate changes because Macaulay duration is expressed in years while modified duration measures percentage changes in price. Modified duration, on the other hand, accounts for the yield to maturity (YTM) and provides a more precise estimate of a bond’s price change in response to a 1% change in interest rates. Therefore, modified duration is generally preferred for assessing interest rate risk and managing bond portfolios.
The choice between Macaulay and modified duration depends on the specific application. Macaulay duration is useful for comparing bonds with similar YTMs, or for getting a general sense of the average time until the investor receives their investment back. Modified duration is essential when managing a portfolio where interest rate risk is a primary concern. Investors use modified duration to estimate the potential price changes of their bond holdings due to shifts in interest rates. Macaulay and modified durations are valuable tools, and understanding their differences is critical for effective bond portfolio management. For example, a bond with a high Macaulay duration will be more sensitive to interest rate changes than a bond with a low Macaulay duration. This translates into a higher modified duration and increased price volatility.
In summary, Macaulay duration provides a fundamental understanding of a bond’s cash flow timing, while modified duration offers a more practical measure of interest rate risk. Macaulay and modified durations are complementary metrics. While modified duration is typically favored for assessing interest rate risk, understanding Macaulay duration provides valuable context and insight into the bond’s overall structure and cash flows. The best approach often involves using both durations together to gain a complete picture of the bond’s characteristics and potential risks.
Real-World Examples of Macaulay and Modified Duration in Action
During the 2008 financial crisis, many investors relied heavily on Macaulay and modified durations are: to navigate the turbulent bond market. Understanding the interest rate sensitivity of their portfolios, as measured by these durations, allowed them to make informed decisions about hedging strategies and asset allocation. For example, investors holding longer-duration bonds anticipated larger price fluctuations and adjusted their holdings accordingly. This proactive risk management proved crucial for mitigating losses in a period of rapidly rising interest rates. Macaulay and modified durations are: invaluable tools for portfolio managers.
Consider a pension fund manager responsible for a large bond portfolio. To ensure the fund can meet its future liabilities, they meticulously track the duration of their holdings. By using Macaulay and modified durations, they can estimate the impact of interest rate changes on the fund’s overall value. This information informs their decision-making around bond purchases and sales, helping them to manage the fund’s exposure to interest rate risk and potentially optimize returns. This illustrates how Macaulay and modified durations are: essential for long-term financial planning and liability management.
Another example involves an investor focusing on a specific sector, such as high-yield corporate bonds. These bonds often exhibit higher durations than government bonds, making them more sensitive to interest rate movements. By analyzing Macaulay and modified durations are: for individual bonds and the portfolio as a whole, the investor can quantify the potential risk and return trade-off. This allows for a targeted approach to portfolio construction that balances risk tolerance and return objectives. Careful use of these duration measures enables the investor to make better informed decisions, and potentially improve their overall investment outcomes. Macaulay and modified durations are: critical to assessing the risk associated with these higher-yielding but potentially more volatile investments.