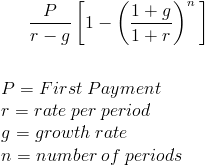What is a Growing Annuity and Why is its Present Value Important?
A growing annuity represents a stream of payments that increase at a constant rate over a specified period. Unlike a regular annuity where payments remain the same, a growing annuity reflects real-world scenarios where payments are adjusted upwards, for example, to account for inflation or salary increases. Consider a rental agreement with annual rent increases, or a retirement plan where pension payments grow each year. Understanding the present value (PV) of a growing annuity is crucial because it allows individuals and businesses to determine the current worth of this future stream of payments. This is essential for making sound financial decisions, such as evaluating the viability of investments or planning for future expenses. Accurately calculating the pv of a growing annuity formula is paramount for informed financial planning, whether it’s assessing the value of a property lease with escalating rent or determining the true cost of a long-term financial obligation. The pv of a growing annuity formula provides a powerful tool for evaluating the current value of future cash flows that are expected to increase over time.
Many financial situations involve cash flows that grow over time, making the concept of a growing annuity extremely relevant. For instance, a bond that pays increasing coupons, or a business that projects increasing profits, can be analyzed using the pv of a growing annuity formula. By discounting these future, growing payments back to their present value, one can arrive at a more accurate assessment of their current worth. Ignoring the growth element in such scenarios could lead to significantly inaccurate valuations, potentially resulting in poor investment choices or flawed financial projections. The ability to accurately calculate the present value of these growing streams using the pv of a growing annuity formula is therefore a critical skill for anyone involved in financial decision-making.
The pv of a growing annuity formula is particularly useful when dealing with long-term financial planning. For example, estimating the present value of future pension payments that are expected to increase annually requires this specific calculation. Similarly, valuing a business with projected growing earnings streams necessitates using the pv of a growing annuity formula, allowing for a more accurate representation of the company’s intrinsic worth compared to methods that ignore the growth component. Mastering the pv of a growing annuity formula provides a significant advantage in accurately assessing the true value of assets and liabilities involving future growing cash flows. It enhances financial literacy and strengthens one’s capacity for informed financial decisions.
Understanding the Formula for Present Value of a Growing Annuity
The mathematical formula used to calculate the present value of a growing annuity, often referred to as the pv of a growing annuity formula, might seem complex at first glance, but it’s built upon a few fundamental concepts. The formula is designed to discount future payments back to their current value, taking into account both the time value of money and the growth of the payments. Specifically, the present value (PV) of a growing annuity is calculated using the following formula: PV = P * [1 – ((1 + g) / (1 + r))^n] / (r – g), where P represents the initial payment, r represents the discount rate, g represents the growth rate of the payments, and n represents the number of periods. The initial payment, P, is the value of the first payment in the annuity stream. The discount rate, r, also known as the interest rate, is used to reflect the opportunity cost of money. The growth rate, g, is the rate at which the payments increase each period. It’s crucial to understand that the discount rate needs to be greater than the growth rate for this formula to be applicable. The number of periods, n, indicates how many payments are considered in the annuity. Each variable plays a critical role in determining the present value, and a change in any of these variables can significantly impact the final result.
Diving deeper into the formula for present value of a growing annuity, consider how each component affects the calculated value. The initial payment (P) acts as the base for all subsequent payments. Higher initial payments will naturally result in a higher present value. The discount rate (r) is inversely related to the present value; that means, a higher discount rate reduces the pv of a growing annuity because it implies a higher opportunity cost for receiving payments in the future. Conversely, a higher growth rate (g) will increase the present value, as it means that future payments are larger and thus more valuable today. It’s vital to note that the relationship between r and g is critical: this formula only works when the discount rate is higher than the growth rate (r > g). If the growth rate is equal to or exceeds the discount rate, the standard formula is invalid and the resulting present value becomes infinite, meaning another different method would be necessary. The number of periods (n) determines for how long we are considering the cashflows, and the longer the period the bigger the impact of the discount and growth rate and the more significant the potential influence on the present value, all of these combined determine the ultimate present value using the pv of a growing annuity formula. The careful consideration of each component makes this calculation very precise and valuable for many financial applications. Visualizing these variables as bullet points can also enhance clarity:
- P = Initial Payment
- r = Discount Rate
- g = Growth Rate
- n = Number of Periods
How to Determine the Present Value of a Growing Annuity: A Step-by-Step Guide
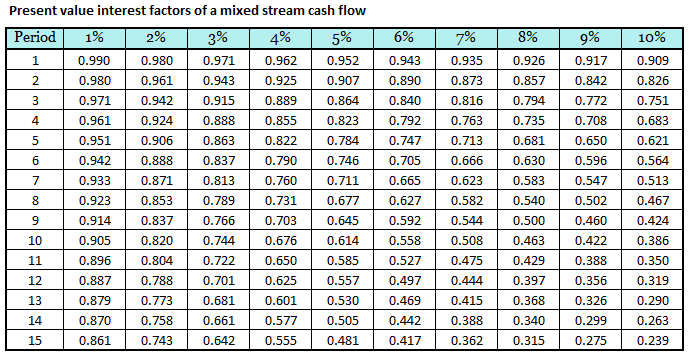
Calculating the present value (PV) of a growing annuity involves using a specific formula that accounts for both the discount rate and the growth rate of payments. The formula is: PV = P * [1 – ((1 + g) / (1 + r))^n] / (r – g), where PV represents the present value, P is the initial payment, r is the discount rate, g is the growth rate, and n is the number of periods. To calculate the pv of a growing annuity formula, you must first identify all the necessary values, which include the payment amount at the start of the annuity, the discount rate per period, the rate of growth in payments per period, and the number of periods over which the payments are made. Ensuring you have these variables accurately is crucial for an effective calculation of the pv of a growing annuity formula. Consider an example, if an initial payment of $1,000 grows at a rate of 3% per year, the discount rate is 5% per year, and the annuity lasts for 10 years. The calculation would be: PV = $1,000 * [1 – ((1 + 0.03) / (1 + 0.05))^10] / (0.05 – 0.03).
Following the formula, the calculation involves several steps. First, calculate the ratio of (1 + g) / (1 + r), which is the growth factor adjusted for the discount rate, then raise that result to the power of n which is the number of periods. The next step subtracts the resulting value from 1. Then, multiply the result by the initial payment, P, and divide the entire expression by (r – g), the difference between the discount rate and the growth rate. In our example, the value of the growth factor adjusted for the discount rate is (1.03/1.05) or 0.98095. Then, raising to the power of 10, the number of periods, is equal to 0.8255. Subtracting from 1 and multiplying by 1000 is equal to 174.5. The denominator of the formula is equal to 0.02. The final PV of a growing annuity formula will be 174.5/0.02 = $8,725. When calculating, it’s crucial to avoid common pitfalls, such as using mismatched time periods for the discount rate, growth rate, and number of periods. If you have a yearly discount rate, you need a yearly growth rate and periods expressed in years. Double-checking your inputs will help avoid these errors.
Impact of Interest and Growth Rates on Present Value
The present value of a growing annuity is significantly influenced by both the discount rate (interest rate) and the growth rate of payments. Understanding this impact is crucial for accurate financial analysis. The discount rate reflects the opportunity cost of capital or the required rate of return. A higher discount rate implies that future cash flows are worth less today, thus lowering the present value of the growing annuity. This is because a higher discount rate suggests a greater preference for receiving money sooner rather than later. Conversely, a lower discount rate increases the present value, as future payments are discounted less heavily. When evaluating investment opportunities, it’s essential to carefully consider the appropriate discount rate. For instance, if a project has a high perceived risk, a higher discount rate is used, leading to a lower present value and potentially influencing the decision to proceed with the investment. This concept directly relates to understanding the pv of a growing annuity formula and its application in real-world scenarios.
The growth rate, which represents the rate at which the payments increase over time, has the opposite effect compared to the discount rate on the present value of a growing annuity. A higher growth rate will increase the present value, as each payment in the future is larger than the previous one, hence making them worth more in present terms. This increase is also subjected to the pv of a growing annuity formula, which takes into account the combined effect of both the discount rate and growth rate. It is worth noting that the discount rate must be higher than the growth rate for the formula to function correctly. Understanding this relationship is key when assessing investments that offer increasing payouts over time, such as dividend paying stocks or rental properties with scheduled rent increases. For example, if a business forecasts a growing income stream, a high growth rate will result in a higher present value. This will make the investment look more attractive, and it also showcases the importance of using the pv of a growing annuity formula in financial planning.
In practical terms, consider scenarios where you are deciding between investment options that have different payout structures. An investment with a lower discount rate and a higher growth rate will generally offer a more attractive present value than an investment with a higher discount rate and a lower growth rate. By understanding how these rates affect present value, one can make more informed decisions in many areas, from project assessment and business valuation to lease analysis and retirement planning. Therefore, a complete understanding of how changes in both interest rates and growth rates affect the present value is essential when working with the pv of a growing annuity formula.
Present Value of a Growing Annuity vs. Other Valuation Methods
Understanding how the present value of a growing annuity contrasts with other valuation methods is crucial for effective financial analysis. While the calculation for the present value of a growing annuity, especially when using the pv of a growing annuity formula, accounts for payments that increase over time, other methods such as the present value of a regular annuity, and the present value of a perpetuity, have different applications and assumptions. The regular annuity method calculates the present value of a series of equal payments over a specified period, which is suitable for situations where payments remain constant, such as a standard loan repayment or a fixed rental agreement. The present value of a perpetuity, on the other hand, calculates the present value of an infinite stream of equal payments, useful when valuing an asset that is expected to yield consistent returns indefinitely, like an endowment. These methods differ significantly from the growing annuity calculation, which specifically accounts for payments that are not constant but grow at a consistent rate. The pv of a growing annuity formula becomes indispensable when dealing with financial instruments or arrangements that feature such growth elements, such as lease agreements with escalations clauses, or retirement payouts that increase over time to cope with inflation.
Choosing the correct present value method depends entirely on the nature of the cash flows being valued. For example, a regular annuity calculation is appropriate for the valuation of a bond that pays a consistent coupon rate. Conversely, the present value of a perpetuity is useful for assets with stable returns over an indefinite future, like a well-established business. However, neither of these methods adequately captures the present value of cash flows when payments are increasing at a specific rate. This is where the pv of a growing annuity formula provides the necessary specificity. The growing annuity formula allows for a more accurate present value calculation when the payment schedule involves incremental increases, a situation that is frequently observed in real estate leases, compensation packages, and certain types of investments. The growing annuity calculation is uniquely designed to handle the complexity of increasing cash flows, which is ignored when using the standard present value of annuity or perpetuity formulas. The distinction lies not in one method being inherently superior but in the appropriateness of each method to the specific characteristics of the cash flow pattern being analysed, making the understanding of when to use the pv of a growing annuity formula an important part of good financial analysis.
In essence, the growing annuity provides a more realistic model for many real-world scenarios, especially when compared to the constant payment approach of the regular annuity and the infinite stream of perpetuity. The regular annuity assumes equal payments, making it inappropriate for situations where the payment amounts increase over time. The perpetuity, with its assumption of infinite payments, is also unsuitable for finite periods involving growth in cash flows. Therefore, if you are trying to find the discounted value of payments that increase over time, such as an investment that provides an increasing income every year, using the pv of a growing annuity formula is the most accurate way to evaluate the actual worth today of future cash flows. The method for a growing annuity serves as a much more nuanced instrument for financial professionals when compared with methods for simple annuities and perpetuities.
Practical Applications of Growing Annuity Present Value Calculation
The pv of a growing annuity formula is not just a theoretical construct; it has widespread practical applications in various financial scenarios. Consider, for example, lease agreements where payments might increase annually. Instead of calculating the present value using a simple annuity, the growing annuity calculation provides a more accurate assessment of the current value of these future lease obligations. This is particularly relevant for businesses assessing the financial viability of long-term leases. In retirement planning, understanding the present value of a growing annuity becomes crucial for individuals expecting periodic payout increases. For instance, if a retiree’s pension payments are projected to increase annually with inflation, the pv of a growing annuity formula can help to accurately estimate the present value of these projected future income streams. This allows for better budgeting and investment planning during retirement years. Similarly, project valuations often rely on cash flow forecasts that incorporate some degree of growth. The pv of a growing annuity formula can be effectively used to determine the present value of these expanding cash flows, making the approach suitable to gauge the potential profitability of a project.
Another important application arises in situations where periodic payments are designed to keep pace with inflation. For example, some insurance policies or structured settlements may provide payments that increase annually. The pv of a growing annuity formula is instrumental in calculating the present value of these payments and understanding the true current value of such an arrangement, providing a more accurate and reliable valuation than assuming constant payments. Understanding the pv of a growing annuity formula also allows investors to compare different investment options by evaluating the present value of their projected payment streams which might be subject to a growth pattern. Consider the valuation of a business with a history of growing dividends; this formula can be used to estimate the present value of the future dividend payments, offering insight into the business’s valuation. Each of these instances highlights how the pv of a growing annuity formula provides a crucial tool for analyzing financial obligations, planning investments, and making well-informed financial decisions.
Tips for Accurately Applying the Present Value Formula
Accurately applying the present value of a growing annuity formula is crucial for obtaining reliable financial insights. One of the most common pitfalls is inconsistency in time periods. It’s imperative to ensure that the discount rate and the growth rate are aligned with the payment frequency. If payments are made annually, both rates should be annual; if payments are monthly, they both should be monthly. Failure to align these periods can lead to a significant miscalculation of the pv of a growing annuity formula. Always double-check that the period used for the number of periods ‘n’ matches the frequency of the growth and discount rates to avoid calculation errors. Another essential step is to meticulously verify the accuracy of all data inputs. Ensure the correct payment amount, discount rate, growth rate, and number of periods are used. Even small errors in input data can have a cascading effect and lead to an incorrect present value. Before performing the calculation, it’s always advisable to review the entered information at least twice for accuracy. A slight mistake can drastically alter the result, especially when dealing with complex financial calculations, and these errors can influence significant financial decisions. Lastly, while manual calculations provide an in-depth understanding of the mechanics, it’s highly beneficial to utilize online calculators or spreadsheet templates to assist with the process. These tools not only provide fast and efficient calculation but also reduce the chances of manual errors. If using spreadsheet templates, be sure to check the formulas and logic implemented in the template to ensure they are accurate for the specific situation. Furthermore, using these resources enables professionals to focus more on financial analysis and less on the mathematical details, which could help lead to better investment strategies and financial planning.
Best practices to avoid errors when calculating the present value of a growing annuity formula involve a combination of meticulous data entry and verification, an understanding of formula consistency, and use of appropriate financial tools. Start by thoroughly reviewing all input data to ensure it aligns with the specific financial scenario being evaluated. Always use the correct notation for the variables and check each value against its source document to verify its accuracy. This step is fundamental in reducing simple data entry mistakes. It’s also important to check the consistency of the time periods for the rate and the periods. Be sure the rates match the calculation frequency, such as converting an annual rate to monthly, which is a critical part of correctly calculating the pv of a growing annuity formula. Avoid using any approximations for the rate, or number of periods which can cause an error of a couple of percentage points. Financial software and spreadsheets are an indispensable tool but must be reviewed as they may have an improper setup that needs adjusting or may be using an incorrect version of the present value of a growing annuity formula. When using these tools be sure to cross-reference with other calculations from different resources. Following these recommendations provides a comprehensive approach to minimize errors and achieve accurate outcomes.
Utilizing Present Value of a Growing Annuity for Informed Decision Making
Understanding the present value of a growing annuity is crucial for making well-informed financial decisions. The concepts discussed throughout this guide, particularly regarding the application of the pv of a growing annuity formula, enable a more accurate assessment of financial opportunities. The ability to calculate the present value of a growing annuity empowers individuals and businesses to evaluate investment options, understand the real cost of long-term financial obligations, and effectively plan for the future. By grasping how discount rates and growth rates affect the present value, one can navigate complex financial landscapes with confidence. For instance, when comparing two seemingly similar investments, knowledge of the pv of a growing annuity formula allows for a more nuanced analysis of their true worth today, accounting for the fact that payments may be increasing over time. The formula also helps to determine if a lease with escalating payments is actually a financially sound option when compared to other alternatives.
The present value calculation provides a framework for understanding the impact of time and growth on money. The nuances of the pv of a growing annuity formula are particularly relevant in scenarios where cash flows are not constant, making it an invaluable tool. When considering retirement planning, for example, understanding how inflation may affect retirement payouts is essential. Utilizing the present value concept, specifically the pv of a growing annuity formula, enables more robust planning for future financial needs. Similarly, in project valuations, this methodology provides a more realistic view of financial returns by taking into account the potential for growth in revenues or savings. The core idea is that money today is worth more than the same amount in the future, and this difference is exacerbated when there is anticipated growth, making the pv of a growing annuity formula vital for financial analysis.
By applying the concepts and techniques of the pv of a growing annuity formula, individuals can make better decisions about leases, retirement plans, project valuations, and many other situations involving periodic payments that increase. The knowledge acquired from understanding and using this formula contributes to a deeper comprehension of financial analysis and improved financial planning. This allows for a more strategic approach to managing resources and making informed decisions about investments and other financial opportunities. The concepts discussed in the previous sections, and particularly the detailed explanation of the pv of a growing annuity formula, serve to reinforce the importance of incorporating this knowledge into daily financial practice.