What Is the Square Root of 252

Understanding the Square Root: A Foundation

The concept of a square root is fundamental in mathematics, serving as the inverse operation of squaring a number. Squaring a number involves multiplying it by itself; for instance, 3 squared (written as 3²) equals 3 * 3, which is 9. The square root, denoted by the symbol √, seeks to find the original number that, when multiplied by itself, gives the number under the root. In essence, the square root of 9 (written as √9) is 3, because 3 * 3 equals 9. Understanding this basic relationship is crucial before exploring more complex numbers. Knowing what is the square root of 252 requires this initial understanding of square roots. This operation is not just an abstract concept; it is widely used in various real-world applications, from calculating areas of squares to advanced physics problems.

To build this understanding further, consider another simple example: the square root of 16 is 4, since 4 * 4 = 16. These basic cases illustrate that the square root of a number is the value that, when multiplied by itself, results in the original number. This understanding will be the base for calculating what is the square root of 252, which is not as straightforward as these initial examples. Knowing how to deal with square roots allows us to measure distances, calculate areas and perform complex engineering calculations. It is a concept that is central to understanding more advanced math. In practical terms, being able to work with and understand square roots will facilitate working through more complex mathematical problems and also to grasp and manipulate more advanced physics and engineering concepts where these values are critical components.

How to Calculate the Root of 252

When considering what is the square root of 252, it’s important to first understand that 252 is not a perfect square. This means that the square root of 252 will not be a whole number. Instead, the result will be a number with a decimal component, requiring us to use methods of approximation or tools like calculators for precision. One helpful approach to simplifying the problem involves identifying perfect square factors within 252. Remember the basics, like 2 multiplied by itself equals 4, or 3 times 3 equals 9. These are examples of perfect squares, and finding such numbers that divide 252 will aid in finding what is the square root of 252.

To understand what is the square root of 252, we need to go a bit deeper. We can start factoring 252 to break it down into numbers that multiply together to equal 252. This process can simplify the root. Although 252 is not a perfect square, it contains factors that are perfect squares. This means there are numbers that when multiplied by themselves, result in a number that can be divided into 252. The goal is to find these square factors, simplifying the calculation of what is the square root of 252. The process of factoring 252 is beneficial because it helps you to rewrite the problem in a way that is more manageable.

Breaking Down 252 Into Its Prime Components

To understand what is the square root of 252, it’s essential to delve into the realm of prime factorization. Prime numbers are whole numbers greater than 1 that are only divisible by 1 and themselves. Examples include 2, 3, 5, 7, and so on. The process of prime factorization involves breaking down a given number into a product of its prime factors. This decomposition will aid in simplifying the square root of 252. To begin, consider dividing 252 by the smallest prime number, which is 2. 252 divided by 2 equals 126. Since 126 is also divisible by 2, we have 126 divided by 2 equaling 63. Moving on, 63 is no longer divisible by 2. The next smallest prime number, 3, can divide 63, giving 21. Once again 21 is divisible by 3, leaving us with 7. Seven is a prime number. Therefore, the prime factorization of 252 is 2 x 2 x 3 x 3 x 7. This step of factorization is crucial to finding what is the square root of 252.

With the prime factorization of 252 now at hand (2 x 2 x 3 x 3 x 7), we can now start to unravel the question of what is the square root of 252. The prime components allow us to look at the number in a different way, highlighting perfect square factors nested within. By breaking down 252 into 2 x 2 x 3 x 3 x 7, we unveil the pairs of 2’s and 3’s, which are essential for simplification in square roots. The presence of these pairs directly impacts how the square root can be simplified. Using prime factors is an extremely useful approach when finding the square root of any number, especially when the number is not a perfect square. This method not only provides a systematic way to approach the problem but also deepens understanding of the components that make up the original number. Therefore, recognizing the prime factors for a number like 252 is an important step towards understanding what is the square root of 252.

Simplifying the Root Using Perfect Square Factors

The prime factorization of 252, as established, is 2 x 2 x 3 x 3 x 7. When determining what is the square root of 252, this factorization becomes crucial. We can rewrite the square root of 252, or √252, as √(2 x 2 x 3 x 3 x 7). The beauty of this approach lies in recognizing perfect square factors. Notice that we have a pair of 2s (2 x 2) and a pair of 3s (3 x 3). These pairs can be pulled out from under the square root symbol as single instances of each number. Remember that the square root of 2 x 2 or 4, is 2 and the square root of 3 x 3 or 9, is 3. Thus, √(2 x 2 x 3 x 3 x 7) simplifies to 2 x 3 x √7. We have essentially taken the square root of the perfect square factors.

Multiplying 2 and 3, we arrive at 6√7. This expression, 6 multiplied by the square root of 7, is an exact simplified form of what is the square root of 252. This simplified form is extremely valuable, as it provides a more manageable representation of the square root. The remaining √7 signifies that the result is not a rational number but an irrational number. While we cannot simplify √7 further into a whole number, it does represent a precise value, and the simplified expression 6√7 maintains the accuracy of the square root of 252. This approach of using prime factorization is not only essential for understanding the root, but for efficient calculation when dealing with large numbers. This way, we have found a way to get the answer of what is the square root of 252 using mathematical principles.

Understanding the Approximate Result

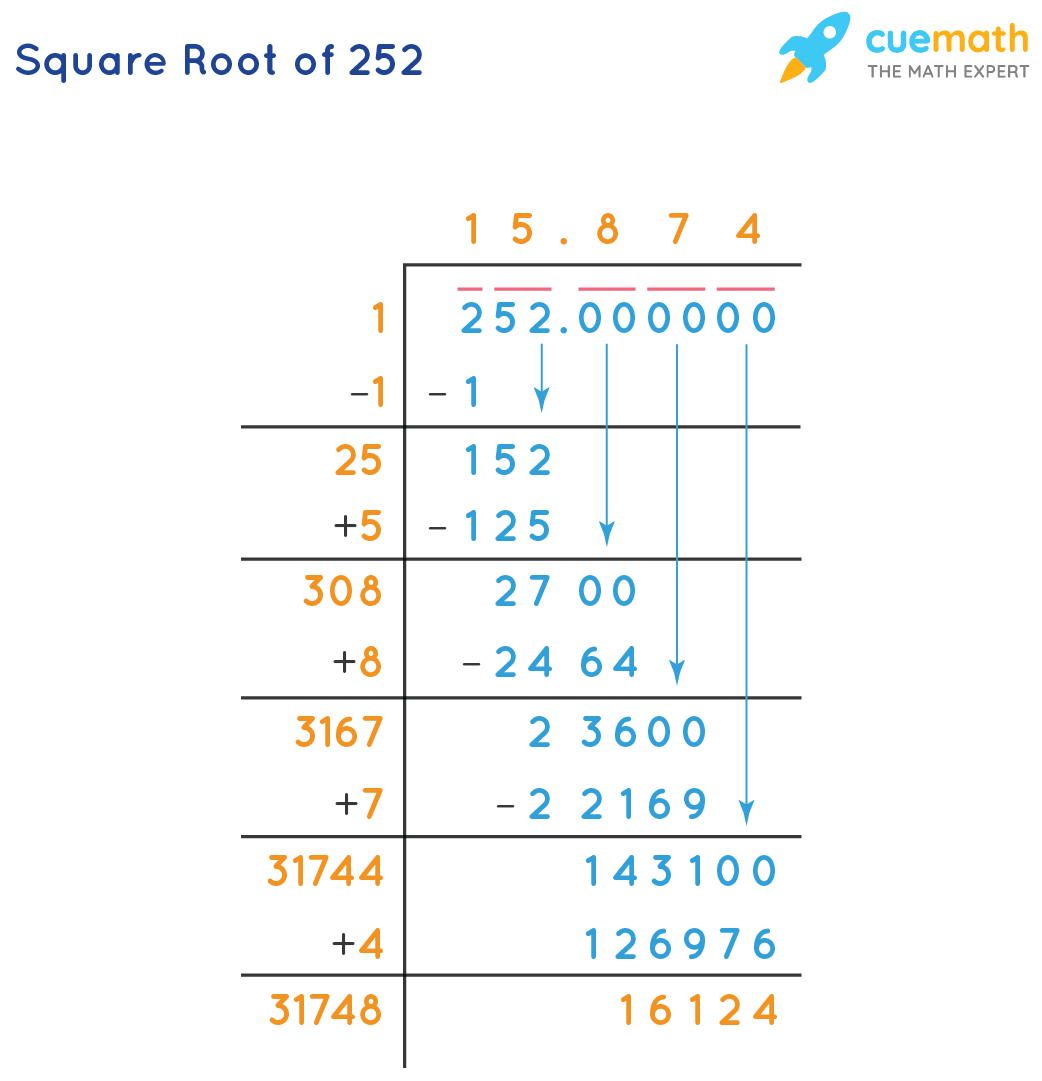
The discussion now moves to the decimal representation of what is the square root of 252. Because 7 is not a perfect square, its square root yields an irrational number, meaning its decimal representation has non-repeating, non-terminating digits. The square root of 7 is approximately 2.646. Consequently, the simplified form, 6 multiplied by the square root of 7, is approximately 6 * 2.646, which results in approximately 15.876. It’s crucial to understand that this is an approximation, not an exact value, as an exact decimal representation is impossible for irrational numbers. Thus, when asked, what is the square root of 252, an approximate answer of 15.87 is often used. Note that the approximation can be refined by using more decimal places of the square root of 7, leading to a more accurate number but the result will still be an approximation of the square root of 252.

While the exact square root of 252 cannot be expressed as a terminating decimal, for practical purposes, approximations like 15.87 are extremely valuable. Approximations allow us to work with numbers that arise from complex calculations more easily. Specifically, in real-world scenarios, understanding an approximate representation of what is the square root of 252 is often more useful than working with the simplified expression that involves root. It allows easier use in calculations, and approximations are accurate enough for many applications. In most cases, this approximation is sufficiently precise for many applications. For example, when measuring a piece of material, a decimal approximation is more useful than the term sqrt(7), and the approximation of what is the square root of 252 can also have real world benefits.

The accuracy of the approximation can be adjusted based on the precision needed for the specific task or use. For many practical problems, the approximation of 15.87 offers an acceptable level of accuracy of what is the square root of 252. While exact representations are essential in theoretical mathematics, in applied fields, approximations bridge the gap between theory and practical application. Furthermore, using approximations allows for easier calculations and provides a better intuition about the magnitude of the number, which is particularly useful in contexts where a quick numerical grasp is necessary. Understanding the process behind finding approximate results enables individuals to assess the accuracy and suitability of a given approximation in various practical situations, and in this case of finding what is the square root of 252.

Understanding the approximate value of what is the square root of 252, is more relevant than the simplified root in many real-world situations. For instance, consider a scenario where an engineer is designing a square platform for a stage. If the desired area of the platform is 252 square meters, the engineer needs to determine the length of each side. The simplified form, 6 times the square root of 7 meters, while mathematically accurate, is difficult to use in construction. In this case, an approximate decimal value is crucial for practical application. Measuring tapes and construction materials are usually calibrated in decimal units, meaning the engineer would need to convert 6 times the square root of 7 to the decimal approximation. In this case, the engineer would use the approximate 15.87 meters to plan and build the platform. Similarly, in physics, calculations often involve square roots. When calculating the speed of an object or the area of a surface, using an approximation for what is the square root of 252 can simplify the process and allow for more streamlined calculations.

Another example would be in the field of computer graphics. When rendering a 3D object, its properties and transformations, including scaling, are often calculated using matrix algebra. Some calculations involve finding the square root of a number, and the result needs to be converted to a usable decimal value for computations. Even in seemingly simple geometrical problems, the approximate value is useful. For example, if one needs to find the diagonal of a rectangle with sides derived from the square root of 252, they would need the approximate value to calculate the diagonal with precision. If for example you have a rectangle of sides 10 meters and 15.87 meters, the diagonal is approximately equal to the square root of (10^2+ 15.87^2) which is very straightforward to calculate if you know that approximately what is the square root of 252 is 15.87. Thus, while simplified or exact expressions have mathematical value, the practical use of the square root of 252 often relies on its decimal approximation. This is because real-world measurements and applications often require a concrete, decimal representation rather than an abstract simplified value.

Tools for Calculating Root Values

While understanding the process of finding the square root of 252 is crucial, various tools can assist in calculating these values efficiently. Calculators, both physical and online, are readily available and designed to perform such calculations quickly. These tools often provide a decimal approximation of the square root, which can be particularly useful for practical applications where a precise value is not always necessary. Additionally, many programming languages include built-in functions for calculating square roots. Using these tools allows for quick numerical evaluation, especially when dealing with complex calculations. The square root of 252, like many other non-perfect squares, leads to a decimal approximation. It’s beneficial to use these tools to quickly find the approximation and avoid the manual process which can be time-consuming.

It is important to acknowledge that even when relying on tools, having a grasp of the fundamental concepts remains essential. These tools can compute the square root of 252 with great precision, but understanding how this result is obtained – through factorization and simplification – allows you to check that the results make sense. Knowing how to manually calculate the values reinforces mathematical intuition and prevents blind reliance on technology. By understanding the logic behind simplifying a root through prime factorization we can check results and better understand what is the square root of 252, and how to address similar problems in the future. The use of technology should serve to enhance and expedite, and never replace fundamental knowledge.

These tools for finding what is the square root of 252 are incredibly convenient when dealing with more complex values. While these tools may offer numerical values, understanding the foundational principles remains extremely valuable in checking results and preventing errors. Learning to approximate square roots also allows you to have a more intuitive sense of the answer, which serves as a vital check on any calculator result. When finding what is the square root of 252, you have the option to rely on the calculator, or apply the theory learned and check the calculator result. In short, the understanding of the math behind finding the square root will allow you to better interpret your results.

Final Thoughts on Finding the Number That Multiplies by Itself

The journey to uncover what is the square root of 252 illustrates a fundamental concept in mathematics: understanding how numbers relate to their roots. We began by exploring the notion of a square root as the inverse of squaring a number, building a foundation for more complex calculations. The number 252, not being a perfect square, presented a unique challenge, necessitating a method for approximation. This involved breaking down 252 into its prime components, which included 2 x 2 x 3 x 3 x 7, and then rewriting the expression to extract perfect square factors. This allowed us to simplify the square root of 252 into the form of 6 times the square root of 7. Through this, we learned that the square root of 252 isn’t a whole number but can be represented and understood using a combination of a whole number and the square root of a prime number.

Understanding what is the square root of 252 also introduced the concept of irrational numbers – numbers whose decimal representation goes on forever without repeating. We discovered that the square root of 7 is approximately 2.646, which, when multiplied by 6, gives us an approximate value of 15.876, simplified to approximately 15.87. This is an important point, as it demonstrates that some numbers do not have perfect square roots and highlights the importance of approximation in practical applications. The practical importance of these approximations was emphasized by illustrating different real-world scenarios where it is useful to work with approximate square roots. Finally, we highlighted that tools can be used for calculating square roots, but a solid grasp of the underlying concepts allows for checking the results and avoiding errors. The process of finding the square root of 252 demonstrates the power of breaking down problems, applying core mathematical principles and how it can lead to a solution. The process we have described is a method that can be used even when the initial number is not a perfect square.