Unlocking the Power of Random Processes in Finance
Stochastic calculus, a branch of mathematics that deals with random processes, has emerged as a powerful tool for modeling and analyzing complex financial systems. In finance, stochastic calculus has far-reaching implications for risk management, derivatives pricing, and portfolio optimization. By applying stochastic calculus to financial modeling, researchers and practitioners can develop more accurate models for predicting stock prices, managing risk, and optimizing investment portfolios. The work of renowned mathematicians like Steven Shreve has been instrumental in shaping the field of stochastic calculus for finance, providing a solid foundation for its applications in modern finance. As the financial industry continues to evolve, the significance of stochastic calculus in finance will only continue to grow, enabling financial professionals to make more informed investment decisions and navigate the intricacies of financial markets with greater confidence.
Steven Shreve’s Contributions to Stochastic Calculus in Finance
Steven Shreve, a renowned mathematician, has made significant contributions to the field of stochastic calculus for finance. His research on Brownian motion and stochastic differential equations has been instrumental in shaping the field of stochastic calculus and its applications in finance. Shreve’s work has provided a solid foundation for the development of stochastic models that can accurately capture the complexities of financial markets. In particular, his research on stochastic differential equations has enabled the creation of more sophisticated models for derivatives pricing and risk management. The impact of Shreve’s work is evident in the widespread adoption of stochastic calculus in finance, with many financial institutions and researchers relying on his theories to inform their investment decisions. As a result, Shreve’s contributions have had a lasting impact on the field of finance, enabling the development of more accurate and effective financial models.
How to Apply Stochastic Calculus to Financial Modeling
Applying stochastic calculus to financial modeling involves a series of steps that enable researchers and practitioners to develop accurate and effective models for predicting stock prices, managing risk, and optimizing investment portfolios. The first step in applying stochastic calculus to financial modeling is the selection of stochastic processes, such as Brownian motion or geometric Brownian motion, that can accurately capture the complexities of financial markets. Once a stochastic process has been selected, the next step is to calibrate the model using historical data, ensuring that the model accurately reflects the behavior of the underlying asset. Simulation techniques, such as Monte Carlo simulations, can then be used to generate multiple scenarios, enabling researchers and practitioners to analyze the behavior of the model under different market conditions. By following these steps, researchers and practitioners can develop sophisticated models that can inform investment decisions and advance our understanding of financial markets. The work of renowned mathematicians like Steven Shreve has been instrumental in shaping the field of stochastic calculus for finance, providing a solid foundation for its applications in modern finance.
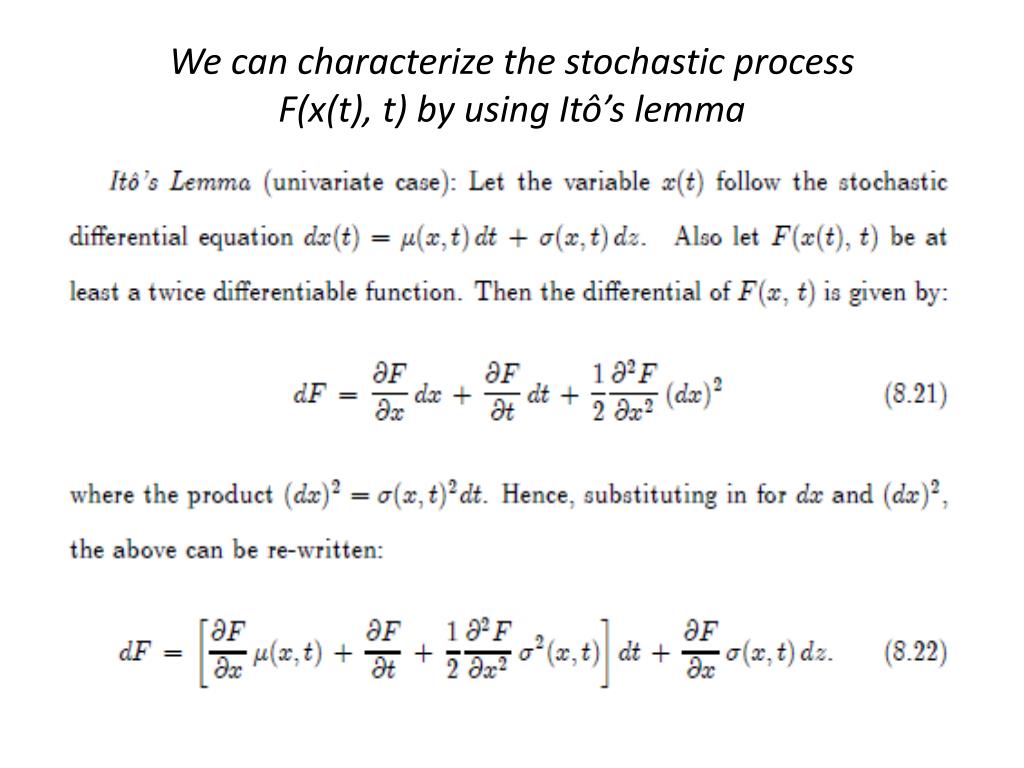
The Role of Ito’s Lemma in Stochastic Calculus for Finance
Ito’s Lemma is a fundamental concept in stochastic calculus, playing a crucial role in the development of stochastic models for finance. In essence, Ito’s Lemma provides a mathematical framework for analyzing the behavior of stochastic processes, enabling researchers and practitioners to accurately price derivatives and manage risk. The lemma’s significance in finance is evident in its application to various areas, including options pricing, credit risk modeling, and portfolio optimization. For instance, Ito’s Lemma can be used to derive the Black-Scholes equation, a fundamental model for pricing European-style options. Furthermore, the lemma’s ability to handle stochastic processes with jumps makes it an essential tool for modeling credit risk and other complex financial phenomena. The work of Steven Shreve, a renowned expert in stochastic calculus for finance, has been instrumental in highlighting the importance of Ito’s Lemma in finance, demonstrating its potential to inform investment decisions and advance our understanding of financial markets.
Stochastic Calculus in Practice: Case Studies in Finance
Stochastic calculus has been successfully applied to a wide range of financial problems, providing valuable insights and solutions to complex issues in finance. One notable example is the use of stochastic calculus in option pricing, where the Black-Scholes model, developed by Fischer Black and Myron Scholes, relies heavily on stochastic calculus to estimate the value of a call option. Another example is credit risk modeling, where stochastic calculus is used to model the probability of default and estimate the expected loss given default. Additionally, stochastic calculus has been applied to portfolio optimization, enabling investors to optimize their portfolios and maximize returns while minimizing risk. The work of Steven Shreve, a renowned expert in stochastic calculus for finance, has been instrumental in developing and applying stochastic calculus to these areas, demonstrating its potential to inform investment decisions and advance our understanding of financial markets. These case studies highlight the power of stochastic calculus in finance, showcasing its ability to provide accurate and reliable results in a wide range of applications.
Overcoming Challenges in Implementing Stochastic Calculus in Finance
While stochastic calculus has revolutionized the field of finance, its implementation is not without challenges. One of the primary obstacles is computational complexity, which can arise from the need to solve complex stochastic differential equations or simulate large datasets. Another challenge is model risk, which occurs when the stochastic model used does not accurately capture the underlying dynamics of the financial system. Data quality issues can also hinder the implementation of stochastic calculus, as high-quality data is essential for calibrating and validating stochastic models. Furthermore, the need for advanced mathematical and computational skills can create a barrier to entry for many finance professionals. Despite these challenges, the work of Steven Shreve and other experts in stochastic calculus for finance has demonstrated the potential of this approach to inform investment decisions and advance our understanding of financial markets. By acknowledging and addressing these challenges, finance professionals can unlock the full potential of stochastic calculus and reap its benefits in risk management, derivatives pricing, and portfolio optimization.
The Future of Stochastic Calculus in Finance: Trends and Opportunities
The field of stochastic calculus for finance, pioneered by experts like Steven Shreve, is poised for significant growth and innovation in the coming years. One of the most promising trends is the integration of machine learning and artificial intelligence with stochastic calculus, enabling the development of more accurate and efficient models for risk management and portfolio optimization. The increasing availability of large datasets and advances in computational power are also expected to drive the adoption of stochastic calculus in finance. Furthermore, the rise of fintech and digital banking is creating new opportunities for the application of stochastic calculus in areas such as credit risk modeling and derivatives pricing. As the field continues to evolve, it is likely that stochastic calculus will play an increasingly important role in informing investment decisions and advancing our understanding of financial markets. With its ability to capture the complexities and uncertainties of financial systems, stochastic calculus is well-positioned to remain a vital tool in the toolkit of finance professionals.
Conclusion: The Importance of Stochastic Calculus in Modern Finance
In conclusion, stochastic calculus has emerged as a powerful tool in modern finance, enabling finance professionals to better understand and manage complex financial systems. The work of Steven Shreve and other experts in stochastic calculus for finance has been instrumental in advancing our understanding of financial markets and informing investment decisions. By applying stochastic calculus to financial modeling, finance professionals can develop more accurate and efficient models for risk management, derivatives pricing, and portfolio optimization. As the field of finance continues to evolve, it is likely that stochastic calculus will play an increasingly important role in shaping our understanding of financial markets and informing investment decisions. With its ability to capture the complexities and uncertainties of financial systems, stochastic calculus is poised to remain a vital tool in the toolkit of finance professionals, enabling them to make more informed investment decisions and drive business growth.