Understanding the Concept of Covariance in Portfolio Management
In the realm of portfolio management, understanding the concept of covariance is crucial for making informed investment decisions. Covariance measures the linear relationship between two assets, providing valuable insights into how they interact with each other. It is a fundamental concept in modern portfolio theory, allowing investors to construct a diversified portfolio that minimizes risk and maximizes returns.
Covariance is a statistical measure that quantifies the extent to which two assets move together. A positive covariance indicates that the two assets tend to move in the same direction, while a negative covariance suggests that they move in opposite directions. A zero covariance implies that the two assets are unrelated. By analyzing the covariance between different assets, investors can identify opportunities to diversify their portfolio and reduce risk.
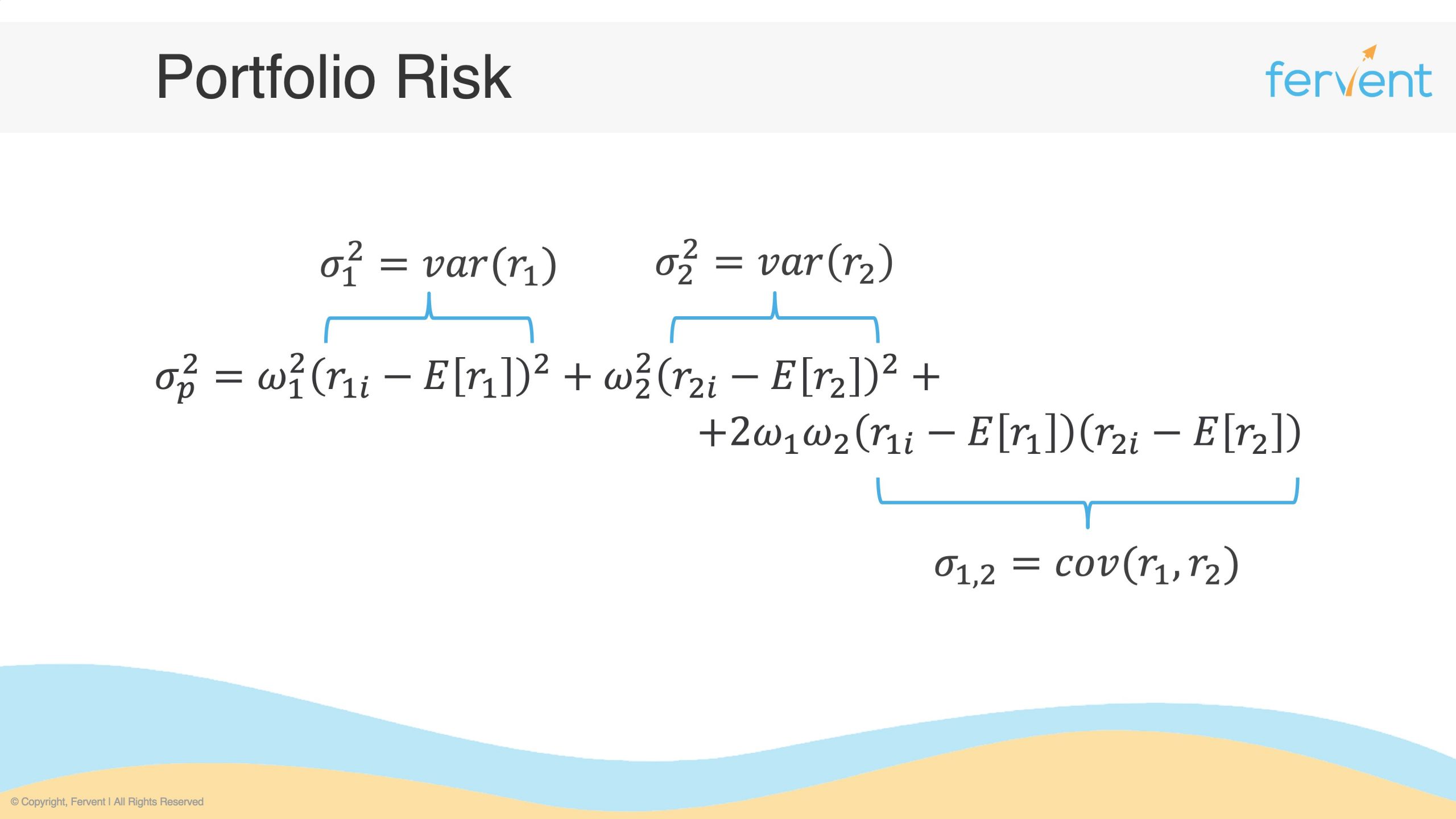
In the context of portfolio management, covariance is essential for calculating the risk of a portfolio. It helps investors to understand how the individual assets within a portfolio interact with each other, and how these interactions affect the overall risk profile of the portfolio. By incorporating covariance into their investment strategy, investors can create a more efficient portfolio that balances risk and return.
The significance of covariance in portfolio management cannot be overstated. It provides a powerful tool for investors to manage risk, optimize returns, and construct a diversified portfolio. As investors navigate the complexities of the financial markets, a deep understanding of covariance is essential for making informed investment decisions and achieving long-term success.
How to Calculate Covariance: A Simple Formula for Portfolio Analysis
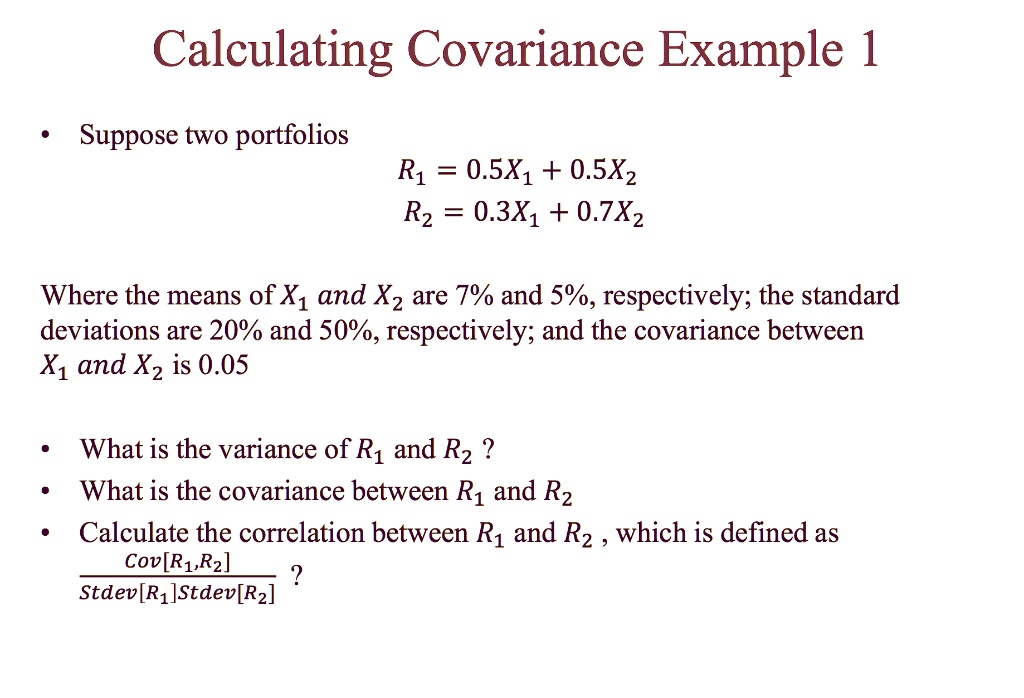
Calculating covariance is a crucial step in portfolio analysis, as it helps investors understand the relationship between different assets in their portfolio. The covariance of a portfolio formula is a powerful tool that enables investors to quantify the linear relationship between two assets, providing valuable insights into how they interact with each other.
The covariance of a portfolio formula is calculated using the following equation:
Cov(X, Y) = Σ[(Xi – X̄)(Yi – Ȳ)] / (n – 1)
The Role of Covariance in Portfolio Diversification
Portfolio diversification is a crucial aspect of investment management, and covariance plays a vital role in achieving this goal. By understanding the covariance of a portfolio formula, investors can construct a diversified portfolio that minimizes risk and maximizes returns. Covariance helps investors to identify the relationships between different assets in their portfolio, enabling them to make informed decisions about asset allocation.
In a diversified portfolio, assets with low or negative covariance are combined to reduce overall risk. This is because these assets tend to move in opposite directions, thereby offsetting each other’s fluctuations. By incorporating assets with low covariance, investors can create a portfolio that is less susceptible to market volatility, thereby reducing the risk of losses.
On the other hand, assets with high covariance tend to move in the same direction, amplifying the overall risk of the portfolio. By avoiding assets with high covariance, investors can reduce the risk of their portfolio and increase the potential for long-term returns.
The importance of covariance in portfolio diversification cannot be overstated. By understanding the relationships between different assets, investors can create a portfolio that is optimized for risk and return. This is particularly important in today’s complex and volatile financial markets, where investors need to be proactive in managing risk and maximizing returns.
In conclusion, covariance is a powerful tool in portfolio diversification, enabling investors to construct a diversified portfolio that minimizes risk and maximizes returns. By understanding the covariance of a portfolio formula, investors can make informed decisions about asset allocation and create a portfolio that is optimized for long-term success.
Interpreting Covariance Results: What Do the Numbers Mean?
Once the covariance of a portfolio formula has been calculated, it’s essential to understand what the results mean. Interpreting covariance results is crucial in portfolio management, as it helps investors make informed decisions about asset allocation and risk management.
A positive covariance value indicates that the two assets tend to move in the same direction. For example, if the covariance between two stocks is 0.8, it means that when one stock increases in value, the other stock is likely to increase as well. Positive covariance values are often associated with high-risk portfolios, as the assets tend to amplify each other’s fluctuations.
On the other hand, a negative covariance value indicates that the two assets tend to move in opposite directions. For instance, if the covariance between two assets is -0.5, it means that when one asset increases in value, the other asset is likely to decrease in value. Negative covariance values are often associated with low-risk portfolios, as the assets tend to offset each other’s fluctuations.
A zero covariance value indicates that the two assets are unrelated, meaning that the movement of one asset does not affect the movement of the other asset. Zero covariance values are often associated with diversified portfolios, as the assets do not tend to amplify or offset each other’s fluctuations.
It’s essential to note that covariance values can range from -1 to 1, with -1 indicating perfect negative correlation, 1 indicating perfect positive correlation, and 0 indicating no correlation. By understanding the covariance results, investors can make informed decisions about asset allocation, risk management, and portfolio optimization.
In addition to understanding the covariance values, investors should also consider the magnitude of the covariance. A high covariance value, whether positive or negative, indicates a strong relationship between the assets, while a low covariance value indicates a weak relationship. By considering both the sign and magnitude of the covariance, investors can gain a deeper understanding of the relationships between their assets and make more informed investment decisions.
Common Mistakes to Avoid When Calculating Covariance
Calculating covariance is a crucial step in portfolio risk management, but it’s not without its pitfalls. Avoiding common mistakes is essential to ensure accurate results and informed investment decisions. Here are some common errors to avoid when calculating covariance:
Incorrect Data Inputs: One of the most critical mistakes is using incorrect or incomplete data inputs. This can lead to inaccurate covariance calculations, which can have a ripple effect on the entire portfolio. Ensure that the data is accurate, complete, and relevant to the assets being analyzed.
Flawed Assumptions: Another common mistake is making flawed assumptions about the relationships between assets. For example, assuming that two assets are perfectly correlated when they’re not can lead to inaccurate covariance calculations. It’s essential to understand the underlying relationships between assets and make informed assumptions.
Misinterpretation of Results: Misinterpreting covariance results is a common mistake that can have significant consequences. For instance, misunderstanding the sign and magnitude of covariance values can lead to incorrect investment decisions. Ensure that you understand what the covariance results mean and how to apply them in practice.
Inconsistent Time Frames: Using inconsistent time frames for data collection can lead to inaccurate covariance calculations. Ensure that the data is collected over the same time frame for all assets being analyzed.
Ignoring Non-Linear Relationships: Covariance only measures linear relationships between assets. Ignoring non-linear relationships can lead to inaccurate covariance calculations. Consider using advanced techniques, such as conditional covariance, to capture non-linear relationships.
Not Accounting for Market Conditions: Failing to account for market conditions, such as volatility and trends, can lead to inaccurate covariance calculations. Ensure that you consider market conditions when calculating covariance and making investment decisions.
By avoiding these common mistakes, investors can ensure accurate covariance calculations and make informed investment decisions. Remember, covariance is a powerful tool in portfolio risk management, but it’s only as good as the data and assumptions that go into it. By being aware of these common mistakes, investors can optimize their portfolios and achieve their investment goals.
Real-World Applications of Covariance in Portfolio Management
Covariance is not just a theoretical concept; it has numerous real-world applications in portfolio management. In this section, we’ll explore how covariance is used in practice, including case studies and industry examples.
Asset Allocation: Covariance is used to optimize asset allocation in portfolios. By analyzing the covariance between different assets, investors can create a diversified portfolio that minimizes risk and maximizes returns. For example, a portfolio manager may use covariance to determine the optimal allocation between stocks and bonds.
Risk Management: Covariance is used to manage risk in portfolios. By analyzing the covariance between different assets, investors can identify potential risks and take steps to mitigate them. For example, a portfolio manager may use covariance to identify assets that are highly correlated and diversify the portfolio to reduce risk.
Portfolio Optimization: Covariance is used to optimize portfolios by identifying the most efficient allocation of assets. By analyzing the covariance between different assets, investors can create a portfolio that maximizes returns while minimizing risk. For example, a portfolio manager may use covariance to determine the optimal allocation between different asset classes, such as stocks, bonds, and commodities.
Case Study: Consider a portfolio manager who wants to create a diversified portfolio of stocks. By analyzing the covariance between different stocks, the manager can identify the most correlated stocks and diversify the portfolio to reduce risk. For example, if the manager finds that two stocks have a high positive covariance, they may decide to reduce the allocation to one of the stocks to minimize risk.
Industry Example: Many investment firms use covariance to manage risk and optimize portfolios. For example, BlackRock, one of the largest investment firms in the world, uses covariance to manage risk in its portfolios. By analyzing the covariance between different assets, BlackRock can create diversified portfolios that minimize risk and maximize returns.
In addition to these examples, covariance is used in a variety of other real-world applications, including hedge fund management, pension fund management, and wealth management. By understanding how covariance is used in practice, investors can gain a deeper appreciation for its importance in portfolio risk management.
By applying the covariance of a portfolio formula in practice, investors can create diversified and optimized portfolios that minimize risk and maximize returns. Whether it’s asset allocation, risk management, or portfolio optimization, covariance is a powerful tool that can help investors achieve their investment goals.
Advanced Covariance Techniques for Sophisticated Investors
While the basic concept of covariance is essential for portfolio risk management, sophisticated investors can benefit from advanced covariance techniques to further optimize their portfolios. In this section, we’ll explore conditional covariance, covariance matrices, and their applications in portfolio management.
Conditional Covariance: Conditional covariance is a technique that measures the covariance between two assets conditional on a specific event or scenario. This technique is useful for investors who want to analyze the behavior of their portfolio under different market conditions. For example, an investor may want to analyze the covariance between two stocks conditional on a market downturn.
Covariance Matrices: A covariance matrix is a table that displays the covariance between multiple assets. This technique is useful for investors who want to analyze the relationships between multiple assets in their portfolio. By analyzing the covariance matrix, investors can identify the most correlated assets and diversify their portfolio to reduce risk.
Applications in Portfolio Management: Advanced covariance techniques have numerous applications in portfolio management. For example, conditional covariance can be used to create scenario-based portfolios that are optimized for different market conditions. Covariance matrices can be used to identify the most correlated assets and diversify the portfolio to reduce risk.
Example: Consider a sophisticated investor who wants to create a portfolio that is optimized for a market downturn. By using conditional covariance, the investor can analyze the covariance between different assets conditional on a market downturn and create a portfolio that is optimized for that scenario.
Industry Example: Many investment firms use advanced covariance techniques to manage risk and optimize portfolios. For example, Goldman Sachs uses covariance matrices to analyze the relationships between different assets and create diversified portfolios for its clients.
By applying advanced covariance techniques, sophisticated investors can gain a deeper understanding of their portfolios and make more informed investment decisions. Whether it’s conditional covariance or covariance matrices, these techniques can help investors create optimized portfolios that minimize risk and maximize returns.
In addition to these advanced techniques, investors can also use the covariance of a portfolio formula to analyze the relationships between different assets and create diversified portfolios. By combining these techniques, investors can create optimized portfolios that are tailored to their specific investment goals and risk tolerance.
Conclusion: The Power of Covariance in Portfolio Risk Management
In conclusion, covariance is a powerful tool in portfolio risk management, enabling investors to create diversified and optimized portfolios that minimize risk and maximize returns. By understanding the concept of covariance, calculating it using the covariance of a portfolio formula, and applying advanced techniques such as conditional covariance and covariance matrices, investors can gain a deeper insight into their portfolios and make more informed investment decisions.
The importance of covariance in portfolio diversification cannot be overstated. By analyzing the covariance between different assets, investors can identify the most correlated assets and diversify their portfolio to reduce risk. This, in turn, can lead to increased returns and a more stable portfolio.
Moreover, covariance is not just a theoretical concept; it has numerous real-world applications in portfolio management. From asset allocation to risk management, covariance is used by investment firms and individual investors alike to create optimized portfolios.
In today’s complex and volatile market environment, understanding covariance is more important than ever. By mastering the concept of covariance and its applications in portfolio management, investors can stay ahead of the curve and achieve their investment goals.
In summary, covariance is a crucial component of portfolio risk management, and its importance cannot be overstated. By applying the concepts and techniques discussed in this guide, investors can create diversified and optimized portfolios that minimize risk and maximize returns.