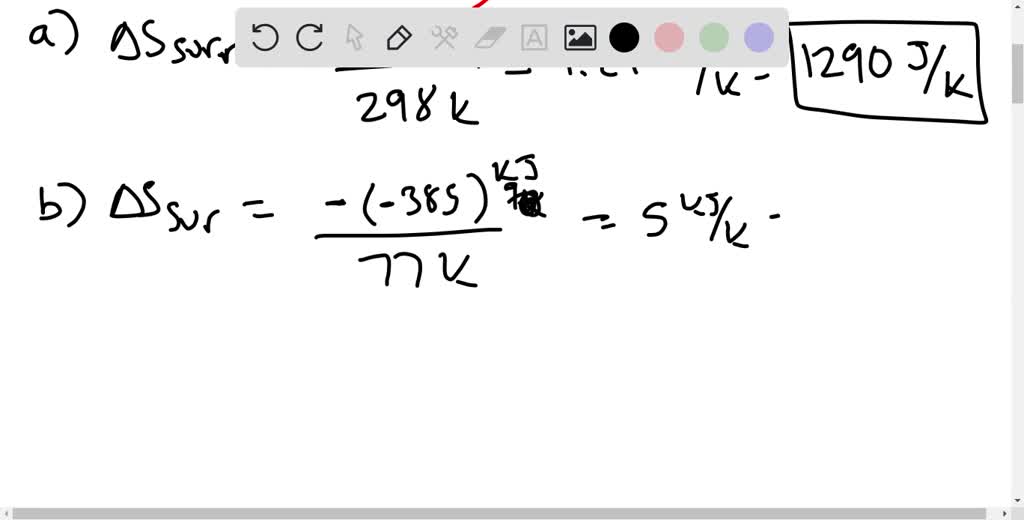Unraveling the Mystery of Delta
In the realms of finance, physics, and engineering, the concept of delta holds significant importance. It is a crucial element in making informed decisions, as it enables professionals to measure change, difference, or variation. But, have you ever wondered how do you calculate delta? Understanding delta calculation is vital in these domains, as it provides valuable insights that facilitate data-driven decision-making. In finance, delta calculation helps investors and analysts assess the sensitivity of an option’s price to changes in the underlying asset’s price. In physics and engineering, delta calculation is used to describe changes in velocity, acceleration, and energy. The significance of delta calculation lies in its ability to provide a deeper understanding of complex systems, enabling professionals to make informed decisions that drive success.
What is Delta Anyway?
Delta, denoted by the symbol Δ (delta), is a fundamental concept in various fields, including finance, physics, and engineering. In essence, delta represents the change, difference, or variation in a quantity or value. It is a crucial element in understanding complex systems and making informed decisions. But, how do you calculate delta? The answer lies in its applications, which span across multiple domains. In finance, delta is used to measure the sensitivity of an option’s price to changes in the underlying asset’s price. In physics and engineering, delta is used to describe changes in velocity, acceleration, and energy. Understanding delta calculation is vital in these domains, as it provides valuable insights that facilitate data-driven decision-making.
The Formula for Calculating Delta
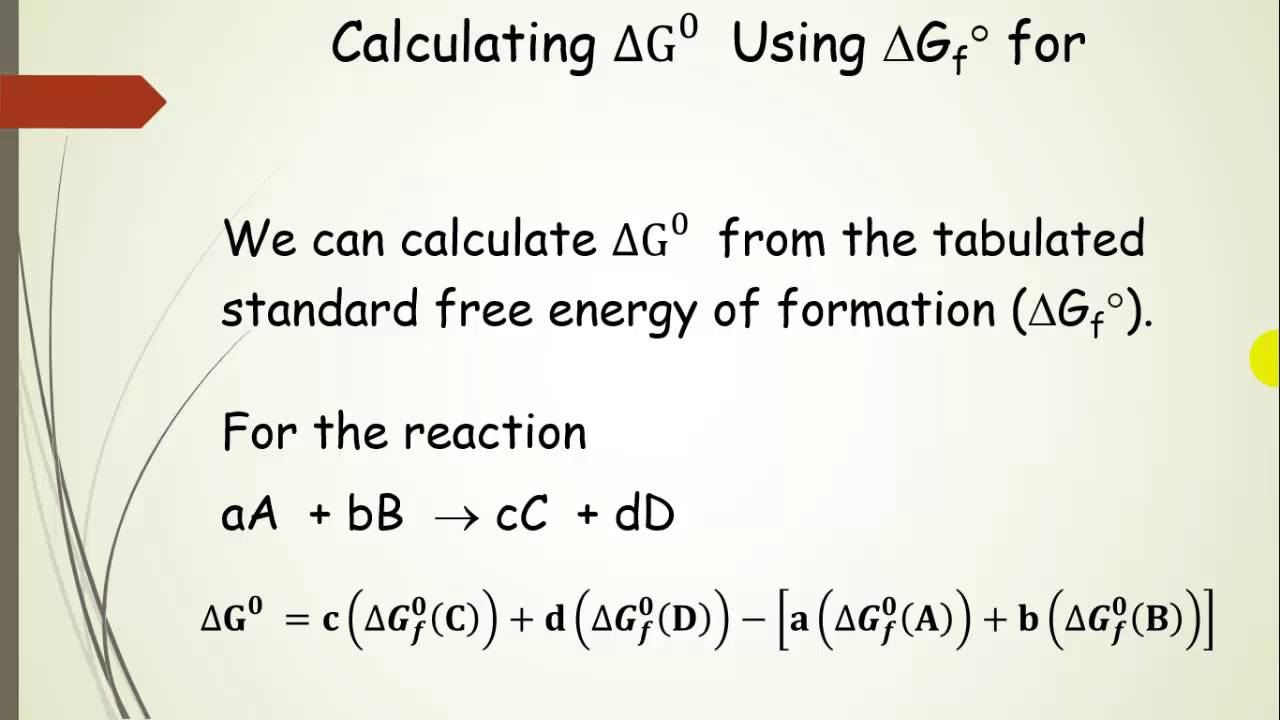
The general formula for calculating delta is Δ = (New Value – Old Value) / Old Value, where Δ represents the change or difference, and New Value and Old Value are the new and old values of the quantity being measured, respectively. This formula is widely applicable across various domains, including finance, physics, and engineering. To illustrate the concept, let’s consider a simple example: if the price of a stock increases from $50 to $60, the delta would be (60 – 50) / 50 = 0.2, indicating a 20% change. Understanding this formula is crucial, as it forms the basis of delta calculation in different contexts. So, how do you calculate delta? By mastering this formula, you can unlock the secrets of delta calculation and make informed decisions in your respective field.
How to Calculate Delta in Finance
In finance, delta calculation plays a crucial role in options trading and risk management. The delta of an option represents the rate of change of the option’s price with respect to the underlying asset’s price. It is a key metric used to measure the sensitivity of an option’s price to changes in the underlying asset’s price. To calculate delta in finance, one can use the Black-Scholes model, which provides a mathematical framework for estimating the delta of an option. The formula for calculating delta in finance is Δ = N(d1), where N(d1) is the cumulative distribution function of the standard normal distribution. By understanding how to calculate delta in finance, investors and traders can make informed decisions about their investments and manage risk more effectively. For instance, if the delta of a call option is 0.5, it means that for every $1 increase in the underlying asset’s price, the option’s price is expected to increase by $0.50. So, how do you calculate delta in finance? By mastering this concept, you can gain a deeper understanding of options trading and risk management.
Delta Calculation in Physics and Engineering
In physics and engineering, delta calculation is a fundamental concept used to describe changes in various physical quantities, such as velocity, acceleration, and energy. In kinematics, delta is used to calculate the change in velocity (Δv) or acceleration (Δa) of an object over a given time period. For instance, if an object’s velocity increases from 10 m/s to 15 m/s in 2 seconds, the delta velocity would be Δv = (15 – 10) / 2 = 2.5 m/s². In dynamics, delta is used to calculate the change in energy (ΔE) of a system, which is essential in understanding the behavior of mechanical systems. In thermodynamics, delta is used to calculate the change in entropy (ΔS) of a system, which is crucial in understanding the efficiency of heat engines and refrigeration systems. By understanding how to calculate delta in physics and engineering, professionals can design and optimize systems more effectively, ensuring improved performance and efficiency. So, how do you calculate delta in physics and engineering? By mastering this concept, you can unlock the secrets of delta calculation and make informed decisions in your respective field.
Common Mistakes to Avoid When Calculating Delta
When calculating delta, it’s essential to avoid common mistakes that can lead to inaccurate results. One of the most common mistakes is incorrect unit conversions. For instance, if you’re calculating the delta of an object’s velocity, you need to ensure that the units of measurement are consistent throughout the calculation. Another mistake is misunderstanding the formula for calculating delta. This can lead to incorrect calculations and inaccurate results. Additionally, neglecting significant digits can also lead to errors in delta calculation. It’s crucial to maintain a high degree of precision when calculating delta to ensure accurate results. So, how do you calculate delta accurately? By being aware of these common mistakes, you can avoid errors and ensure that your delta calculations are accurate and reliable. Furthermore, it’s essential to double-check your calculations and verify your results to ensure that they are correct. By doing so, you can build confidence in your ability to calculate delta and make informed decisions in your respective field.
Real-World Applications of Delta Calculation
Delta calculation has numerous real-world applications across various industries, including finance, physics, engineering, and data analysis. In finance, delta calculation is used to measure the sensitivity of an option’s price to changes in the underlying asset’s price, enabling investors to make informed investment decisions. In physics and engineering, delta calculation is used to describe changes in velocity, acceleration, and energy, which is crucial in designing and optimizing systems. In data analysis, delta calculation is used to identify trends and patterns in data, enabling businesses to make data-driven decisions. For instance, in quality control, delta calculation can be used to measure the change in product quality over time, enabling manufacturers to identify areas for improvement. So, how do you calculate delta in these real-world applications? By understanding the concept of delta calculation and its applications, professionals can make informed decisions and drive success in their respective fields. The importance of accurate delta calculation cannot be overstated, as it can have a significant impact on the bottom line of a business or the success of a project. By mastering delta calculation, professionals can unlock the secrets of change and variation, and make a meaningful impact in their respective fields.
Conclusion: Mastering Delta Calculation for Success
In conclusion, mastering the art of delta calculation is crucial for making informed decisions in various fields, including finance, physics, engineering, and data analysis. By understanding the concept of delta, its applications, and the formula for calculating delta, professionals can unlock the secrets of change and variation. It is essential to avoid common mistakes and pitfalls when calculating delta, such as incorrect unit conversions and misunderstanding the formula. By applying delta calculation in real-world scenarios, professionals can drive success in their respective fields. Remember, how do you calculate delta is a critical question that requires a deep understanding of the concept and its applications. By practicing and applying delta calculation, professionals can make a meaningful impact in their fields and achieve success. With the knowledge and skills acquired from this article, readers can now confidently tackle complex problems and make informed decisions in their respective fields.